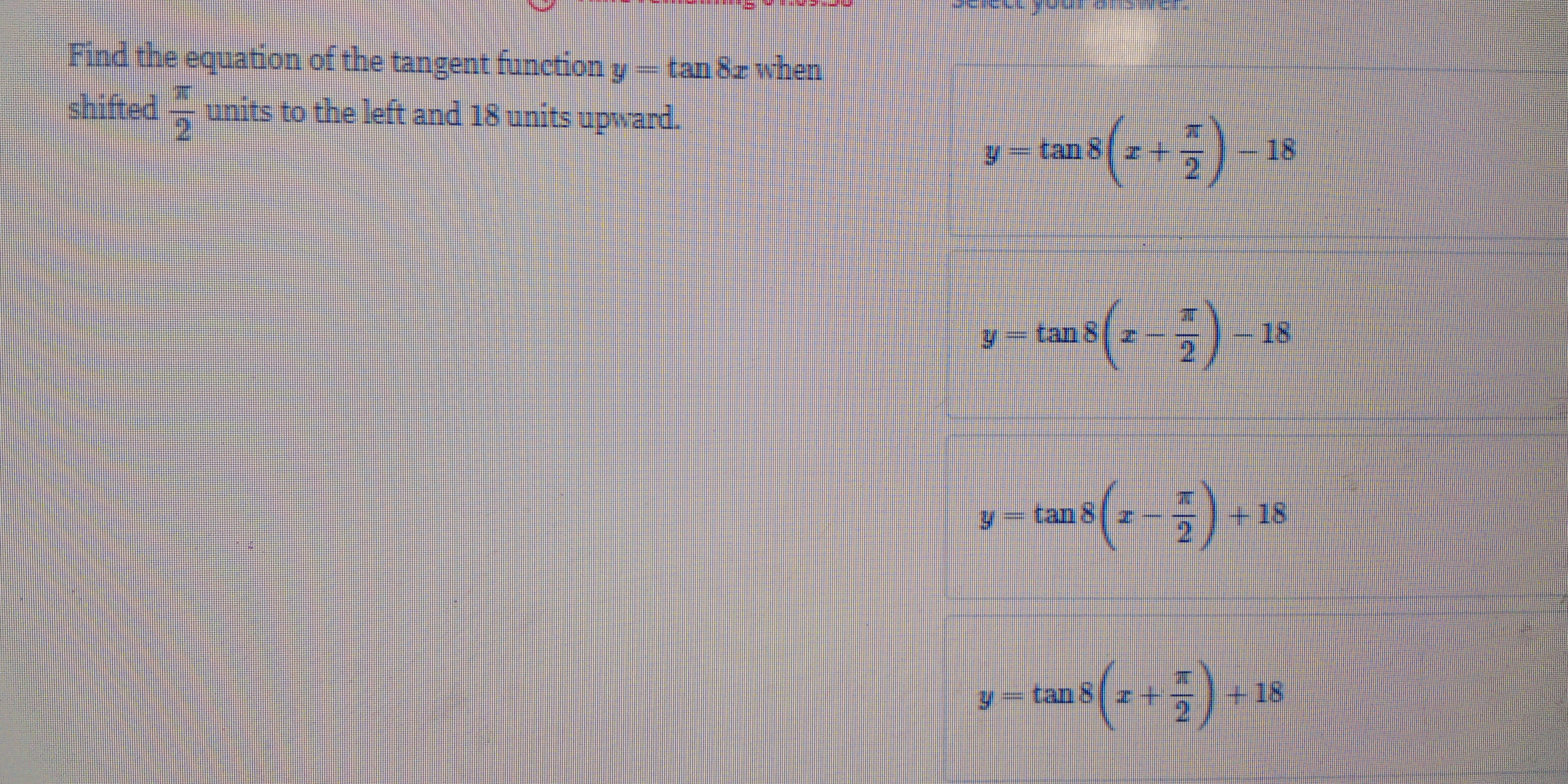
Find the equation of the tangent function y = tan(8x) when shifted -π/2 units to the left and 18 units upward.
Understand the Problem
The question is asking for the equation of the tangent function y = tan(8x) after being shifted -π/2 units to the left and 18 units upward. This requires understanding how horizontal and vertical transformations affect the function.
Answer
$$ y = \tan(8(x + \frac{\pi}{2})) + 18 $$
Answer for screen readers
The final equation after shifting is: $$ y = \tan(8(x + \frac{\pi}{2})) + 18 $$
Steps to Solve
- Start with the original function
The original tangent function given is: $$ y = \tan(8x) $$
- Shift horizontally to the left
To shift the graph to the left by $\frac{\pi}{2}$ units, you replace $x$ with $(x + \frac{\pi}{2})$ in the function. This gives: $$ y = \tan\left(8\left(x + \frac{\pi}{2}\right)\right) $$
- Expand the equation
Next, distribute the 8 inside the function: $$ y = \tan(8x + 4\pi) $$
- Shift vertically upward
To shift the function upward by 18 units, you add 18: $$ y = \tan(8x + 4\pi) + 18 $$
- Final expression
Since the $\tan$ function has a period of $\pi$, adding $4\pi$ does not change its graph. Thus, the function simplifies to: $$ y = \tan(8x) + 18 $$
However, to maintain the leftward shift notation, we write it in the form: $$ y = \tan(8(x + \frac{\pi}{2})) + 18 $$
The final equation after shifting is: $$ y = \tan(8(x + \frac{\pi}{2})) + 18 $$
More Information
The equation of the tangent function can be transformed through both horizontal and vertical shifts. The $\tan$ function has a periodic nature, meaning shifts in its input can often be simplified based on its period.
Tips
- Confusing the direction of shifts. Remember that shifting left involves adding a positive value to $x$.
- Forgetting to adjust the vertical position of the function after horizontal shifts. Always apply the vertical transformations after the horizontal ones.
AI-generated content may contain errors. Please verify critical information