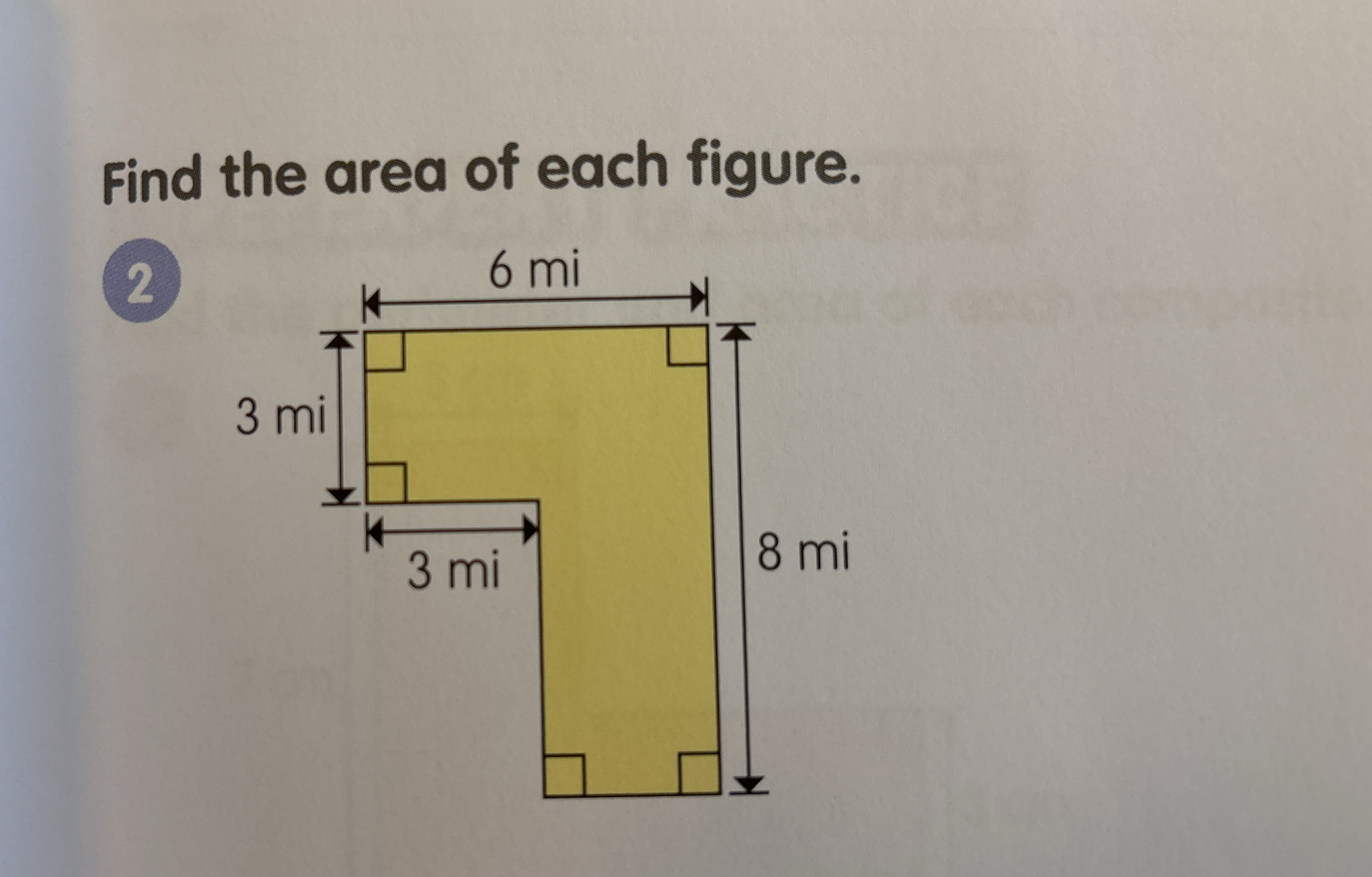
Find the area of each figure.
Understand the Problem
The question is asking to find the area of a given figure, which appears to have a complex shape that can be divided into simpler rectangular parts for calculation.
Answer
The total area of the figure is $39 \, \text{mi}^2$.
Answer for screen readers
The total area of the figure is $39 , \text{mi}^2$.
Steps to Solve
- Identify the Shape Components
The figure can be divided into two rectangles for easier area calculation. The dimensions of the rectangles are as follows:
- Rectangle 1: Width = $6 , \text{mi}$, Height = $8 , \text{mi}$
- Rectangle 2 (the cut-out part): Width = $3 , \text{mi}$, Height = $3 , \text{mi}$
- Calculate the Area of Rectangle 1
To find the area of Rectangle 1, use the formula for the area of a rectangle: $$ \text{Area} = \text{Width} \times \text{Height} $$
For Rectangle 1: $$ \text{Area}_1 = 6 , \text{mi} \times 8 , \text{mi} = 48 , \text{mi}^2 $$
- Calculate the Area of Rectangle 2
Now calculate the area of Rectangle 2 (the part that is subtracted): $$ \text{Area}_2 = 3 , \text{mi} \times 3 , \text{mi} = 9 , \text{mi}^2 $$
- Find the Total Area of the Figure
To find the area of the overall figure, subtract the area of Rectangle 2 from Rectangle 1: $$ \text{Total Area} = \text{Area}_1 - \text{Area}_2 $$ $$ \text{Total Area} = 48 , \text{mi}^2 - 9 , \text{mi}^2 = 39 , \text{mi}^2 $$
The total area of the figure is $39 , \text{mi}^2$.
More Information
The calculation involves breaking down a complex shape into simpler rectangles. This technique can be applied to many geometric figures to simplify area calculations.
Tips
- Forgetting to subtract the area of the cut-out part. To avoid this, always double-check dimensions and ensure all parts of the shape are accounted for.
AI-generated content may contain errors. Please verify critical information