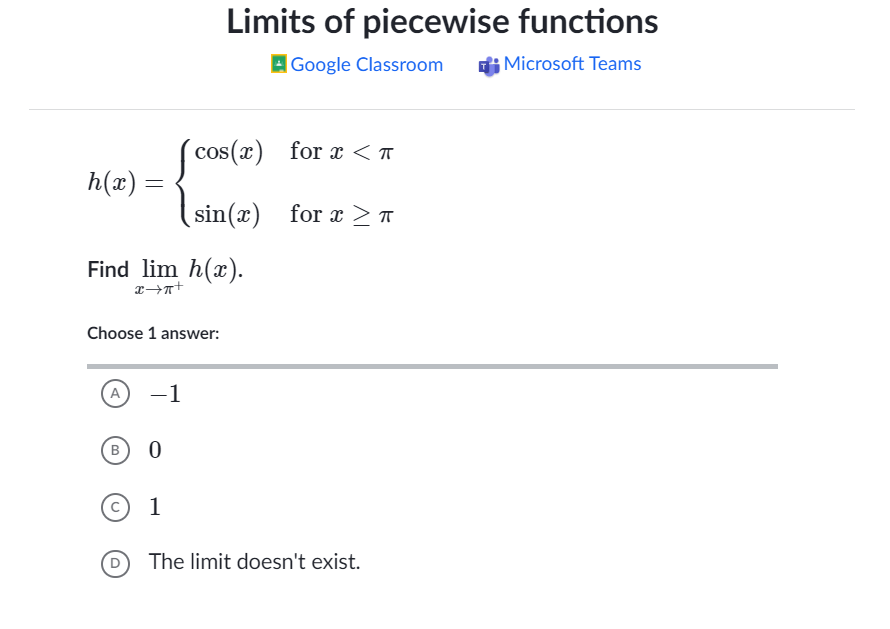
Find lim (x→π⁺) h(x).
Understand the Problem
The question is asking for the limit of a piecewise function as x approaches π from the right. It requires evaluating the function h(x) based on the defined behavior for values of x greater than or equal to π.
Answer
The limit is $0$.
Answer for screen readers
The limit is $0$.
Steps to Solve
- Identify the function for the limit approach
Since we are finding the limit as $x$ approaches $\pi$ from the right (denoted as $x \to \pi^+$), we will use the piece of the function defined for $x \geq \pi$. Thus, we consider $h(x) = \sin(x)$.
- Evaluate the limit
Now we substitute $\pi$ into the function:
$$ \lim_{x \to \pi^+} h(x) = \sin(\pi) $$
- Calculate $\sin(\pi)$
The sine of $\pi$ is:
$$ \sin(\pi) = 0 $$
- State the final result
Thus, we conclude that:
$$ \lim_{x \to \pi^+} h(x) = 0 $$
The limit is $0$.
More Information
The sine function, $\sin(x)$, takes on specific values at various points. For example, $\sin(0) = 0$, $\sin(\pi) = 0$, and $\sin(2\pi) = 0$. Understanding these values is crucial in limit evaluations for piecewise functions.
Tips
- Ignoring the piecewise condition: Ensure you use the correct function for the limit depending on the direction from which you approach the value.
- Confusing sine and cosine: Remember that you have to choose $h(x)$ correctly based on the piecewise function.
AI-generated content may contain errors. Please verify critical information