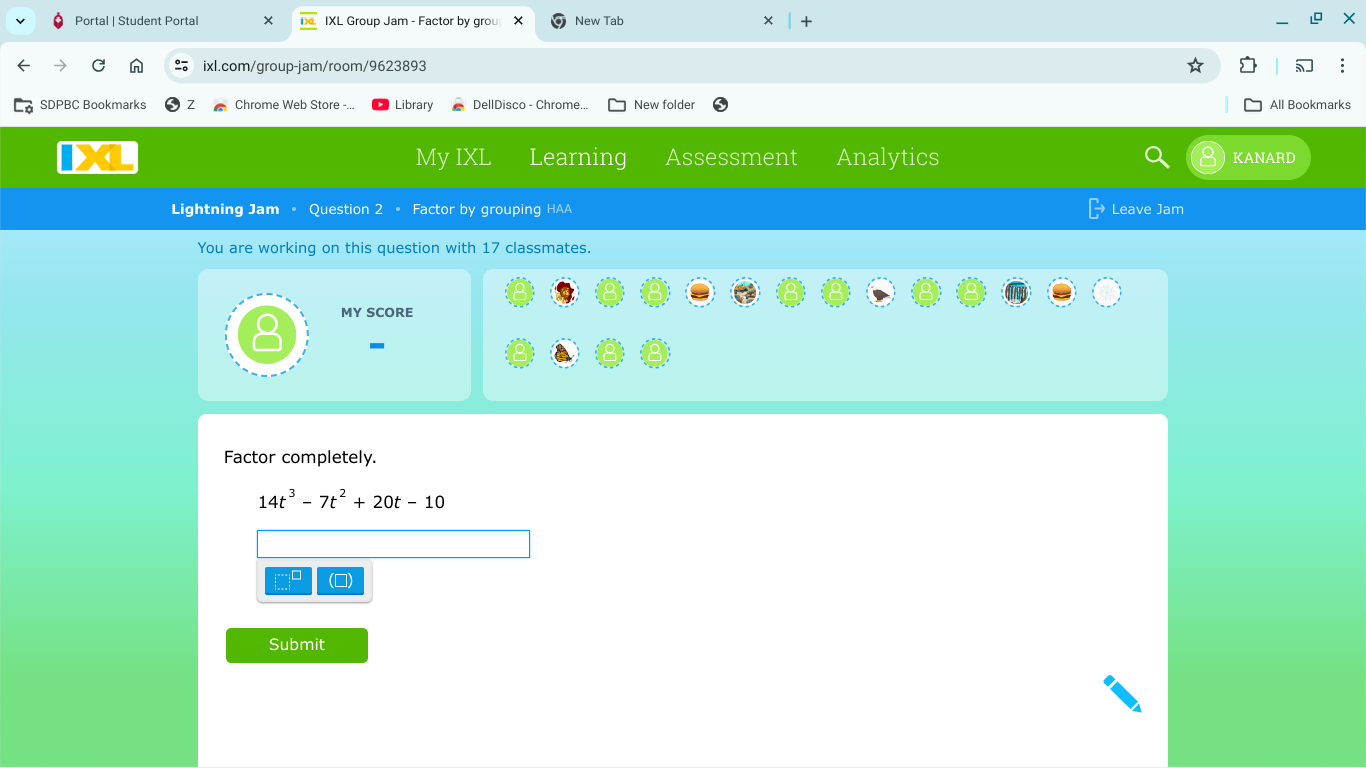
Factor completely. 14t³ - 7t² + 20t - 10

Understand the Problem

The question is asking how to completely factor the polynomial expression 14t³ - 7t² + 20t - 10. This involves finding common factors and possibly applying techniques such as grouping or using polynomial identities.

Answer

The completely factored form of the polynomial is $$(2t - 1)(7t^2 + 10)$$.

Steps to Solve

Factor out the greatest common factor (GCF)

First, we find the GCF of the coefficients (14, -7, 20, -10). The GCF is 1 (all coefficients share no larger common factor). We can decide to factor out a GCF of 1 for simplicity.

The expression remains: $$ 14t^3 - 7t^2 + 20t - 10 $$

Group the terms

Next, we group the polynomial in pairs: $$ (14t^3 - 7t^2) + (20t - 10) $$

Factor out the GCF from each group

Now, factor out the GCF from each group:

From the first group, $7t^2$ can be factored out: $$ 7t^2(2t - 1) $$
From the second group, $10$ can be factored out, but we need to remember the sign: $$ 10(2t - 1) $$

Now we can write the polynomial as: $$ 7t^2(2t - 1) + 10(2t - 1) $$

Factor out the common binomial

Notice that both terms have a common binomial factor $(2t - 1)$: $$ (2t - 1)(7t^2 + 10) $$

Now, we have completely factored the polynomial.

The completely factored form of the polynomial (14t^3 - 7t^2 + 20t - 10) is: $$(2t - 1)(7t^2 + 10)$$

More Information

Factoring polynomials is a fundamental skill in algebra. This specific polynomial featured coefficients that allowed for easy grouping and factoring. Understanding how to recognize patterns and apply the grouping method is crucial for higher-level math.

Tips

Ignoring the signs: When factoring out terms, always pay attention to the signs of the coefficients.
Forgetting to check for GCF: Some might forget to initially seek the GCF of the terms before grouping.

AI-generated content may contain errors. Please verify critical information

Thank you for voting!