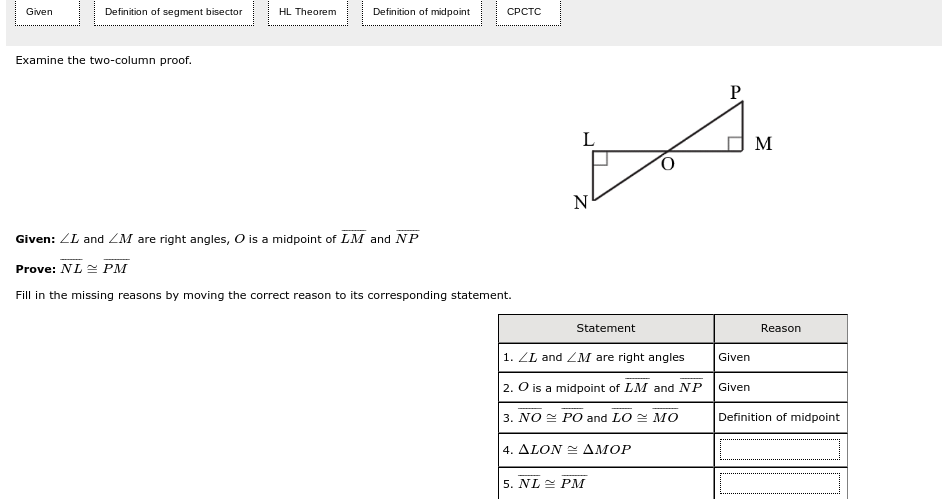
Examine the two-column proof. Given: ∠L and ∠M are right angles, O is a midpoint of LM and NP. Prove: NL ≅ PM. Fill in the missing reasons by moving the correct reason to its corre... Examine the two-column proof. Given: ∠L and ∠M are right angles, O is a midpoint of LM and NP. Prove: NL ≅ PM. Fill in the missing reasons by moving the correct reason to its corresponding statement.
Understand the Problem
The question is asking us to complete a two-column proof by filling in the missing reasons that correspond to given statements about right angles, midpoints, and triangle congruence.
Answer
1. Given 2. Given 3. Definition of midpoint 4. HL Theorem 5. CPCTC
Answer for screen readers
Fill in the reasons as follows:
-
( \angle L ) and ( \angle M ) are right angles. - Given
-
( O ) is a midpoint of ( \overline{LM} ) and ( \overline{NP} ). - Given
-
( NO \cong PO ) and ( LO \cong MO ). - Definition of midpoint
-
( \triangle LON \cong \triangle MOP ). - HL Theorem
-
( NL \cong PM ). - CPCTC
Steps to Solve
-
Identifying the Given Information
The given information states that angles ( \angle L ) and ( \angle M ) are right angles, and point ( O ) is the midpoint of segments ( \overline{LM} ) and ( \overline{NP} ). -
Filling in the First Given Statement
The first statement is " ( \angle L ) and ( \angle M ) are right angles." The reason is clearly marked as "Given." -
Filling in the Second Given Statement
The second statement " ( O ) is a midpoint of ( \overline{LM} ) and ( \overline{NP} )" also has the reason marked as "Given." -
Filling in the Third Statement
For the third statement " ( NO \cong PO ) and ( LO \cong MO )", the reason is "Definition of midpoint." This is because ( O ) being the midpoint means ( NO = PO ) and ( LO = MO ). -
Filling in the Fourth Statement
For the fourth statement " ( \triangle LON \cong \triangle MOP )", the reason is "HL Theorem" (Hypotenuse-Leg Theorem), which applies since both triangles are right triangles and have one leg equal (as shown by the segments). -
Filling in the Last Statement
For the last statement " ( NL \cong PM )", the reason is "CPCTC" (Corresponding Parts of Congruent Triangles are Congruent), which indicates that corresponding parts of congruent triangles are equal.
Fill in the reasons as follows:
-
( \angle L ) and ( \angle M ) are right angles. - Given
-
( O ) is a midpoint of ( \overline{LM} ) and ( \overline{NP} ). - Given
-
( NO \cong PO ) and ( LO \cong MO ). - Definition of midpoint
-
( \triangle LON \cong \triangle MOP ). - HL Theorem
-
( NL \cong PM ). - CPCTC
More Information
This proof utilizes common geometric principles and theorems, such as the definition of a midpoint, properties of right angles, and the Hypotenuse-Leg theorem. The CPCTC principle is fundamental in triangle congruence evaluations.
Tips
- Assuming that midpoints imply congruence of multiple segments without clarifying each step.
- Forgetting to apply the HL theorem properly, especially in identifying the relevant triangles.
- Mislabeling or skipping the proof structure, which can lead to gaps in reasoning.
AI-generated content may contain errors. Please verify critical information