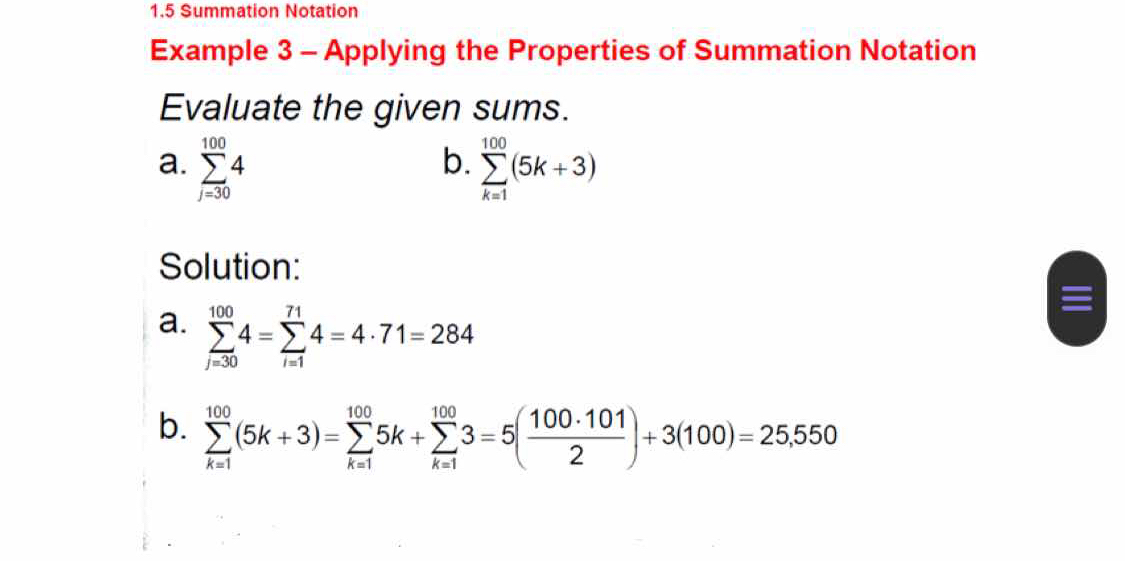
Evaluate the given sums: a. Σ from j=30 to 100 of 4. b. Σ from k=1 to 100 of (5k + 3).

Steps to Solve

1. Evaluate the first sum

To calculate the sum of the constant value (4) from (j=30) to (j=100):

The number of terms can be found by:

$$100 - 30 + 1 = 71$$

So the sum is:

$$\sum_{j=30}^{100} 4 = 4 \cdot 71 = 284$$

2. Evaluate the second sum

Now, we evaluate the sum of the expression (5k + 3) from (k=1) to (k=100).

This can be broken down into two parts:

$$\sum_{k=1}^{100} (5k + 3) = \sum_{k=1}^{100} 5k + \sum_{k=1}^{100} 3$$

3. Calculating the first part

First, calculate (\sum_{k=1}^{100} 5k):

Using the formula for the sum of the first (n) integers:

$$\sum_{k=1}^{n} k = \frac{n(n+1)}{2}$$

We apply this for (n=100):

$$\sum_{k=1}^{100} k = \frac{100(100 + 1)}{2} = \frac{100 \cdot 101}{2} = 5050$$

Thus,

$$\sum_{k=1}^{100} 5k = 5 \cdot 5050 = 25250$$

4. Calculating the second part

Next, calculate (\sum_{k=1}^{100} 3):

This is simply (3) multiplied by the number of terms:

$$\sum_{k=1}^{100} 3 = 3 \cdot 100 = 300$$

5. Combine both parts

Now combine the results of the two parts:

$$\sum_{k=1}^{100} (5k + 3) = 25250 + 300 = 25550$$