Evaluate ∫ from 0 to 1 of 1/(1+x^2) dx by using Simpson's 3/8 rule.
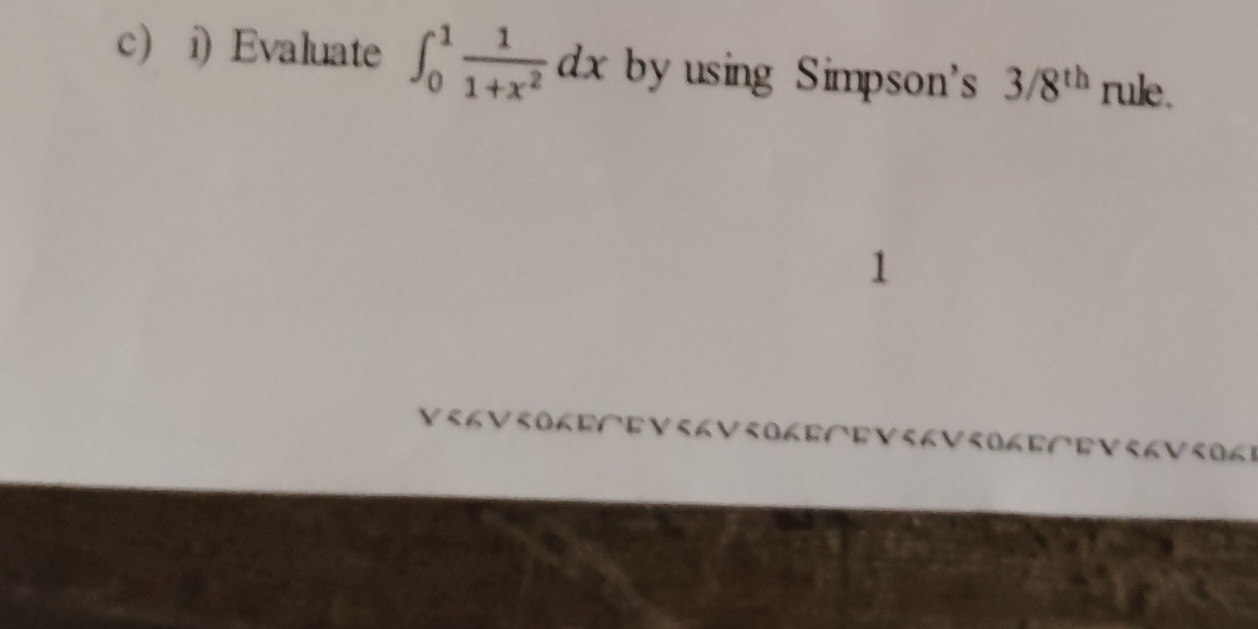
Understand the Problem
The question is asking to evaluate the integral of the function 1/(1+x^2) from 0 to 1 using Simpson's 3/8 rule. This involves applying numerical integration techniques to estimate the value of the definite integral.
Answer
The estimated value of the integral is $$ \frac{51}{65} $$
Answer for screen readers
The value of the integral is
$$ \frac{51}{65} $$
Steps to Solve
- Identify the integral and function We need to evaluate the integral
$$ \int_0^1 \frac{1}{1+x^2} , dx $$
using Simpson's 3/8 rule.
-
Determine the number of intervals (n) For Simpson's 3/8 rule, we need ( n ) to be a multiple of 3. In this case, let’s use ( n = 3 ) over the interval [0, 1]. This gives us ( h = \frac{b - a}{n} = \frac{1 - 0}{3} = \frac{1}{3} ).
-
Calculate the points The points at which we will evaluate the function are
- ( x_0 = 0 )
- ( x_1 = h = \frac{1}{3} )
- ( x_2 = 2h = \frac{2}{3} )
- ( x_3 = 1 )
- Evaluate the function at these points Calculate ( f(x) = \frac{1}{1 + x^2} ) at these points:
- ( f(0) = \frac{1}{1+0^2} = 1 )
- ( f\left(\frac{1}{3}\right) = \frac{1}{1+\left(\frac{1}{3}\right)^2} = \frac{1}{1+\frac{1}{9}} = \frac{1}{\frac{10}{9}} = \frac{9}{10} )
- ( f\left(\frac{2}{3}\right) = \frac{1}{1+\left(\frac{2}{3}\right)^2} = \frac{1}{1+\frac{4}{9}} = \frac{1}{\frac{13}{9}} = \frac{9}{13} )
- ( f(1) = \frac{1}{1+1^2} = \frac{1}{2} )
- Apply Simpson's 3/8 rule formula The formula for Simpson's 3/8 rule is:
$$ I \approx \frac{3h}{8} \left[ f(x_0) + 3f(x_1) + 3f(x_2) + f(x_3) \right] $$
Substituting in our values:
$$ I \approx \frac{3 \cdot \frac{1}{3}}{8} \left[ 1 + 3 \cdot \frac{9}{10} + 3 \cdot \frac{9}{13} + \frac{1}{2} \right] $$
- Calculate the integral value First calculate the coefficients:
$$ I \approx \frac{1}{8} \left[ 1 + \frac{27}{10} + \frac{27}{13} + \frac{1}{2} \right] $$
Finding a common denominator (130) for simplification:
- ( 1 = \frac{130}{130} )
- ( \frac{27}{10} = \frac{351}{130} )
- ( \frac{27}{13} = \frac{270}{130} )
- ( \frac{1}{2} = \frac{65}{130} )
So:
$$ I \approx \frac{1}{8} \left[ \frac{130 + 351 + 270 + 65}{130} \right] = \frac{1}{8} \left[ \frac{816}{130} \right] $$
Now simplifying:
$$ I \approx \frac{816}{1040} = \frac{204}{260} = \frac{102}{130} = \frac{51}{65} $$
- Final value The estimated value of the integral is
$$ \frac{51}{65} $$
The value of the integral is
$$ \frac{51}{65} $$
More Information
Simpson's 3/8 rule provides a numerical estimation for integrals, particularly useful for functions that are difficult to integrate analytically.
Tips
- Using an incorrect number of intervals: For Simpson’s 3/8 rule, ( n ) must be a multiple of 3.
- Miscalculating function values at each evaluation point.
- Forgetting to multiply by ( \frac{3h}{8} ) correctly.
AI-generated content may contain errors. Please verify critical information