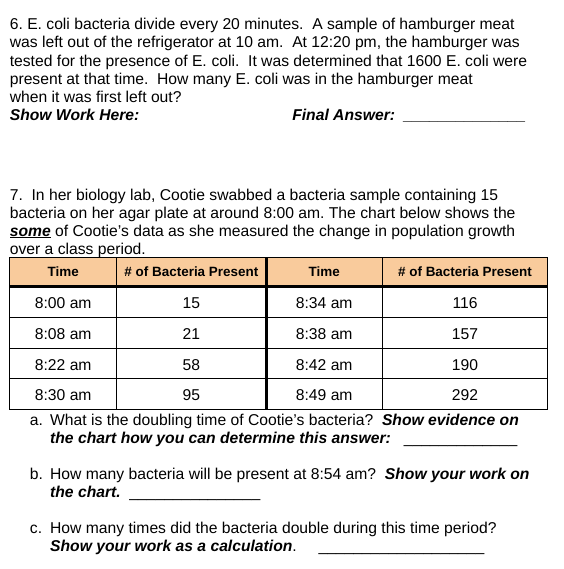
E. coli bacteria divide every 20 minutes. A sample of hamburger meat was left out of the refrigerator at 10 am. At 12:20 pm, the hamburger was tested and it was determined that 160... E. coli bacteria divide every 20 minutes. A sample of hamburger meat was left out of the refrigerator at 10 am. At 12:20 pm, the hamburger was tested and it was determined that 1600 E. coli were present at that time. How many E. coli were in the hamburger meat when it was first left out? Show Work Here: Final Answer: _____. In her biology lab, Cootie swabbed a bacteria sample containing 15 bacteria on her agar plate at around 8:00 am. The chart below shows some of Cootie's data as she measured the change in population growth over a class period. What is the doubling time of Cootie’s bacteria? Show evidence on the chart how you can determine this answer: ____. How many bacteria will be present at 8:54 am? Show your work on the chart: ____. How many times did the bacteria double during this time period? Show your work as a calculation: _____.
Understand the Problem
The question is asking for calculations related to E. coli bacterial growth based on provided data points. Specifically, it involves determining the initial population of E. coli in hamburger meat and answering several questions regarding bacterial growth rates and population projections based on the data provided in the chart.
Answer
The initial number of E. coli in the hamburger was approximately \(13\).
Answer for screen readers
The initial population of E. coli in the hamburger meat when it was first left out is ( P_0 = 13 ).
Steps to Solve
-
Determine the growth period of E. coli
The E. coli bacteria double every 20 minutes. From 10:00 am to 12:20 pm (the time of testing) is a total of 2 hours and 20 minutes, which is equivalent to:
$$ 2 \text{ hours} \times 60 \text{ minutes/hour} + 20 \text{ minutes} = 140 \text{ minutes} $$
To find out how many 20-minute intervals are in 140 minutes, calculate:
$$ \frac{140 \text{ minutes}}{20 \text{ minutes/interval}} = 7 \text{ intervals} $$
-
Calculate the initial population
If 1600 E. coli were present at the time of testing, we can determine the initial population (denoted as (P_0)) using the formula for exponential growth:
$$ P = P_0 \times 2^n $$
where (P) is the final population, (P_0) is the initial population, and (n) is the number of intervals.
Plugging in our values:
$$ 1600 = P_0 \times 2^7 $$
-
Solve for (P_0)
Calculate (2^7):
$$ 2^7 = 128 $$
Then substitute and solve for (P_0):
$$ P_0 = \frac{1600}{128} = 12.5 $$
Since we cannot have half a bacterium in context, we'll round up to:
$$ P_0 = 13 $$
The initial population of E. coli in the hamburger meat when it was first left out is ( P_0 = 13 ).
More Information
The calculation shows that the initial population of bacteria doubles every 20 minutes. Each interval creates a significant increase, illustrating the rapid growth capabilities of bacteria like E. coli under favorable conditions.
Tips
- Forgetting to convert hours into minutes.
- Miscalculating the number of doubling intervals, which can lead to incorrect estimates of initial populations.
- Rounding down the population to a non-integer.
AI-generated content may contain errors. Please verify critical information