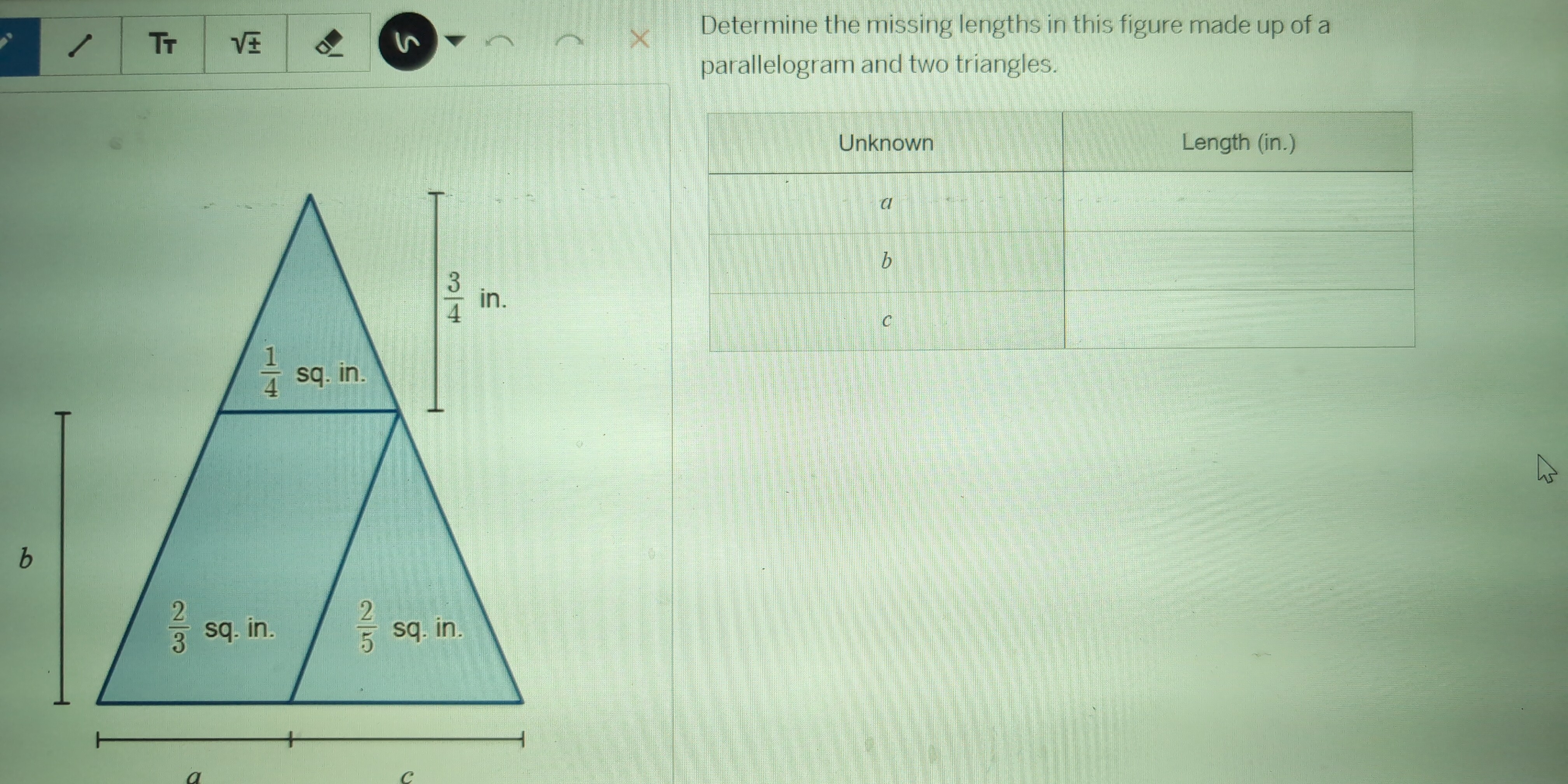
Determine the missing lengths in this figure made up of a parallelogram and two triangles.

Steps to Solve

1. Determine the total area of the triangles The total area of the top triangle and the bottom triangle can be calculated by adding the given areas: $$ \text{Total Area} = \frac{1}{4} + \frac{2}{3} + \frac{2}{5} $$

2. Find a common denominator To sum these fractions, we need a common denominator. The least common multiple of 4, 3, and 5 is 60.

Convert each fraction:

• $$ \frac{1}{4} = \frac{15}{60} $$
• $$ \frac{2}{3} = \frac{40}{60} $$
• $$ \frac{2}{5} = \frac{24}{60} $$

3. Calculate the total area Now sum the converted fractions: $$ \frac{15}{60} + \frac{40}{60} + \frac{24}{60} = \frac{15 + 40 + 24}{60} = \frac{79}{60} $$

4. Calculate the height of the triangles Because the height of the triangles is given as ( \frac{3}{4} ) in., we can use this to find the values of ( a ), ( b ), and ( c ).

5. Solving for the base using the area formula For the area of a triangle: $$ \text{Area} = \frac{1}{2} \times \text{base} \times \text{height} $$ We set our area equal to the calculated area for both triangles.

For the triangle with area ( \frac{1}{4} ): $$ \frac{1}{4} = \frac{1}{2} \times a \times \frac{3}{4} $$ Solving for ( a ): $$ a = \frac{1/4 \times 2}{(3/4)} = \frac{2}{3} $$

6. Solve for ( b ) and ( c ) similarly Using the area formulas similarly for the other areas: For ( b ) with: $$ b = \frac{2/3 \times 2}{(3/4)} = \frac{8}{9} $$

And for ( c ) with: $$ c = \frac{2/5 \times 2}{(3/4)} = \frac{8}{15} $$