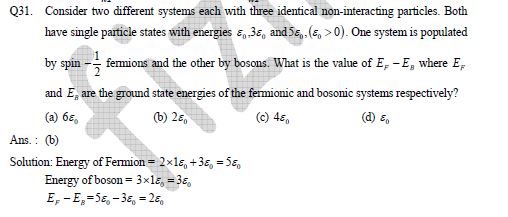
Consider two different systems each with three identical non-interacting particles. Both have single particle states with energies ε_0, 3ε_0, and 5ε_0 (ε_0 > 0). One system is popu... Consider two different systems each with three identical non-interacting particles. Both have single particle states with energies ε_0, 3ε_0, and 5ε_0 (ε_0 > 0). One system is populated by spin-1/2 fermions and the other by bosons. What is the value of E_f - E_b, where E_f and E_b are the ground state energies of the fermionic and bosonic systems respectively?
Understand the Problem
The question is asking to determine the difference in ground state energies of two systems: one containing fermions and the other containing bosons. The approach is to calculate the total energies for both systems using the given particle energies and identify the relationship between the ground state energies E_f and E_b.
Answer
The difference in ground state energies \(E_f - E_b\) is \(2ε_0\).
Answer for screen readers
The value of (E_f - E_b) is (2ε_0).
Steps to Solve
- Calculate Ground State Energy for Fermions Fermions follow the Pauli exclusion principle, meaning no two fermions can occupy the same state. For three spin-1/2 fermions, we can occupy the lowest three available states (i.e., $ε_0$, $ε_1$, and $ε_2$):
[ E_f = ε_0 + ε_1 + ε_2 = ε_0 + 3ε_0 + 5ε_0 = 2ε_0 + 3ε_0 ]
- Formulate Energy for Bosons Bosons do not follow the exclusion principle, so all three particles can occupy the lowest single particle energy state, which is $ε_0$. Thus, the ground state energy for the bosons can be calculated as:
[ E_b = 3ε_0 ]
- Find the Difference in Energies Now we can compute the difference between the ground state energies of fermions and bosons:
[ E_f - E_b = (2ε_0 + 3ε_0) - 3ε_0 ]
- Calculate the Final Difference Simplifying this results in:
[ E_f - E_b = 2ε_0 ]
The value of (E_f - E_b) is (2ε_0).
More Information
Fermions and bosons exhibit different statistical behaviors leading to their distinct ground state energies. Fermions fill up the lowest energy states following the Pauli exclusion principle, while bosons can all reside in the same state, making their ground state energy lower in this case.
Tips
- Miscalculating the energies by omitting the proper occupation of states for fermions and bosons.
- Confusing the energies due to not applying the exclusion principle correctly for fermions.
AI-generated content may contain errors. Please verify critical information