Complete the square to re-write the quadratic function in vertex form: y = x^2 + 3x + 4
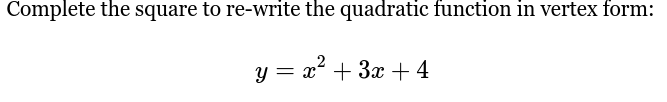
Understand the Problem
The question is asking to complete the square for the given quadratic function in order to re-write it in vertex form. This involves manipulating the equation to identify the vertex of the parabola represented by the quadratic function.
Answer
The vertex form of the quadratic function is $$ y = \left(x + \frac{3}{2}\right)^2 + \frac{7}{4} $$
Answer for screen readers
The vertex form of the quadratic function is
$$ y = \left(x + \frac{3}{2}\right)^2 + \frac{7}{4} $$
Steps to Solve
-
Identify the quadratic expression
The given function is $y = x^2 + 3x + 4$. We need to focus on the terms $x^2 + 3x$ to complete the square. -
Factor out the coefficient of $x^2$ (if necessary)
In this case, the coefficient of $x^2$ is 1, so we can proceed without factoring. -
Find the term to complete the square
To find the term that completes the square, take half of the coefficient of $x$ (which is 3), square it, and add/subtract that value.
Half of 3 is $\frac{3}{2}$, and squaring it gives:
$$ \left(\frac{3}{2}\right)^2 = \frac{9}{4} $$ -
Rewrite the quadratic expression
Now, we can write the function as:
$$ y = (x^2 + 3x + \frac{9}{4}) + 4 - \frac{9}{4} $$
This simplifies to:
$$ y = \left(x + \frac{3}{2}\right)^2 + \frac{16}{4} - \frac{9}{4} $$ -
Simplify the constant terms
Now combine the constants:
$$ \frac{16}{4} - \frac{9}{4} = \frac{7}{4} $$
Thus, the function becomes:
$$ y = \left(x + \frac{3}{2}\right)^2 + \frac{7}{4} $$ -
Write the final vertex form
The vertex form of the quadratic function is:
$$ y = \left(x + \frac{3}{2}\right)^2 + \frac{7}{4} $$
where the vertex is at $\left(-\frac{3}{2}, \frac{7}{4}\right)$.
The vertex form of the quadratic function is
$$ y = \left(x + \frac{3}{2}\right)^2 + \frac{7}{4} $$
More Information
The vertex form of a quadratic function highlights the vertex of the parabola. Here, the vertex is at $\left(-\frac{3}{2}, \frac{7}{4}\right)$. This form is useful for graphing and understanding the properties of the parabola.
Tips
- Forgetting to adjust the constant after completing the square.
- Not squaring the half of the coefficient correctly. Always ensure to calculate $\left(\frac{b}{2}\right)^2$ properly.
AI-generated content may contain errors. Please verify critical information