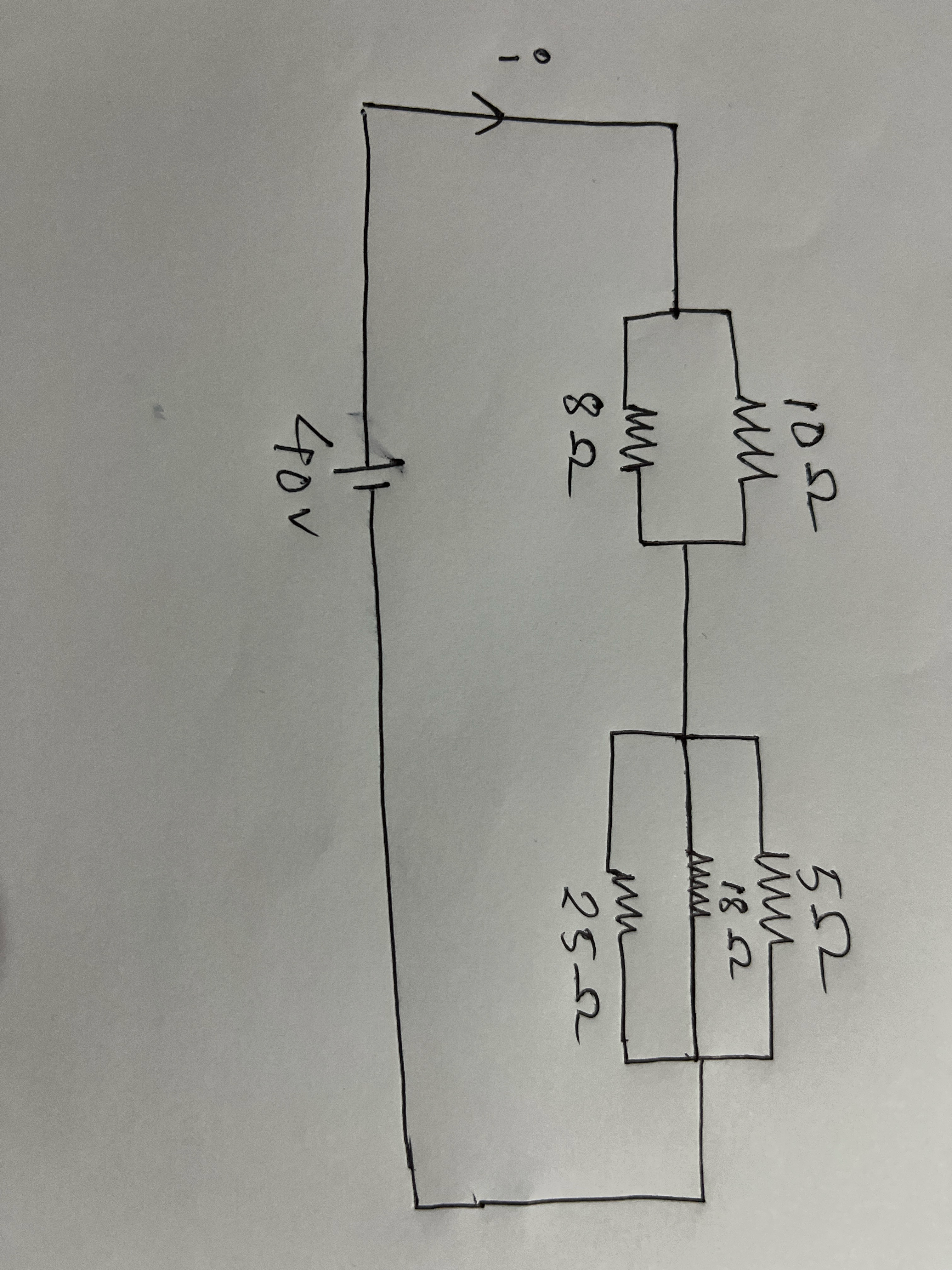
Calculate the total resistance of the circuit.
Understand the Problem
The question involves analyzing an electrical circuit with several resistors connected in series and parallel. We need to calculate the total resistance or other electrical characteristics based on the resistor values provided and the voltage source.
Answer
The total resistance of the circuit is approximately \( R_{total} \approx 25.47 \, \Omega \).
Answer for screen readers
The total resistance of the circuit is approximately ( R_{total} \approx 25.47 , \Omega ).
Steps to Solve
- Identify the components of the circuit
The circuit consists of a 40V voltage source and several resistors: 5Ω, 10Ω, 8Ω, 18Ω, and 25Ω.
- Group the resistors
The resistors are connected as follows:
- The 10Ω and 5Ω are in series.
- The 18Ω and 25Ω resistors are in parallel.
- Calculate the equivalent resistance of the series resistors
For resistors in series, the total resistance ($R_{total, series}$) is calculated as:
$$ R_{total, series} = R_1 + R_2 $$
Here, $R_1 = 10Ω$ and $R_2 = 5Ω$:
$$ R_{total, series} = 10 + 5 = 15Ω $$
- Calculate the equivalent resistance of the parallel resistors
For resistors in parallel, the total resistance ($R_{total, parallel}$) is calculated using the formula:
$$ \frac{1}{R_{total, parallel}} = \frac{1}{R_3} + \frac{1}{R_4} $$
Here, $R_3 = 18Ω$ and $R_4 = 25Ω$:
$$ \frac{1}{R_{total, parallel}} = \frac{1}{18} + \frac{1}{25} $$
Calculating this gives:
$$ \frac{1}{R_{total, parallel}} = \frac{25 + 18}{450} = \frac{43}{450} $$
Therefore:
$$ R_{total, parallel} = \frac{450}{43} \approx 10.47Ω $$
- Combine the equivalent resistances
Now, we can find the total resistance ($R_{total}$) of the entire circuit by adding the series and parallel resistances:
$$ R_{total} = R_{total, series} + R_{total, parallel} $$
Substituting the values we calculated:
$$ R_{total} = 15 + 10.47 \approx 25.47Ω $$
The total resistance of the circuit is approximately ( R_{total} \approx 25.47 , \Omega ).
More Information
This total resistance helps determine how the circuit reacts to the voltage supply. The total current can be calculated using Ohm's Law, where ( I = \frac{V}{R} ).
Tips
- Forgetting to convert all resistor values to the same unit (e.g., ohms).
- Miscounting which resistors are in series and which are in parallel.
- Incorrectly applying the formulas for series and parallel combinations.
AI-generated content may contain errors. Please verify critical information