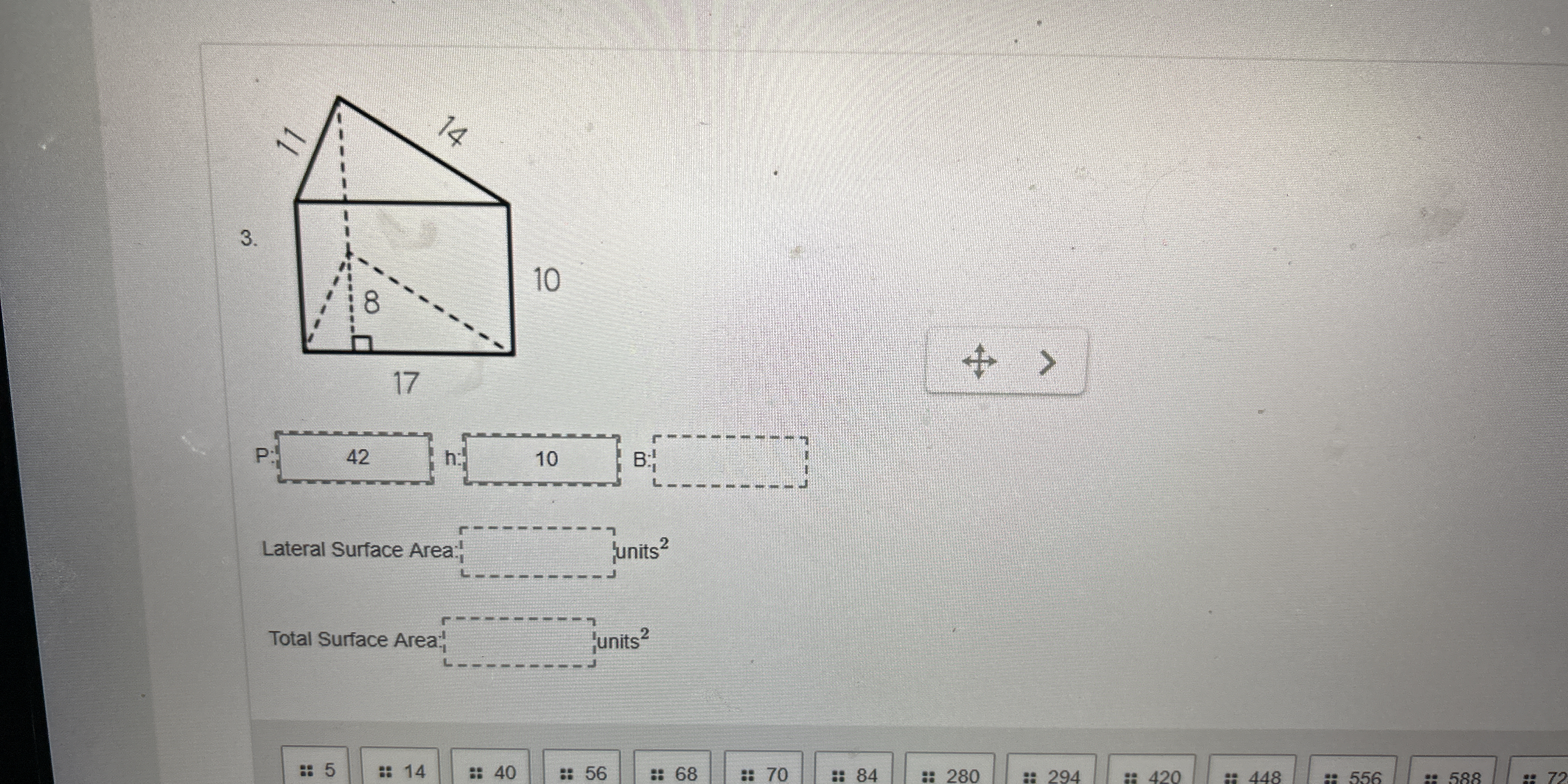
Calculate the lateral surface area and total surface area of the given house-shaped figure.

Steps to Solve

1. Identify Dimensions of the House-shaped Figure
   The house has the following dimensions:

• Length of the rectangular part: ( 17 ) units
• Width of the rectangular part: ( 10 ) units

For the triangular part of the house, we have:

• Height of the triangle: ( 11 ) units
• Base of the triangle (the same as the length of the house): ( 17 ) units

2. Calculate Lateral Surface Area
   The lateral surface area of the house consists of the walls of the rectangular part and the two sides of the triangular part. The lateral area is calculated as follows:

• Rectangular part:

  • Two identical rectangular sides: Each has an area ( A_r = \text{Length} \times \text{Height} = 17 \times 10 )

• Triangular part:

  • The two triangular sides: Each has an area ( A_t = \frac{1}{2} \times \text{Base} \times \text{Height} = \frac{1}{2} \times 17 \times 11 )

So the total lateral surface area is:
$$ Lateral , Surface , Area = 2A_r + 2A_t $$

3. Calculate Total Surface Area
   The total surface area includes the lateral area plus the area of the roof and the base. Since the roof is a triangle, we calculate:

• Roof area (same as the triangular part):
  $$ A_{\text{roof}} = \frac{1}{2} \times \text{Base} \times \text{Height} $$

• Base area (perhaps assumed to be the rectangular base):
  $$ A_{\text{base}} = \text{Length} \times \text{Width} = 17 \times 10 $$

Thus, the total surface area is calculated as:
$$ Total , Surface , Area = Lateral , Surface , Area + A_{\text{roof}} + A_{\text{base}} $$

4. Substituting and Calculating Values
   Substitute calculated values into the equations:

• Area of rectangular sides:
  $$ A_r = 17 \times 10 = 170 $$
• Area of triangular sides:
  $$ A_t = \frac{1}{2} \times 17 \times 11 = 93.5 $$

Combine these:
$$ Lateral , Surface , Area = 2(170) + 2(93.5) = 340 + 187 = 527 , \text{units}^2 $$

• Area of the roof:
  $$ A_{\text{roof}} = 93.5 , \text{units}^2 $$
• Area of the base:
  $$ A_{\text{base}} = 170 , \text{units}^2 $$

Finally, calculate total surface area:
$$ Total , Surface , Area = 527 + 93.5 + 170 = 790.5 , \text{units}^2 $$