Calculate the area of the shape.
Understand the Problem
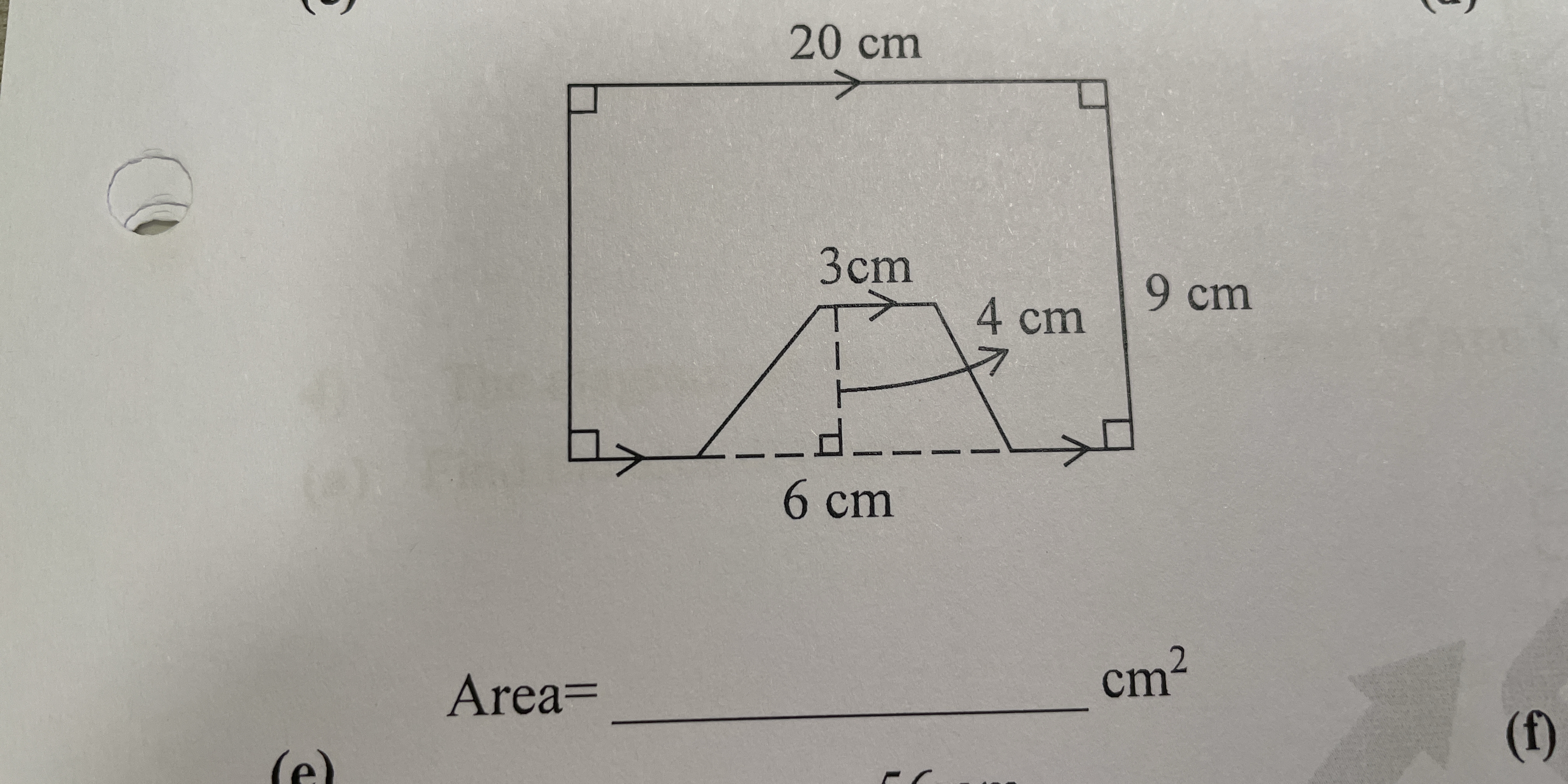
The question is asking to calculate the area of a given shape, which appears to be a combination of a rectangle and a trapezoid. We'll likely need to break down the shape into its parts to find the total area.
Answer
The area is $220 \, \text{cm}²$.
Answer for screen readers
The total area is $220 , \text{cm}²$.
Steps to Solve
- Identify the shape components
The shape consists of a rectangle (20 cm by 9 cm) combined with a trapezoid at the top.
- Calculate the area of the rectangle
The area of a rectangle is calculated using the formula: $$ \text{Area} = \text{length} \times \text{width} $$ For our rectangle: $$ \text{Area}_{\text{rectangle}} = 20 , \text{cm} \times 9 , \text{cm} = 180 , \text{cm}² $$
- Identify the trapezoid dimensions
The trapezoid appears to have bases of 6 cm and a top base that is determined by the top width (the remaining part of the rectangle after accounting for the sides, which are 3 cm each). Therefore, the top base is: $$ \text{Top base} = 20 , \text{cm} - (3 , \text{cm} + 3 , \text{cm}) = 14 , \text{cm} $$
The height of the trapezoid is 4 cm.
- Calculate the area of the trapezoid
The area of a trapezoid is calculated using the formula: $$ \text{Area} = \frac{1}{2} \times (\text{Base}_1 + \text{Base}2) \times \text{Height} $$ For our trapezoid: $$ \text{Area}{\text{trapezoid}} = \frac{1}{2} \times (6 , \text{cm} + 14 , \text{cm}) \times 4 , \text{cm} $$
Calculating it step by step gives: $$ \text{Area}_{\text{trapezoid}} = \frac{1}{2} \times 20 , \text{cm} \times 4 , \text{cm} = 40 , \text{cm}² $$
- Calculate the total area
Finally, add the areas of both shapes: $$ \text{Total Area} = \text{Area}{\text{rectangle}} + \text{Area}{\text{trapezoid}} $$ $$ \text{Total Area} = 180 , \text{cm}² + 40 , \text{cm}² = 220 , \text{cm}² $$
The total area is $220 , \text{cm}²$.
More Information
The area consists of both the rectangle and the trapezoid, showcasing how composite shapes can be analyzed mathematically. Understanding this method can help in more complex geometric calculations in the future.
Tips
- Forgetting to account for both components of the shape (rectangle and trapezoid).
- Miscalculating the dimensions of the trapezoid bases. It's essential to carefully check the segments when determining the bases.
AI-generated content may contain errors. Please verify critical information