Calculate T(f)''(1).
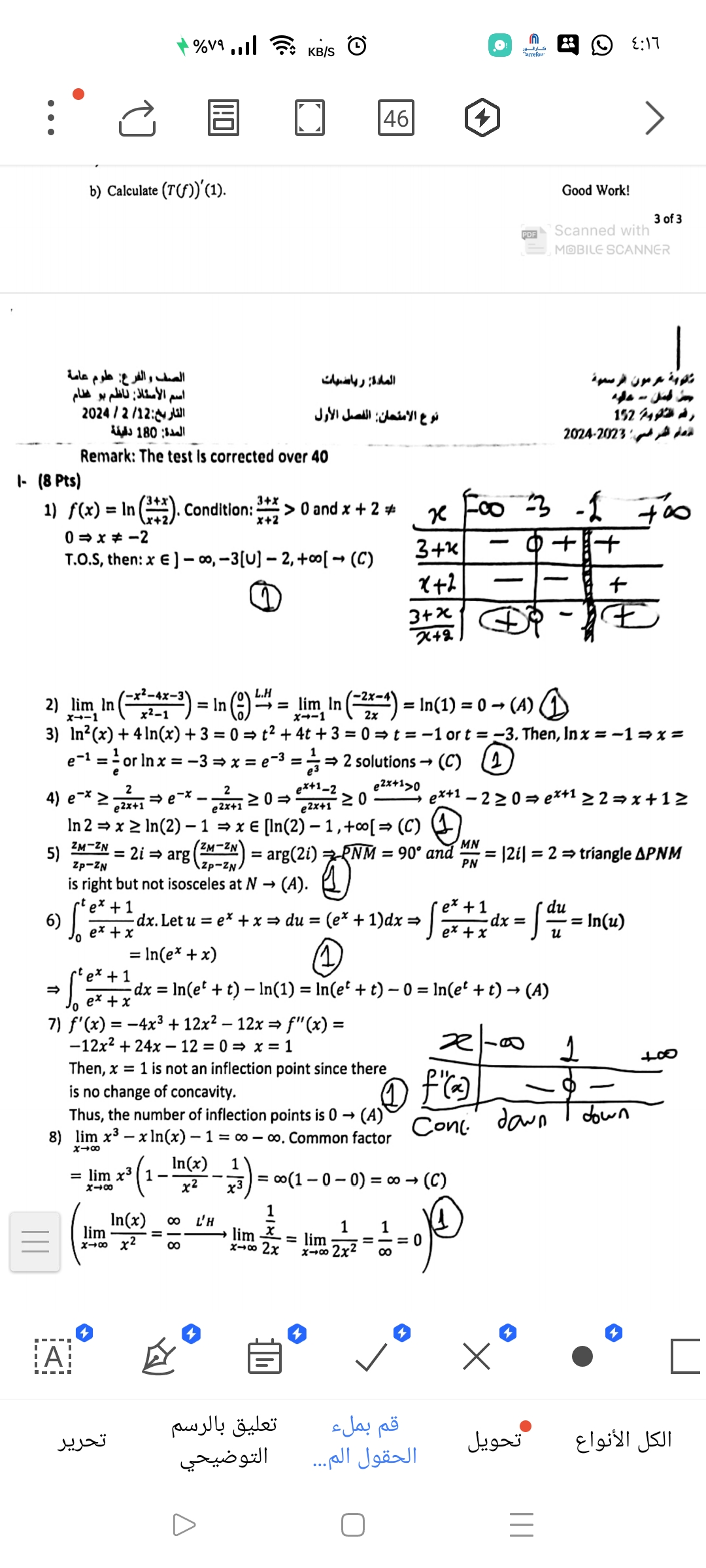
Understand the Problem
The question is asking to calculate a specific value of the function denoted as 'T(f)''(1)', which implies that it relates to the evaluation of a derivative or a transformation of the function at a particular point.
Answer
The value of \( T(f)''(1) \) is \( \frac{2}{27} \).
Answer for screen readers
The final answer is
$$ T(f)''(1) = \frac{2}{27} $$
Steps to Solve
- Identify the function and its parameters
Given that we need to calculate $T(f)''(1)$ from the provided notation, we first need to compute the first derivative of the function ( f(x) = \frac{3+x}{x+2} ).
- Calculate the first derivative of ( f(x) )
Using the quotient rule for differentiation, where if ( f(x) = \frac{g(x)}{h(x)} ), then
$$ f'(x) = \frac{g'(x)h(x) - g(x)h'(x)}{(h(x))^2} $$
Here, ( g(x) = 3+x ) and ( h(x) = x+2 ).
- Calculate ( g'(x) = 1 ) and ( h'(x) = 1 ).
- Thus,
$$ f'(x) = \frac{(1)(x+2) - (3+x)(1)}{(x+2)^2} $$
- Simplifying this gives:
$$ f'(x) = \frac{x + 2 - 3 - x}{(x+2)^2} = \frac{-1}{(x+2)^2} $$
- Calculate the second derivative of ( f(x) )
Next, differentiate ( f'(x) ) again to find ( f''(x) ):
Using the quotient rule again:
$$ f''(x) = \frac{(0)(x+2)^2 - (-1)(2(x+2)(1))}{(x+2)^4} $$
- Simplifying gives:
$$ f''(x) = \frac{2(x+2)}{(x+2)^4} = \frac{2}{(x+2)^3} $$
- Evaluate ( f''(1) )
Substituting ( x = 1 ):
$$ f''(1) = \frac{2}{(1+2)^3} = \frac{2}{3^3} = \frac{2}{27} $$
So, ( T(f)''(1) = f''(1) = \frac{2}{27} ).
The final answer is
$$ T(f)''(1) = \frac{2}{27} $$
More Information
The derivative we calculated, ( T(f)''(1) ), represents the acceleration or concavity of the function at the point ( x = 1 ). Derivatives provide insight into the behavior of functions, such as identifying concave up or down regions.
Tips
- Forgetting the quotient rule: It's essential to apply the quotient rule correctly when differentiating fractions.
- Simplification errors: Be sure to carefully simplify derivatives to avoid errors in subsequent calculations.
AI-generated content may contain errors. Please verify critical information