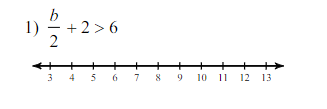
b/2 + 2 > 6
Understand the Problem
The question is a mathematical inequality that is asking to solve for the variable b such that the expression (b/2) + 2 is greater than 6. This will require isolating b and determining the solution set.
Answer
The solution to the inequality is \( b > 8 \).
Answer for screen readers
The solution to the inequality ( \frac{b}{2} + 2 > 6 ) is ( b > 8 ).
Steps to Solve
-
Isolate the variable term To solve the inequality ( \frac{b}{2} + 2 > 6 ), we first subtract 2 from both sides: $$ \frac{b}{2} > 6 - 2 $$ This simplifies to: $$ \frac{b}{2} > 4 $$
-
Eliminate the fraction Next, we multiply both sides of the inequality by 2 to eliminate the fraction. Remember that multiplying or dividing by a positive number does not change the inequality direction: $$ b > 4 \times 2 $$ This gives us: $$ b > 8 $$
-
Write the solution set The solution set is all values of ( b ) that are greater than 8. In interval notation, this is expressed as: $$ (8, \infty) $$
The solution to the inequality ( \frac{b}{2} + 2 > 6 ) is ( b > 8 ).
More Information
In this inequality, we found that ( b ) must be greater than 8 to satisfy the condition. This means any number larger than 8 is a valid solution. The graph of this inequality would typically be represented with an open circle at 8 and a shaded line extending to the right towards infinity.
Tips
- Mistake in sign handling: Some may forget that the inequality symbol flips when multiplying or dividing by a negative number, but that wasn’t necessary here.
- Incorrectly interpreting the solution: Choosing values not greater than 8 can lead to misunderstanding the solution set.
AI-generated content may contain errors. Please verify critical information