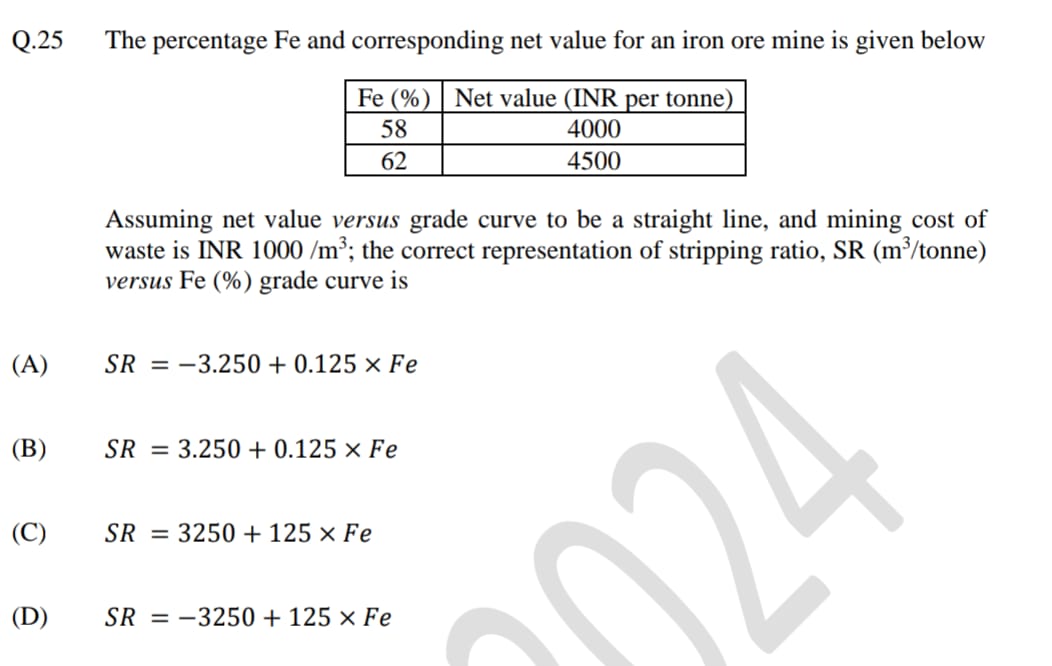
Assuming net value versus grade curve to be a straight line, and mining cost of waste is INR 1000/m³; the correct representation of stripping ratio, SR (m³/tonne) versus Fe (%) gra... Assuming net value versus grade curve to be a straight line, and mining cost of waste is INR 1000/m³; the correct representation of stripping ratio, SR (m³/tonne) versus Fe (%) grade curve is?
Understand the Problem
The question is asking to determine the correct linear relationship between the stripping ratio (SR) and the percentage of iron (Fe) based on given net value data and a constant mining cost. This involves interpreting the data provided and selecting the correct equation from the options given.
Answer
The correct representation of stripping ratio, SR (m³/tonne) versus Fe (%) grade curve is: $$ SR = -3250 + 125 \cdot Fe $$
Answer for screen readers
The correct representation of stripping ratio, SR (m³/tonne) versus Fe (%) grade curve is:
$$ SR = -3250 + 125 \cdot Fe $$
Steps to Solve
- Identify the given data
The problem provides two values, where the percentage of iron (Fe) and the corresponding net values are given as follows:
- For 58% Fe: Net value = 4000 INR/tonne
- For 62% Fe: Net value = 4500 INR/tonne
- Calculate the slope (m)
To find the slope of the line, we use the formula for the slope $m$ between two points $(x_1, y_1)$ and $(x_2, y_2)$:
$$ m = \frac{y_2 - y_1}{x_2 - x_1} $$
Here, let $y_1 = 4000$, $y_2 = 4500$, $x_1 = 58$, and $x_2 = 62$:
$$ m = \frac{4500 - 4000}{62 - 58} = \frac{500}{4} = 125 $$
- Determine the y-intercept (b)
Now, we can use one of the points to find the y-intercept $b$ using the linear equation formula:
$$ y = mx + b $$
Using the point (58, 4000):
$$ 4000 = 125 \cdot 58 + b $$
Calculate $125 \cdot 58 = 7250$, then:
$$ b = 4000 - 7250 = -3250 $$
- Form the linear equation
Now that we have both the slope and the intercept, we can write the linear relationship:
$$ SR = mx + b = 125 \cdot Fe - 3250 $$
Rearranging gives us:
$$ SR = -3250 + 125 \cdot Fe $$
The correct representation of stripping ratio, SR (m³/tonne) versus Fe (%) grade curve is:
$$ SR = -3250 + 125 \cdot Fe $$
More Information
In linear equations, the form $y = mx + b$ is used, where $m$ is the slope indicating the change in $y$ for a change in $x$, and $b$ is the y-intercept representing the value of $y$ when $x$ is zero. Here, the stripping ratio increases as the percentage of iron increases.
Tips
- Confusing the roles of $x$ and $y$. Remember that in this context, Fe percentage is your $x$, and the stripping ratio is your $y$.
- Incorrectly calculating the slope or the y-intercept. Always double-check your arithmetic.
AI-generated content may contain errors. Please verify critical information