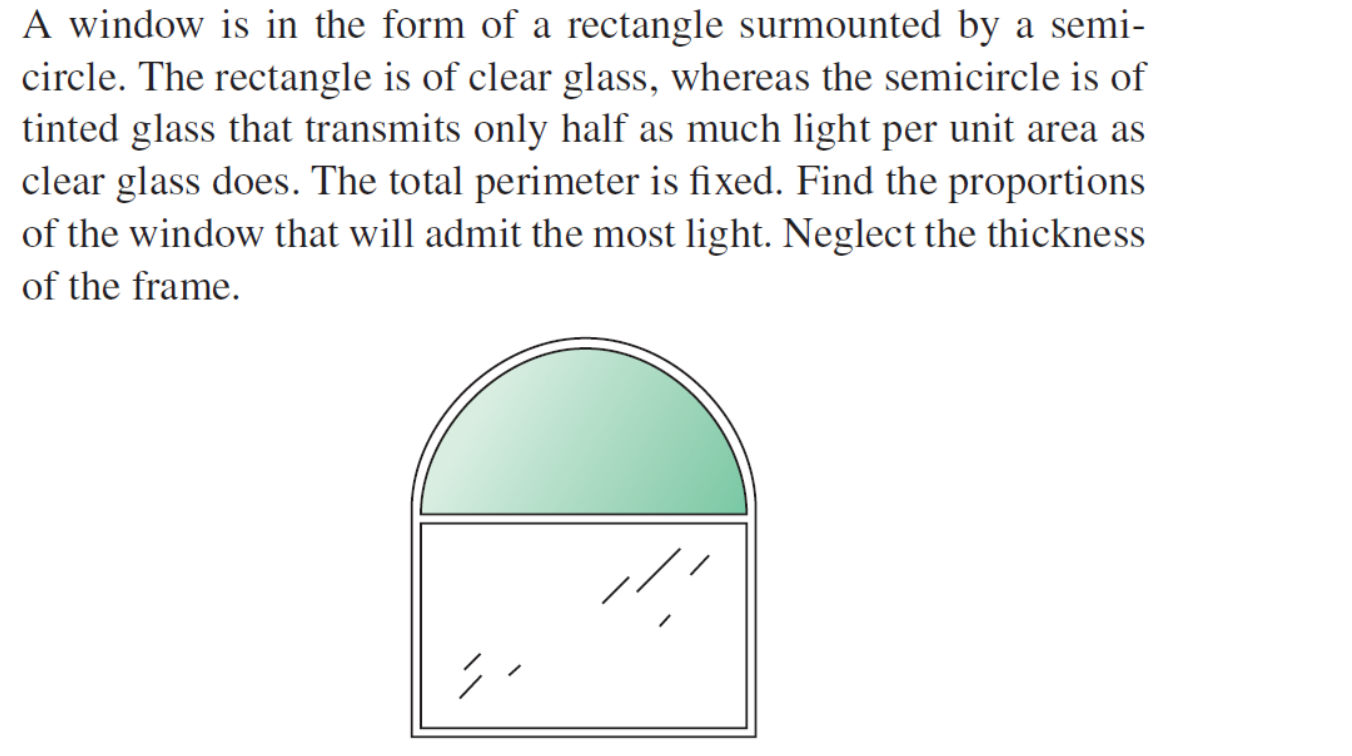
A window is in the form of a rectangle surmounted by a semi-circle. The rectangle is of clear glass, whereas the semicircle is of tinted glass that transmits only half as much ligh... A window is in the form of a rectangle surmounted by a semi-circle. The rectangle is of clear glass, whereas the semicircle is of tinted glass that transmits only half as much light per unit area as clear glass does. The total perimeter is fixed. Find the proportions of the window that will admit the most light. Neglect the thickness of the frame.
Understand the Problem
The question is asking for the optimal dimensions of a window that consists of a rectangle and a semicircle, aiming to maximize the area that admits light while considering the difference in light transmission between clear and tinted glass. We need to analyze the geometry of the shapes and apply constraints from the fixed perimeter.
Answer
The optimal proportions are \( w = \frac{2P}{\pi + 4} \) and \( h = \frac{P(2 - \pi)}{2(\pi + 4)} \).
Answer for screen readers
The proportions of the window that will admit the most light are ( w = \frac{2P}{\pi + 4} ) for the width and ( h = \frac{P(2 - \pi)}{2(\pi + 4)} ) for the height.
Steps to Solve
-
Define Variables Let ( w ) be the width of the rectangle and ( h ) be its height. The radius of the semicircle will be ( r = \frac{w}{2} ).
-
Perimeter Constraint The total perimeter ( P ) of the window can be expressed as: $$ P = w + 2h + \pi r $$ Substituting ( r ): $$ P = w + 2h + \pi \left(\frac{w}{2}\right) = w + 2h + \frac{\pi w}{2} $$
-
Expressing h in terms of w Rearranging the perimeter equation to solve for ( h ): $$ 2h = P - w - \frac{\pi w}{2} $$ $$ h = \frac{P - w - \frac{\pi w}{2}}{2} $$
-
Area Calculation The area ( A ) of the window will be: $$ A = \text{Area of Rectangle} + \text{Area of Semicircle} $$ $$ A = w \cdot h + \frac{1}{2} \pi r^2 $$ Substituting ( r ): $$ A = w \cdot h + \frac{1}{2} \pi \left(\frac{w}{2}\right)^2 = w \cdot h + \frac{\pi w^2}{8} $$
-
Substituting h in Area Now, substituting the expression for ( h ) into the area formula: $$ A = w \cdot \left(\frac{P - w - \frac{\pi w}{2}}{2}\right) + \frac{\pi w^2}{8} $$
-
Maximizing Area Differentiate ( A ) with respect to ( w ) and set the derivative equal to zero to find the maximum area: $$ A'(w) = 0 $$
-
Solve for w and h After solving the derivative, find the value of ( w ) that maximizes ( A ), and substitute back to find ( h ).
The proportions of the window that will admit the most light are ( w = \frac{2P}{\pi + 4} ) for the width and ( h = \frac{P(2 - \pi)}{2(\pi + 4)} ) for the height.
More Information
This solution considers the areas with the difference in light transmission between clear and tinted glass. The optimization approach ensures we find the best dimensions for maximizing useful light admitted through the window.
Tips
- Forgetting to account for the difference in light transmission for the tinted glass while calculating the effective area.
- Not correctly applying calculus, such as differentiating the area correctly or simplifying the derivative.
AI-generated content may contain errors. Please verify critical information