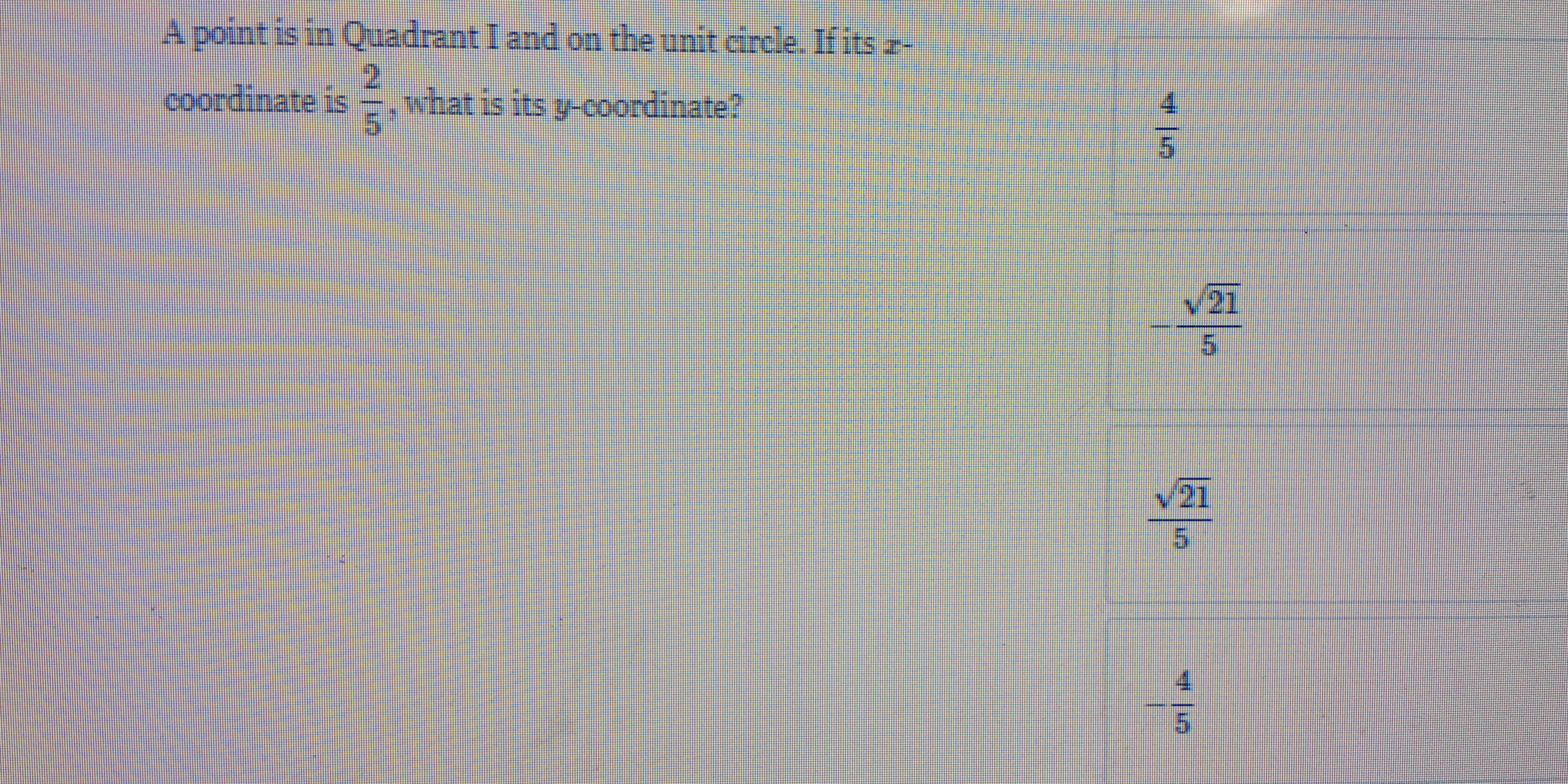
A point is in Quadrant I and on the unit circle. If its x-coordinate is 2/5, what is its y-coordinate?
Understand the Problem
The question is asking for the y-coordinate of a point on the unit circle, given its x-coordinate and that the point is located in Quadrant I. To find the y-coordinate, we use the unit circle equation, x² + y² = 1.
Answer
The y-coordinate is \( \frac{\sqrt{21}}{5} \).
Answer for screen readers
The y-coordinate is ( \frac{\sqrt{21}}{5} ).
Steps to Solve
- Use the Unit Circle Equation
The equation of the unit circle is given by:
$$ x^2 + y^2 = 1 $$
Here, we know the x-coordinate, which is given as ( \frac{2}{5} ).
- Substitute the x-coordinate into the Equation
Substituting ( x ) into the equation:
$$ \left( \frac{2}{5} \right)^2 + y^2 = 1 $$
This can be simplified as:
$$ \frac{4}{25} + y^2 = 1 $$
- Isolate ( y^2 )
To find ( y^2 ), subtract ( \frac{4}{25} ) from both sides:
$$ y^2 = 1 - \frac{4}{25} $$
- Simplify the Right Side
Convert 1 into a fraction with a denominator of 25:
$$ 1 = \frac{25}{25} $$
Now, the equation becomes:
$$ y^2 = \frac{25}{25} - \frac{4}{25} = \frac{21}{25} $$
- Calculate the Value of ( y )
To find ( y ), take the square root of both sides:
$$ y = \sqrt{\frac{21}{25}} $$
This simplifies to:
$$ y = \frac{\sqrt{21}}{5} $$
Since the point is in Quadrant I, ( y ) must be positive.
The y-coordinate is ( \frac{\sqrt{21}}{5} ).
More Information
In Quadrant I, both x and y coordinates are positive. The unit circle helps relate these coordinates to trigonometric functions.
Tips
- Ignoring Quadrant Limitations: Forgetting that in Quadrant I the y-coordinate must be positive can lead to choosing the negative square root.
- Incorrectly Substituting Values: Mixing up the x-coordinate's substitution into the unit circle equation can lead to calculation errors.
AI-generated content may contain errors. Please verify critical information