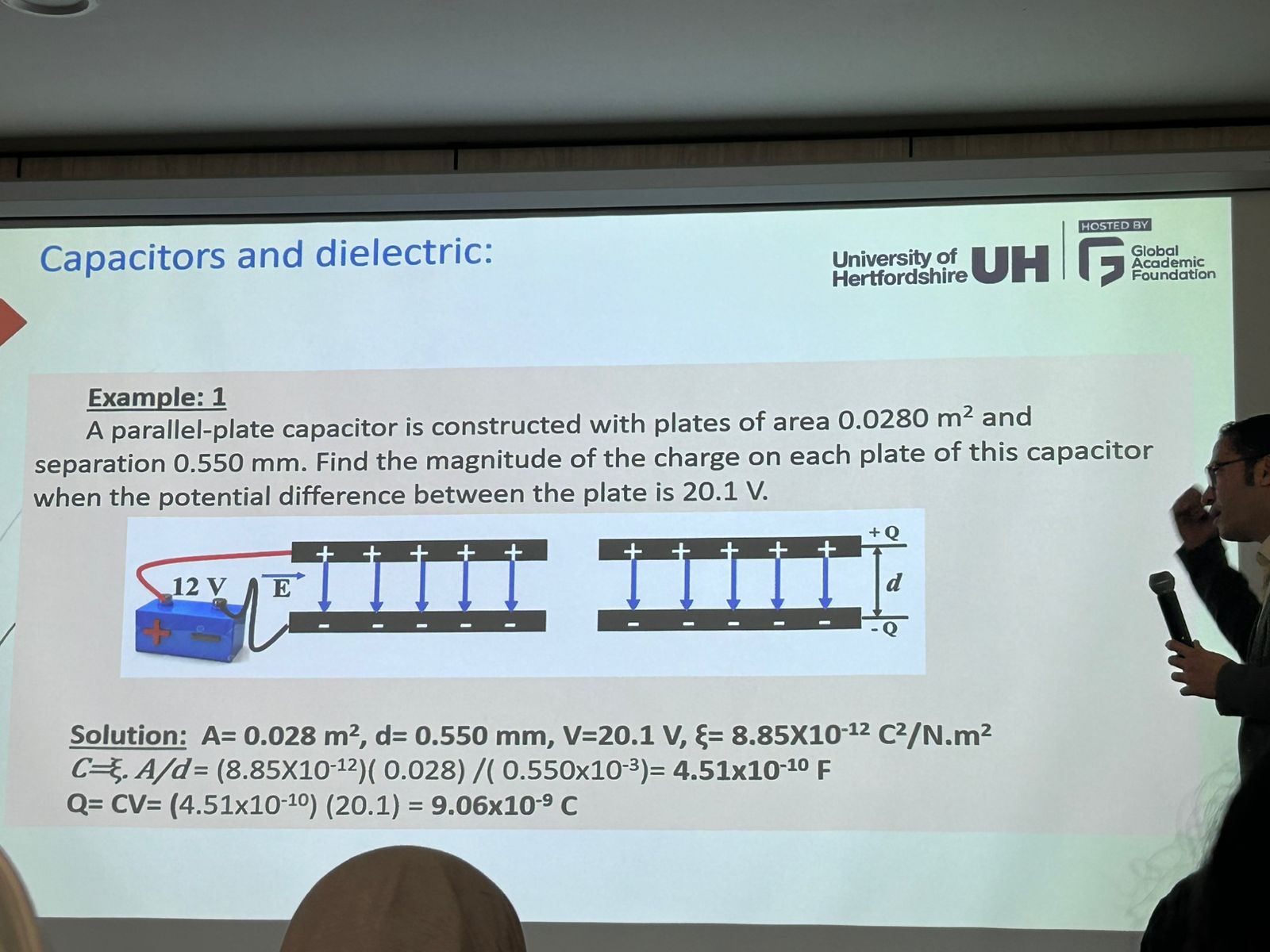
A parallel-plate capacitor is constructed with plates of area 0.0280 m² and separation 0.550 mm. Find the magnitude of the charge on each plate of this capacitor when the potential... A parallel-plate capacitor is constructed with plates of area 0.0280 m² and separation 0.550 mm. Find the magnitude of the charge on each plate of this capacitor when the potential difference between the plates is 20.1 V.
Understand the Problem
The question involves calculating the charge on each plate of a parallel-plate capacitor, given its area, separation distance, and potential difference. We'll follow the formula for capacitance and charge to find the answer.
Answer
The charge on each plate is \( Q = 9.06 \times 10^{-9} \, \text{C} \).
Answer for screen readers
The charge on each plate of the capacitor is approximately ( Q = 9.06 \times 10^{-9} , \text{C} ).
Steps to Solve
- Identify the Given Values
From the problem, we have the following information:
- Area of the plates, $A = 0.0280 , \text{m}^2$
- Separation distance, $d = 0.550 , \text{mm} = 0.550 \times 10^{-3} , \text{m}$
- Potential difference, $V = 20.1 , \text{V}$
- Permittivity of free space (for a parallel-plate capacitor), $\xi = 8.85 \times 10^{-12} , \text{C}^2/\text{N}\cdot\text{m}^2$
- Calculate the Capacitance
Use the formula for capacitance of a parallel-plate capacitor:
$$ C = \frac{\xi \cdot A}{d} $$
Substituting the values:
$$ C = \frac{(8.85 \times 10^{-12} , \text{C}^2/\text{N}\cdot\text{m}^2) \cdot (0.0280 , \text{m}^2)}{0.550 \times 10^{-3} , \text{m}} $$
- Calculate Charge on Each Plate
The charge $Q$ on each plate can be found using the formula:
$$ Q = C \cdot V $$
Substituting the capacitance value calculated in the previous step and the given potential difference:
$$ Q = C \cdot 20.1 , \text{V} $$
- Final Calculation
After obtaining $C$, substitute back to find $Q$:
Using the values found:
- Calculate capacitance.
- Substitute into the charge formula.
The charge on each plate of the capacitor is approximately ( Q = 9.06 \times 10^{-9} , \text{C} ).
More Information
In capacitors, charge is stored due to the separation of positive and negative charges between the plates. The relationship between charge, capacitance, and voltage is fundamental in understanding how capacitors work in electronic circuits.
Tips
- Unit Conversion Errors: Forgetting to convert millimeters to meters when using SI units can lead to incorrect calculations.
- Forgetting to Use Capacitance: Sometimes, students may directly calculate charge without first finding capacitance, missing an important step in the process.
AI-generated content may contain errors. Please verify critical information