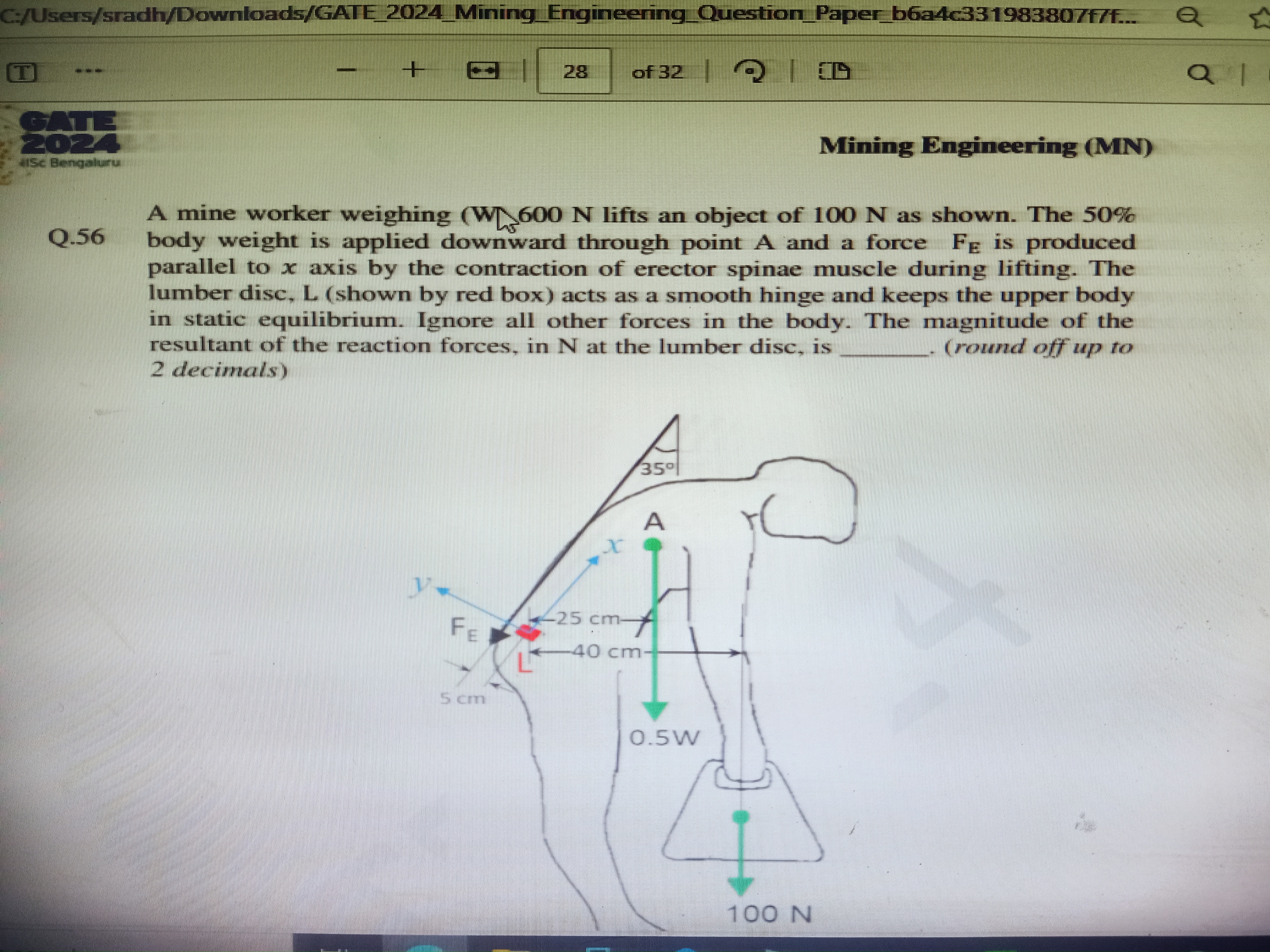
A mine worker weighing 600 N lifts an object of 100 N as shown. The 50% body weight is applied downward through point A and a force F_E is produced parallel to x axis by the contra... A mine worker weighing 600 N lifts an object of 100 N as shown. The 50% body weight is applied downward through point A and a force F_E is produced parallel to x axis by the contraction of erector spinae muscle during lifting. The lumber disc, L, acts as a smooth hinge and keeps the upper body in static equilibrium. Ignore all other forces in the body. The magnitude of the resultant of the reaction forces, in N at the lumber disc, is ______ (round off up to 2 decimals).
Understand the Problem
The question involves calculating the resultant of the reaction forces acting on a lumber disc due to a mine worker lifting an object. We will need to consider the forces and equilibrium conditions outlined in the question to find the correct solution.
Answer
The magnitude of the resultant of the reaction forces at the lumber disc is \( 400.00 \, \text{N} \).
Answer for screen readers
The magnitude of the resultant of the reaction forces at the lumber disc is ( 400.00 , \text{N} ).
Steps to Solve
- Calculate the downward force due to the worker's body weight
The weight of the worker is given as ( W = 600 , \text{N} ). Since only 50% of the body weight acts downwards at point A: $$ F_A = 0.5 \times W = 0.5 \times 600 , \text{N} = 300 , \text{N} $$
- Identify all downward forces
The downward forces acting at point A include the force due to the worker's weight and the weight of the lifted object: $$ F_{\text{total}} = F_A + 100 , \text{N} = 300 , \text{N} + 100 , \text{N} = 400 , \text{N} $$
- Set up the equilibrium conditions
To find the resulting force at the lumber disc, we need to consider both the vertical and horizontal components of forces acting on it. The forces at point L must balance the total downward force, which is ( 400 , \text{N} ).
- Recall the geometry of the forces
The force ( F_E ) is acting parallel to the x-axis, we can find its contribution to the net force. The angle with respect to the horizontal can help us resolve forces if necessary; however, we can establish equilibrium more easily here.
- Calculate the resultant reaction force at point L
The resultant reaction force ( R_L ) at point L can be defined from the balanced vertical forces: From equilibrium: $$ R_L = F_{\text{total}} = 400 , \text{N} $$
Now incorporating the horizontal aspect (if ( F_E ) is defined later with a specific relationship). But for this simplistic equilibrium: The total resultant using Pythagorean theorem if necessary: $$ R_{total} = \sqrt{(F_E)^2 + (400 , \text{N})^2} $$
Assuming ( F_E ) in the x is balanced hence: $$ R_L = 400 , \text{N} $$
- Round off the resultant if necessary
As the question states, round off the resultant to two decimal points: The resultant is ( R_L = 400.00 , \text{N} ).
The magnitude of the resultant of the reaction forces at the lumber disc is ( 400.00 , \text{N} ).
More Information
In a static equilibrium scenario, all forces acting on a body must balance. This problem involves analyzing and summing forces acting downwards and forces that uphold these to find the net reaction force.
Tips
- Forgetting to consider all forces: Make sure to include both the worker's weight and any additional weights.
- Not resolving horizontal and vertical forces properly: Ensure clarity on how forces cause moments or contribute to maintain equilibrium.
- Assuming other forces are present: The question specifies to consider only those outlined.
AI-generated content may contain errors. Please verify critical information