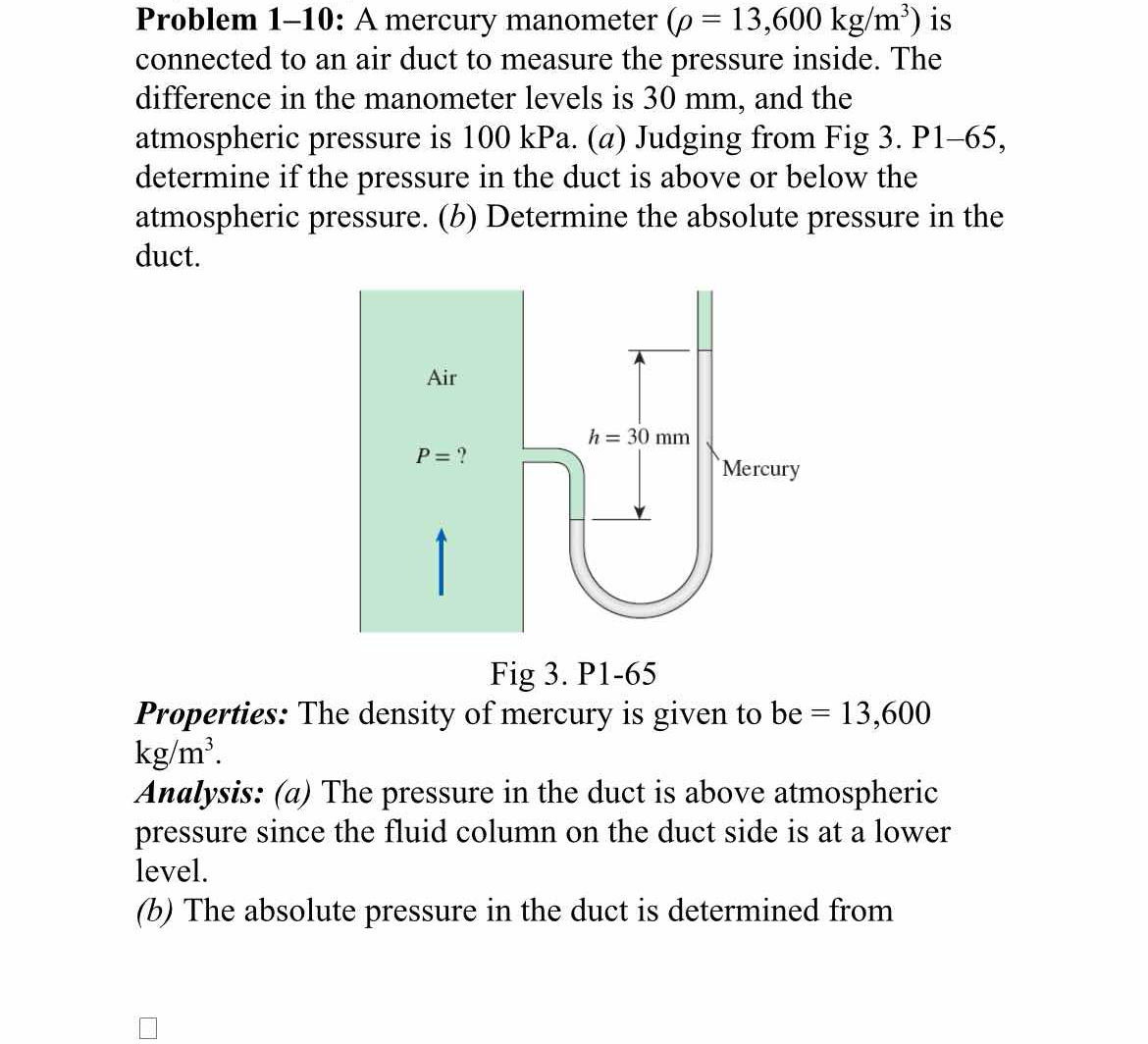
A mercury manometer (ρ = 13,600 kg/m³) is connected to an air duct to measure the pressure inside. The difference in the manometer levels is 30 mm, and the atmospheric pressure is... A mercury manometer (ρ = 13,600 kg/m³) is connected to an air duct to measure the pressure inside. The difference in the manometer levels is 30 mm, and the atmospheric pressure is 100 kPa. (a) Judging from Fig 3. P1–65, determine if the pressure in the duct is above or below atmospheric pressure. (b) Determine the absolute pressure in the duct.
Understand the Problem
The question is asking to determine the pressure in an air duct using a mercury manometer. It involves calculating both whether the duct's pressure is above or below atmospheric pressure and finding the absolute pressure in the duct based on the provided values.
Answer
$104.00 \, \text{kPa}$
Answer for screen readers
The absolute pressure in the duct is approximately $104.00 , \text{kPa}$.
Steps to Solve
-
Convert height from mm to meters
To calculate pressure, convert the height of the mercury column from millimeters to meters:
$$ h = 30 \text{ mm} = 0.030 \text{ m} $$ -
Calculate pressure difference using hydrostatic pressure formula
The pressure difference caused by the mercury column can be calculated using the formula:
$$ \Delta P = \rho g h $$
where:
- $\rho = 13,600 \text{ kg/m}^3$ (density of mercury)
- $g = 9.81 \text{ m/s}^2$ (acceleration due to gravity)
Substituting the values in:
$$ \Delta P = 13600 , \text{kg/m}^3 \times 9.81 , \text{m/s}^2 \times 0.030 , \text{m} $$
-
Calculate the pressure difference
Performing the calculations:
$$ \Delta P = 13600 \times 9.81 \times 0.030 = 4004.8 , \text{Pa} $$
Converting this to kilopascals:
$$ \Delta P \approx 4.0048 , \text{kPa} $$ -
Determine if duct pressure is above or below atmospheric pressure
Since the level of mercury in the duct side is lower, the pressure in the duct is higher than atmospheric pressure. -
Calculate absolute pressure in the duct
The absolute pressure in the duct can be determined using the formula:
$$ P_{duct} = P_{atm} + \Delta P $$
Substituting the values:
$$ P_{duct} = 100 , \text{kPa} + 4.0048 , \text{kPa} $$ -
Final calculation for absolute pressure
Calculating the absolute pressure in the duct:
$$ P_{duct} \approx 104.0048 , \text{kPa} $$
The absolute pressure in the duct is approximately $104.00 , \text{kPa}$.
More Information
The manometer helps measure pressure differences based on fluid columns. In this case, the height difference indicates a higher pressure in the duct compared to the atmospheric pressure.
Tips
- Neglecting unit conversion: Ensure all measurements are in the same unit system (e.g., converting mm to meters for height).
- Using incorrect values for gravity or density: Use standard values (e.g., $g = 9.81 , \text{m/s}^2$) for accurate calculations.
AI-generated content may contain errors. Please verify critical information