4/9 z - 3/9 z + 2 - 5/9 z - 9
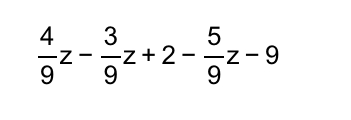
Understand the Problem
The question involves combining like terms in the expression involving variable z and simplifying it.
Answer
The simplified expression is: $$ -\frac{4}{9}z - 7 $$
Answer for screen readers
The simplified expression is:
$$ -\frac{4}{9}z - 7 $$
Steps to Solve
-
Identify the like terms
We start by identifying the terms that contain the variable $z$ and the constant terms separately.
The like terms containing $z$ are:
$$ \frac{4}{9}z, -\frac{3}{9}z, -\frac{5}{9}z $$
The constant terms are:
$$ 2, -9 $$ -
Combine the like terms for $z$
Next, we will combine the $z$ terms.
Add together the coefficients:
$$ \frac{4}{9} - \frac{3}{9} - \frac{5}{9} $$
This simplifies as follows:
- Combine the fractions:
$$ \frac{4 - 3 - 5}{9} = \frac{-4}{9} $$
-
Combine the constant terms
Now, we add the constants together:
$$ 2 - 9 = -7 $$ -
Write the final simplified expression
Now we combine the results from the previous steps:
$$ -\frac{4}{9}z - 7 $$
The simplified expression is:
$$ -\frac{4}{9}z - 7 $$
More Information
This expression indicates a linear relationship involving the variable $z$, and the constant term suggests a vertical shift in graphical representation.
Tips
- Forgetting to combine constants: Ensure every term is accounted for, both variable and constant.
- Mismanaging the fractions: Double-check the calculations when adding and subtracting fractions to avoid errors.
AI-generated content may contain errors. Please verify critical information