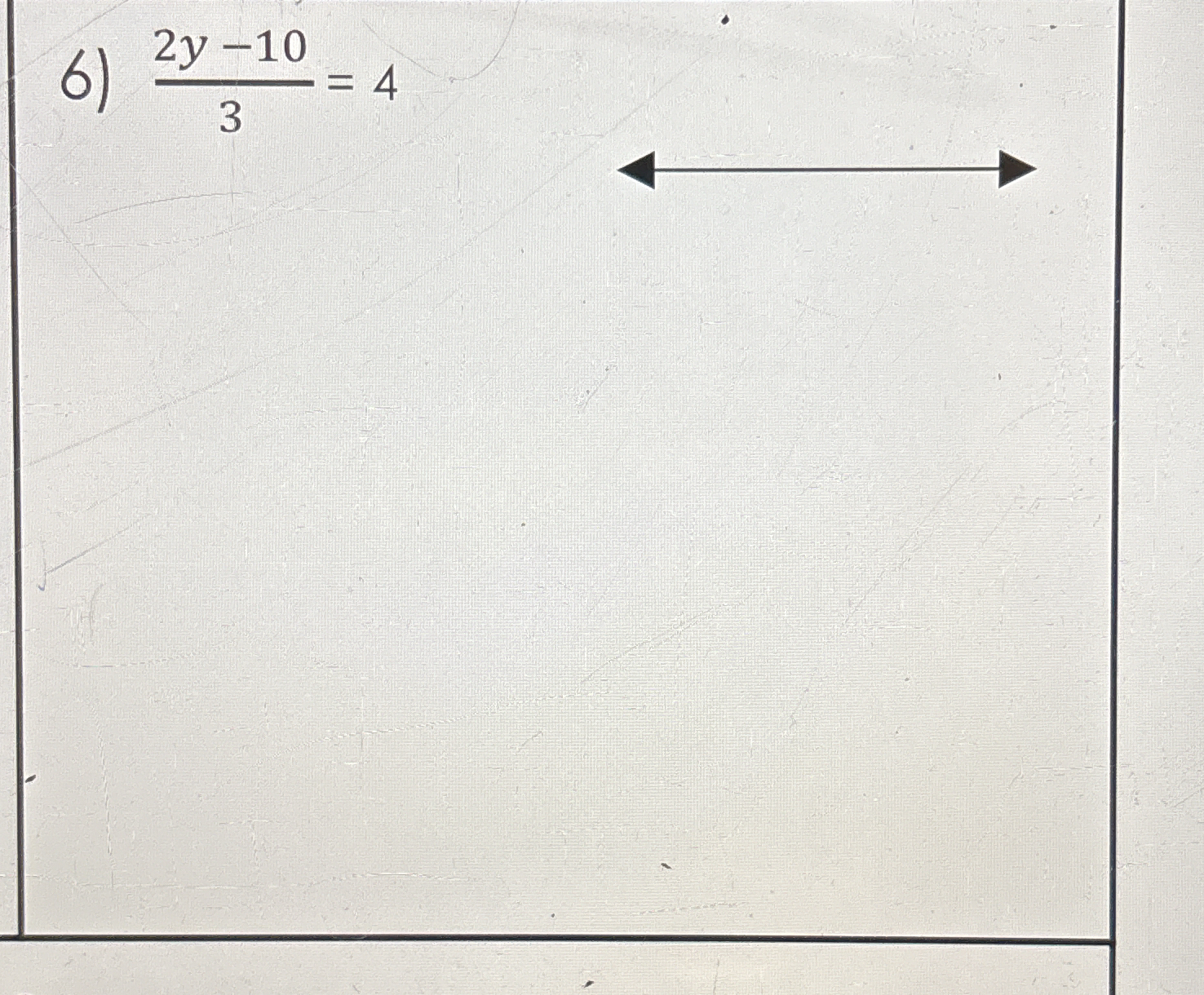
2(y - 10)/3 = 4

Understand the Problem

The question is asking to solve the equation $ \frac{2y - 10}{3} = 4 $. To solve this, we need to isolate the variable ( y ). This involves multiplying both sides by 3 to eliminate the fraction, then adding 10, and finally dividing by 2.

Answer

The solution is $ y = 11 $.

Steps to Solve

Multiply Both Sides by 3

To get rid of the fraction, multiply both sides of the equation by 3:

$$ 3 \cdot \frac{2y - 10}{3} = 3 \cdot 4 $$

This simplifies to:

$$ 2y - 10 = 12 $$

Add 10 to Both Sides

Next, isolate the term with ( y ) by adding 10 to both sides:

$$ 2y - 10 + 10 = 12 + 10 $$

This simplifies to:

$$ 2y = 22 $$

Divide by 2

Finally, divide both sides by 2 to solve for ( y ):

$$ \frac{2y}{2} = \frac{22}{2} $$

This gives us:

$$ y = 11 $$

The solution to the equation is ( y = 11 ).

More Information

This problem involves solving a linear equation with a variable. It demonstrates the basic principles of isolating a variable through the use of multiplication, addition, and division. Understanding these operations is crucial for solving similar algebraic equations.

Tips

Mistakes often made include:

Forgetting to multiply the entire equation by 3, only applying it partially.
Miscalculating the addition or division steps. To avoid these, keep track of each operation and ensure that it is applied to both sides of the equation consistently.

AI-generated content may contain errors. Please verify critical information

Thank you for voting!