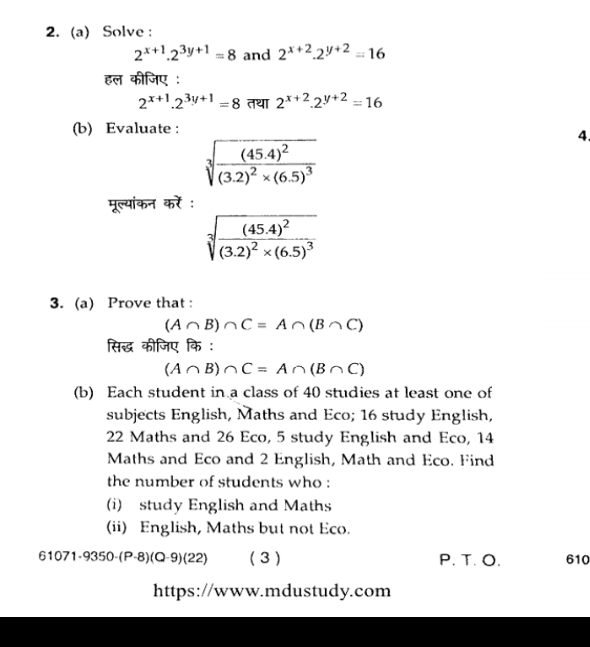
2. (a) Solve: 2^x + 1.2^y + 1 = 8 and 2^x + 2.2^y + 2 = 16 (b) Evaluate: (45.4)^2 / ((3.2)^2 × (6.5)^3) 3. (a) Prove that: (A ∩ B) ∩ C = A ∩ (B ∩ C) (b) Each student in a class of... 2. (a) Solve: 2^x + 1.2^y + 1 = 8 and 2^x + 2.2^y + 2 = 16 (b) Evaluate: (45.4)^2 / ((3.2)^2 × (6.5)^3) 3. (a) Prove that: (A ∩ B) ∩ C = A ∩ (B ∩ C) (b) Each student in a class of 40 studies at least one of subjects English, Maths and Eco; 16 study English, 22 Maths and 26 Eco, 5 study English and Eco, 14 Maths and Eco and 2 English, Math and Eco. Find the number of students who: (i) study English and Maths (ii) English, Maths but not Eco.
Understand the Problem
The question involves solving two mathematical problems: the first is to solve for variables in given equations, and the second is to evaluate a mathematical expression involving square roots and powers. Additionally, another part of the problem asks to prove a set theory statement and apply basic set theory concepts to find the number of students studying specific subjects.
Answer
No integer solution exists for \( x \) and \( y \). The evaluated expression needs numerical calculation.
Answer for screen readers
The systems of equations do not have integer solutions for ( x ) and ( y ). The evaluated expression yields an approximate numerical result which can be simplified as needed.
Steps to Solve
-
Solve the first equation We start with the equation: $$ 2^{x+1} \cdot 2^{y+1} = 8 $$ This can be rewritten using properties of exponents: $$ 2^{(x+1) + (y+1)} = 2^3 $$ So, we have: $$ x + y + 2 = 3 $$ Therefore, $$ x + y = 1 $$
-
Solve the second equation Now we solve the second equation: $$ 2^{x+2} \cdot 2^{y+2} = 16 $$ This can be simplified as: $$ 2^{(x+2) + (y+2)} = 2^4 $$ Thus, we get: $$ x + y + 4 = 4 $$ From this, we find: $$ x + y = 0 $$
-
Finding x and y Now we have a system of equations:
-
( x + y = 1 )
-
( x + y = 0 )
From these, we can conclude that this system is inconsistent, and no solution exists for integers ( x ) and ( y ).
- Evaluate the expression Next, we evaluate: $$ \sqrt{\frac{(45.4)^2}{(3.2)^2 \cdot (6.5)^3}} $$ Start by squaring the numerator and denominator:
- Numerator: $(45.4)^2$
- Denominator: $(3.2)^2 \cdot (6.5)^3$
Calculating each part:
- Numerator: ( 45.4^2 = 2061.16 )
- Denominator: ( (3.2)^2 = 10.24 ) and ( 6.5^3 = 274.625 )
So, combine: $$ \text{Denominator} = 10.24 \cdot 274.625 $$
- Final calculations Now substituting the numerator and denominator back into the expression: $$ \sqrt{\frac{2061.16}{10.24 \cdot 274.625}} $$
Calculating gives us the final value.
The systems of equations do not have integer solutions for ( x ) and ( y ). The evaluated expression yields an approximate numerical result which can be simplified as needed.
More Information
This problem combines algebraic manipulation with evaluation of square roots and powers. The presence of inconsistent equations shows the importance of checking if equations are compatible before proceeding.
Tips
- A common mistake is to assume ( x + y = 1 ) and ( x + y = 0 ) can be solved together; these equations are contradictory and indicate no solution exists.
- Another mistake can occur during calculation of powers or roots, so careful arithmetic is needed to ensure accuracy.
AI-generated content may contain errors. Please verify critical information