-2 - 2x > -8

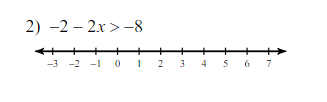
Understand the Problem

The question is asking to solve the inequality -2 - 2x > -8, which involves isolating the variable x and determining the range of values that satisfy the inequality.

Answer

$x < 3$

Steps to Solve

Isolate the constant on one side

Start by adding 2 to both sides of the inequality:

$$ -2 - 2x + 2 > -8 + 2 $$

This simplifies to:

$$ -2x > -6 $$

Isolate the variable term

Next, divide both sides by -2. Remember that dividing or multiplying by a negative number reverses the inequality:

$$ \frac{-2x}{-2} < \frac{-6}{-2} $$

This gives:

$$ x < 3 $$

Graph the solution

You can represent the solution on a number line. Shade to the left of 3, indicating that x can take any value less than 3, but not including 3 itself.

The solution to the inequality is $x < 3$.

More Information

Inequalities show a range of values for variables. Remember that the endpoint (3 in this case) is not included because of the use of "<". This means any number smaller than 3 satisfies the inequality.

Tips

Forgetting to reverse the inequality sign when dividing by a negative number. Always remember that this is a crucial step.

AI-generated content may contain errors. Please verify critical information

Thank you for voting!