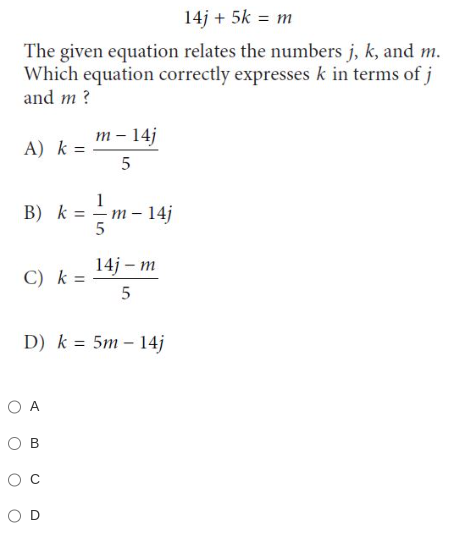
14j + 5k = m. Which equation correctly expresses k in terms of j and m?
Understand the Problem
The question is asking to solve the equation 14j + 5k = m for k, identifying which of the provided options correctly expresses k in terms of j and m.
Answer
$$ k = \frac{m - 14j}{5} $$
Answer for screen readers
The correct expression for (k) in terms of (j) and (m) is: $$ k = \frac{m - 14j}{5} $$
Steps to Solve
-
Identify the equation to solve for k We start with the equation: $$ 14j + 5k = m $$
-
Isolate the term containing k Move the term (14j) to the right side by subtracting it from both sides: $$ 5k = m - 14j $$
-
Solve for k by dividing by the coefficient of k Now, divide both sides by (5) to isolate (k): $$ k = \frac{m - 14j}{5} $$
The correct expression for (k) in terms of (j) and (m) is: $$ k = \frac{m - 14j}{5} $$
More Information
This equation expresses (k) directly in terms of (j) and (m), showing the relationship between these variables according to the original equation.
Tips
-
Forgetting to divide by the coefficient: Make sure to divide by 5 at the end to correctly isolate (k).
-
Incorrectly rearranging terms: Always check your algebra when moving terms from one side of the equation to the other.
AI-generated content may contain errors. Please verify critical information