(-1/2 + i)(2 - i)/i = ?
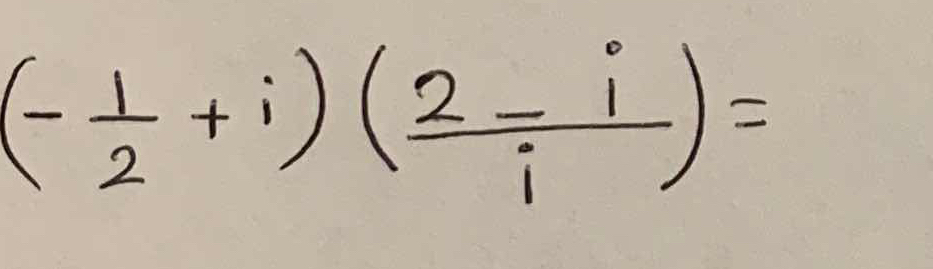
Understand the Problem
The question is asking to perform the multiplication of two complex numbers: (-1/2 + i) and (2 - i)/i. The goal is to simplify the expression and find the resulting complex number.
Answer
The result is \( \frac{5}{2} \).
Answer for screen readers
The final answer is ( \frac{5}{2} ).
Steps to Solve
-
Simplify the second complex number
We first simplify (\frac{2 - i}{i}). To do this, we can multiply the numerator and denominator by (i):
[ \frac{(2 - i) \cdot i}{i \cdot i} = \frac{2i - i^2}{-1} = \frac{2i + 1}{-1} = -2i - 1 ]
This gives us the simplified form of the second complex number.
-
Substitute and Expand
Now substitute (-2i - 1) back into the original expression:
[ (-\frac{1}{2} + i)(-2i - 1) ]
Next, we apply the distributive property (also known as the FOIL method) to multiply the complex numbers:
[ = (-\frac{1}{2})(-2i) + (-\frac{1}{2})(-1) + (i)(-2i) + (i)(-1) ]
Simplifying each term gives:
[ = i + \frac{1}{2} - 2(-1) - i = i + \frac{1}{2} + 2 - i ]
-
Combine Like Terms
Combine the real and imaginary parts:
[ = \left(\frac{1}{2} + 2\right) + (i - i) = \frac{5}{2} + 0i ]
Thus, we find our final result.
The final answer is ( \frac{5}{2} ).
More Information
The result ( \frac{5}{2} ) indicates that the multiplication of the two complex numbers yields a real number, with no imaginary component.
Tips
- Forgetting to multiply by the conjugate when simplifying fractions involving (i).
- Not properly combining like terms (real and imaginary parts) after expansion.
AI-generated content may contain errors. Please verify critical information