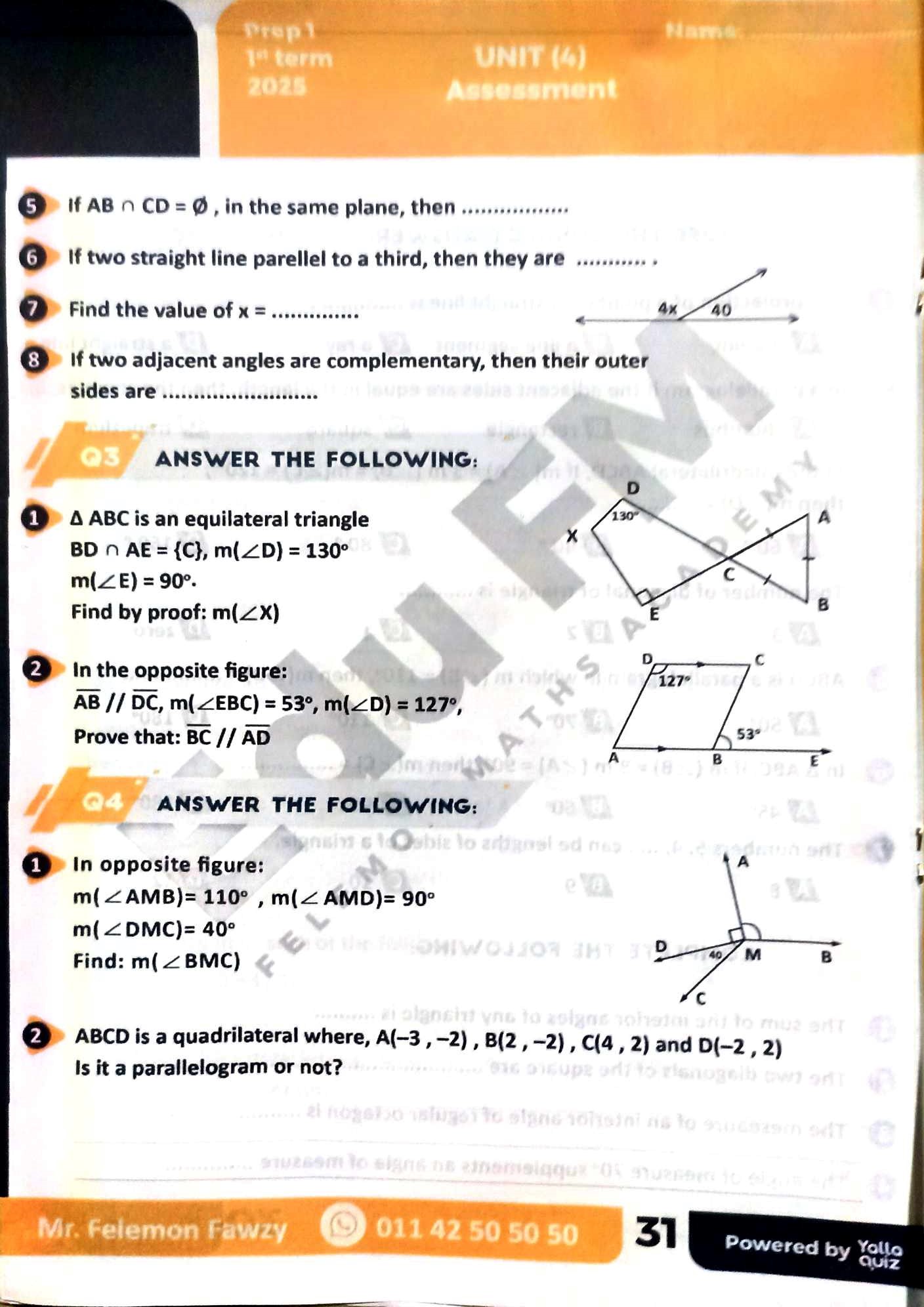
1. Triangle ABC is an equilateral triangle. Find m(<X). 2. In the opposite figure, prove that BC // AD. 3. Find m(<BMC) in the opposite figure. 4. Determine if quadrilateral ABCD i... 1. Triangle ABC is an equilateral triangle. Find m(<X). 2. In the opposite figure, prove that BC // AD. 3. Find m(<BMC) in the opposite figure. 4. Determine if quadrilateral ABCD is a parallelogram. Read more

Steps to Solve

1. Understanding Triangle ABC Triangle ABC is given as an equilateral triangle, meaning all sides and angles are equal. Therefore, each angle measures $60^\circ$.

2. Finding Angle X In triangle ABC, we know that: $$ m(\angle ABC) + m(\angle ACB) + m(\angle BAC) = 180^\circ $$ Since it is equilateral, we have: $$ 60^\circ + 60^\circ + m(\angle BAC) = 180^\circ $$ Thus, we conclude: $$ m(\angle BAC) = 60^\circ $$

Next, we are given that $m(\angle D) = 130^\circ$.

3. Using Angle Relationships From the given figure, since angles D and X are interior angles: $$ m(\angle D) + m(\angle X) = 180^\circ $$ Substituting for $m(\angle D)$: $$ 130^\circ + m(\angle X) = 180^\circ $$

4. Solving for X To find $m(\angle X)$, rearrange the equation: $$ m(\angle X) = 180^\circ - 130^\circ = 50^\circ $$

Now we proceed to the next question about parallel lines.

5. Proof of Parallel Lines (Question 2) To prove $BC \parallel AD$, we must use the Alternate Interior Angles Theorem, which states that if a transversal intersects two parallel lines, then any pair of alternate interior angles are equal.

6. Identify Relationships In the given figure:

• $m(\angle EBC) = 53^\circ$
• $m(\angle D) = 127^\circ$

By the Alternate Interior Angles Theorem: Since $AB \parallel CD$, $$ m(\angle EBC) + m(\angle D) = 180^\circ $$ This shows that the angles are supplementary, confirming that the lines are parallel.