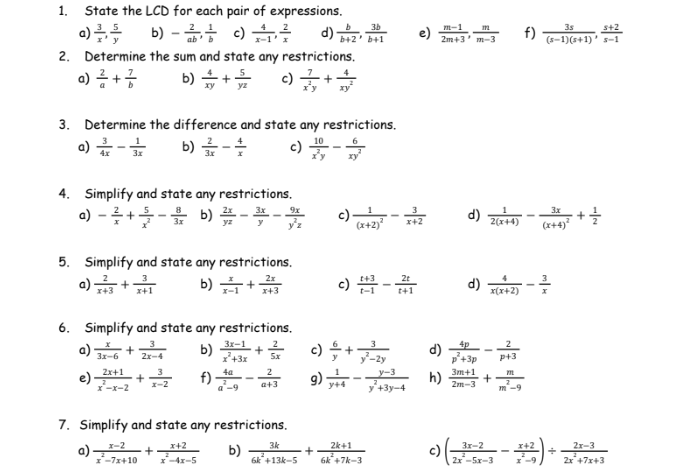
1. State the LCD for each pair of expressions. a) 3/y, 5/a b) -2/(ab), 1/b c) 4/(x-1), 2/(3b) d) 3b/(b^2/x), 3/(m-1) e) (m-1)/(2m^3+3), 3s/(s-1)(s+1) f) 2. Determine the sum and st... 1. State the LCD for each pair of expressions. a) 3/y, 5/a b) -2/(ab), 1/b c) 4/(x-1), 2/(3b) d) 3b/(b^2/x), 3/(m-1) e) (m-1)/(2m^3+3), 3s/(s-1)(s+1) f) 2. Determine the sum and state any restrictions. a) 2 + 7/4 b) 4/5 + 6/y c) 7/xy d) 3. Determine the difference and state any restrictions. a) 3/4 - 1/3 b) 2/3x - 4/x c) 10/xy - 3/y 4. Simplify and state any restrictions. a) 2/x + 5/x^2 - 8/3x b) 3x/xy - 9/x^2 c) 1/(x^2+2) d) 3/(x+4) - 1/2 e) 5. Simplify and state any restrictions. a) 2/(x^3+3) b) 3/(x-1) - 2/(x+3) c) x^3 + 1/(x-1) d) 3/(x+4) - 2/3x 6. Simplify and state any restrictions. a) -x/(x^2-7x+10) b) 3x-1/(x^2+13x+5) e) 6/(3y) - 5x/(2x^2-3y) f) 4/(y^4) + 3/(y^3+4) g) 7. Simplify and state any restrictions. a) -x^2/(x^2-3x-9) b) 3k/(6k^2+13k+5) c) (3x-2)/(x^2-5x-9) - (x^2-3)/(x^2+7x+3) Read more

Steps to Solve

1. State the LCD for Each Pair of Expressions For each pair, identify the least common denominator (LCD).

   a) For $\frac{5}{y}$ and $\frac{2}{ab/b}$, the LCD is $ab$.

   b) For $\frac{2}{x-1}$ and $\frac{1}{x}$, the LCD is $x(x-1)$.

   c) For $\frac{4}{x^2}$ and $\frac{3b}{b^2/z}$, the LCD is $x^2 z$.

   d) For $\frac{m-1}{2m^3 + 3}$ and $\frac{3}{m-3}$, first factor $2m^3 + 3$. The LCD is $2m^3 + 3(m-3)$.

   e) For $\frac{3s}{(s-1)(g+1)}$ and $\frac{s^2}{-1}$, the LCD is $-(s-1)(g+1)$.

   f) For $\frac{3s}{(s-1)(g+1)}$ and $s^2-1$, factor $s^2 - 1$ to get the LCD.

2. Determine the Sum and State Restrictions Add the given rational expressions and state any restrictions.

   a) $\frac{2}{3} + \frac{7}{4x}$

   Find a common denominator ($12x$) to combine: $$\frac{8x}{12x} + \frac{21}{12x} = \frac{8x + 21}{12x}$$

   Restrictions from $x \neq 0$.

   b) $\frac{4}{5} + \frac{5}{yz}$

   Use $\text{LCD} = 5yz$: $$\frac{4yz + 25}{5yz}$$

   Restrictions: $y \neq 0$, $z \neq 0$.

   c) $\frac{7}{xy} + \frac{4}{3}$

   LCD is $3xy$: $$\frac{21 + 4xy}{3xy}$$

   Restrictions: $x \neq 0$, $y \neq 0$.

3. Determine the Difference and State Any Restrictions Subtract the given expressions and state restrictions.

   a) $\frac{3}{4} - \frac{1}{x}$

   LCD is $4x$: $$\frac{3x - 4}{4x}$$

   Restrictions: $x \neq 0$.

   b) $\frac{2}{3x} - \frac{4}{xy}$

   LCD is $3xy$: $$\frac{2y - 12}{3xy}$$

   Restrictions: $x \neq 0$, $y \neq 0$.

   c) $\frac{10}{xy} - \frac{4}{x}$

   Use $\text{LCD} = xy$: $$\frac{10 - 4y}{xy}$$

   Restrictions: $x \neq 0$, $y \neq 0$.

4. Simplify and State Any Restrictions For each expression, simplify and note the restrictions.

   a) $\frac{2}{x^2} - \frac{8}{x^3}$

   Find common denominator ($x^3$): $$\frac{2x - 8}{x^3} = \frac{2(x-4)}{x^3}$$

   Restrictions: $x \neq 0$.

   b) $\frac{3x}{xy} - \frac{9}{y^2}$

   LCD is $y^2$: $$\frac{3x^2 - 9}{y^2} = \frac{3(x^2 - 3)}{y^2}$$

   Restrictions: $y \neq 0$.

   c) $\frac{1}{x+2} - \frac{3}{x^2 + 4}$

   Factor $x^2 + 4$: LCD is $(x+2)(x^2+4)$.

5. Continue with Remaining Problems Follow similar steps for problems involving simplifying expressions with addition, subtraction, and identifying restrictions.