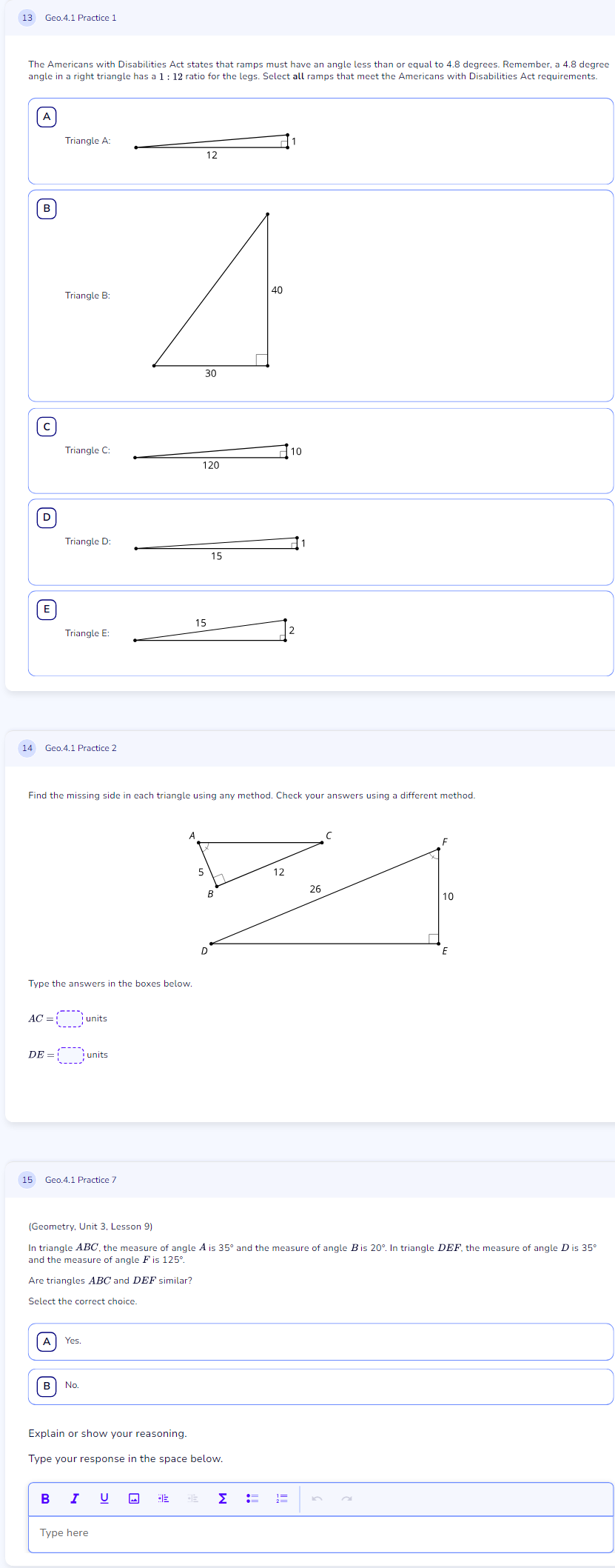
1. Select all ramps that meet the Americans with Disabilities Act requirements. 2. Find the missing side in each triangle. 3. Are triangles ABC and DEF similar, and explain your re... 1. Select all ramps that meet the Americans with Disabilities Act requirements. 2. Find the missing side in each triangle. 3. Are triangles ABC and DEF similar, and explain your reasoning. Read more

Steps to Solve

1. Check Ramp Triangle Angles Each triangle has a right angle, making it necessary to calculate the angle corresponding to the given side lengths using the tangent function. The tangent is defined as the ratio of the opposite side to the adjacent side.

2. Calculate Triangle A Angles For Triangle A, using: $$ \text{tan}(\theta) = \frac{\text{opposite}}{\text{adjacent}} = \frac{1}{12} $$ Find $\theta$: $$ \theta = \text{arctan}\left(\frac{1}{12}\right) $$

3. Calculate Triangle B Angles For Triangle B, using: $$ \text{tan}(\theta) = \frac{40}{30} $$ Calculate $\theta$: $$ \theta = \text{arctan}\left(\frac{40}{30}\right) $$

4. Calculate Triangle C Angles For Triangle C, using: $$ \text{tan}(\theta) = \frac{10}{120} $$ Calculate $\theta$: $$ \theta = \text{arctan}\left(\frac{10}{120}\right) $$

5. Calculate Triangle D Angles For Triangle D, using: $$ \text{tan}(\theta) = \frac{1}{15} $$ Calculate $\theta$: $$ \theta = \text{arctan}\left(\frac{1}{15}\right) $$

6. Calculate Triangle E Angles For Triangle E, using: $$ \text{tan}(\theta) = \frac{2}{15} $$ Calculate $\theta$: $$ \theta = \text{arctan}\left(\frac{2}{15}\right) $$

7. Determine Compliance with Angle Requirement Verify if the calculated angles are less than or equal to 4.8 degrees for compliance with the Americans with Disabilities Act.

8. Identify Missing Sides For triangles requiring missing sides (in the second problem), use the Pythagorean theorem: $$ c^2 = a^2 + b^2 $$ Calculate the missing sides based on the provided values.

9. Check Triangle Similarity Compare angle measures of triangles. If all corresponding angles are equal, then triangles are similar.