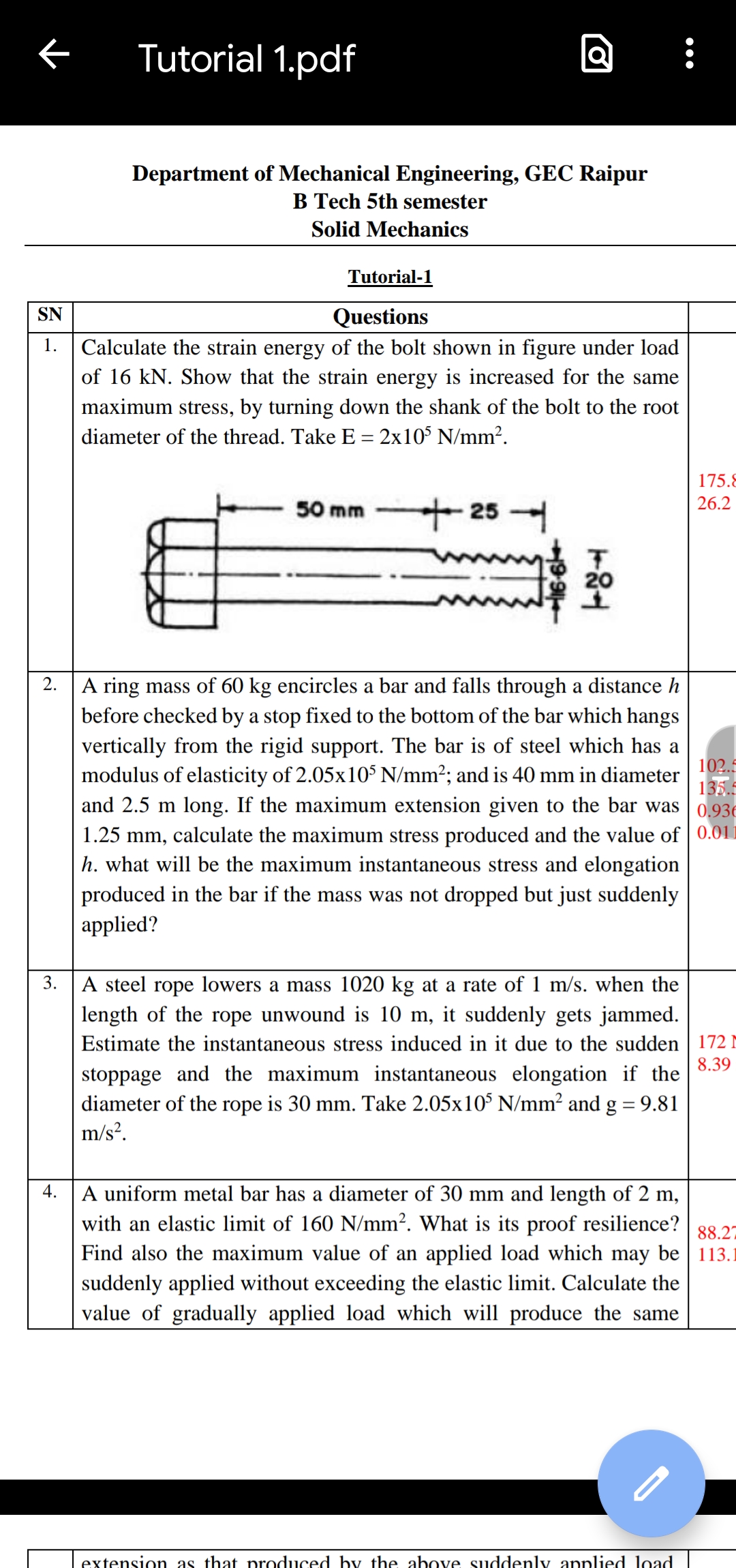
1. Calculate the strain energy of the bolt shown in the figure under load of 16 kN. Show that the strain energy is increased for the same maximum stress, by turning down the shank... 1. Calculate the strain energy of the bolt shown in the figure under load of 16 kN. Show that the strain energy is increased for the same maximum stress, by turning down the shank of the bolt to the root diameter of the thread. Take E = 2x10^5 N/mm^2. 2. A ring mass of 60 kg encircles a bar and falls through a distance h before checked by a stop fixed to the bottom of the bar which hangs vertically from the rigid support. The bar is of steel which has a modulus of elasticity of 2.05x10^5 N/mm^2; and is 40 mm in diameter and 2.5 m long. If the maximum extension given to the bar was 1.25 mm, calculate the maximum stress produced and the value of h. What will be the maximum instantaneous stress and elongation produced in the bar if the mass was not dropped but just suddenly applied? 3. A steel rope lowers a mass 1020 kg at a rate of 1 m/s. when the length of the rope unwound is 10 m, it suddenly gets jammed. Estimate the instantaneous stress induced in it due to the sudden stoppage and the maximum instantaneous elongation if the diameter of the rope is 30 mm. Take 2.05x10^5 N/mm^2 and g = 9.81 m/s^2. 4. A uniform metal bar has a diameter of 30 mm and length of 2 m, with an elastic limit of 160 N/mm^2. What is its proof resilience? Find also the maximum value of an applied load which may be suddenly applied without exceeding the elastic limit. Calculate the value of gradually applied load which will produce the same extension as that produced by the above suddenly applied load.
Understand the Problem
The questions are related to the field of solid mechanics and involve calculations regarding strain energy, maximum stress, and material properties under various load conditions.
Answer
The strain energy increases from approximately \( 0.54 \text{ J} \) to \( 1.64 \text{ J} \) when the diameter is reduced.
Answer for screen readers
The strain energy of the bolt under a load of 16 kN is approximately ( 0.54 \text{ J} ). When the diameter is turned down to the root diameter, the strain energy increases to approximately ( 1.64 \text{ J} ).
Steps to Solve
- Identify Given Information Identify the relevant data from the problem:
- Load, ( P = 16 \text{ kN} = 16,000 \text{ N} )
- Diameter of the bolt, ( d = 25 \text{ mm} = 0.025 \text{ m} )
- Modulus of elasticity, ( E = 2 \times 10^5 \text{ N/mm}^2 = 2 \times 10^9 \text{ N/m}^2 )
-
Calculate the Area of the Bolt The area ( A ) can be calculated using the formula for the cross-sectional area of a circle: $$ A = \frac{\pi d^2}{4} $$ Substituting in the diameter: $$ A = \frac{\pi (0.025)^2}{4} \approx 4.91 \times 10^{-4} \text{ m}^2 $$
-
Calculate Stress on the Bolt Stress ( \sigma ) is defined as force per unit area: $$ \sigma = \frac{P}{A} $$ Substituting in the values: $$ \sigma = \frac{16000}{4.91 \times 10^{-4}} \approx 3.25 \times 10^7 \text{ N/m}^2 $$
-
Calculate Strain Energy (U) The strain energy can be calculated using the formula: $$ U = \frac{\sigma^2 A}{2E} $$ Substituting in the calculated stress, area, and Young's modulus: $$ U = \frac{(3.25 \times 10^7)^2 \cdot (4.91 \times 10^{-4})}{2 \cdot (2 \times 10^9)} \approx 0.54 \text{ J} $$
-
Show the Effect of Reducing the Diameter When the diameter is reduced to the root diameter, recalculate:
- New diameter ( d' = 20 \text{ mm} = 0.020 \text{ m} )
- New area ( A' ): $$ A' = \frac{\pi (0.020)^2}{4} \approx 3.14 \times 10^{-4} \text{ m}^2 $$
- New stress ( \sigma' ): $$ \sigma' = \frac{16000}{3.14 \times 10^{-4}} \approx 5.09 \times 10^7 \text{ N/m}^2 $$
- New strain energy ( U' ): $$ U' = \frac{(5.09 \times 10^7)^2 \cdot (3.14 \times 10^{-4})}{2 \cdot (2 \times 10^9)} \approx 1.64 \text{ J} $$
- Comparison of Strain Energies Show that ( U' ) is greater than ( U ): $$ U' > U $$
The strain energy of the bolt under a load of 16 kN is approximately ( 0.54 \text{ J} ). When the diameter is turned down to the root diameter, the strain energy increases to approximately ( 1.64 \text{ J} ).
More Information
Strain energy is a measure of the energy stored in a material due to deformation. Reducing the diameter increases the stress experienced by the material, which in turn increases the strain energy absorbed.
Tips
- Failing to convert units properly, such as kN to N.
- Miscalculating the area of the bolt due to incorrect diameter usage.
AI-generated content may contain errors. Please verify critical information