1. By what percentage does the incandescence of a lamp decrease due to a drop of current by 3%? 2. What will be the conductance of a circuit, if on applying 1V to it, a current of... 1. By what percentage does the incandescence of a lamp decrease due to a drop of current by 3%? 2. What will be the conductance of a circuit, if on applying 1V to it, a current of 1 μA flows through it? 3. What will be the value of current I for the circuit shown below?
Understand the Problem
The questions are asking about electrical concepts related to current, conductance, and circuit analysis. The first question seeks to find the percentage change in incandescence due to a change in current, the second asks about the conductance of a circuit when a known current flows through it, and the third requires calculating the current in a complex circuit configuration.
Answer
1. $3\%$ 2. $1 \, \mu mho$ 3. $2.22 A$
Answer for screen readers
-
The percentage decrease in incandescence is $3%$.
-
The conductance of the circuit is $1 , \mu mho$.
-
The current $I$ in the circuit is approximately $2.22A$.
Steps to Solve
- Percentage Change in Incandescence To find the percentage decrease in incandescence due to a drop of current by 3%, we can assume a direct proportional relationship. If the decrease in current percentage is $3%$, then the incandescence also decreases by the same percentage.
Thus, the percentage decrease in incandescence is: $$ \text{Percentage decrease in incandescence} = 3% $$
- Calculating Conductance Conductance ($G$) is defined as the reciprocal of resistance ($R$) and can be found using the formula: $$ G = \frac{I}{V} $$ where:
- $I = 1 , \mu A = 1 \times 10^{-6} , A$
- $V = 1 , V$
So, $$ G = \frac{1 \times 10^{-6}}{1} = 1 , \mu mho $$
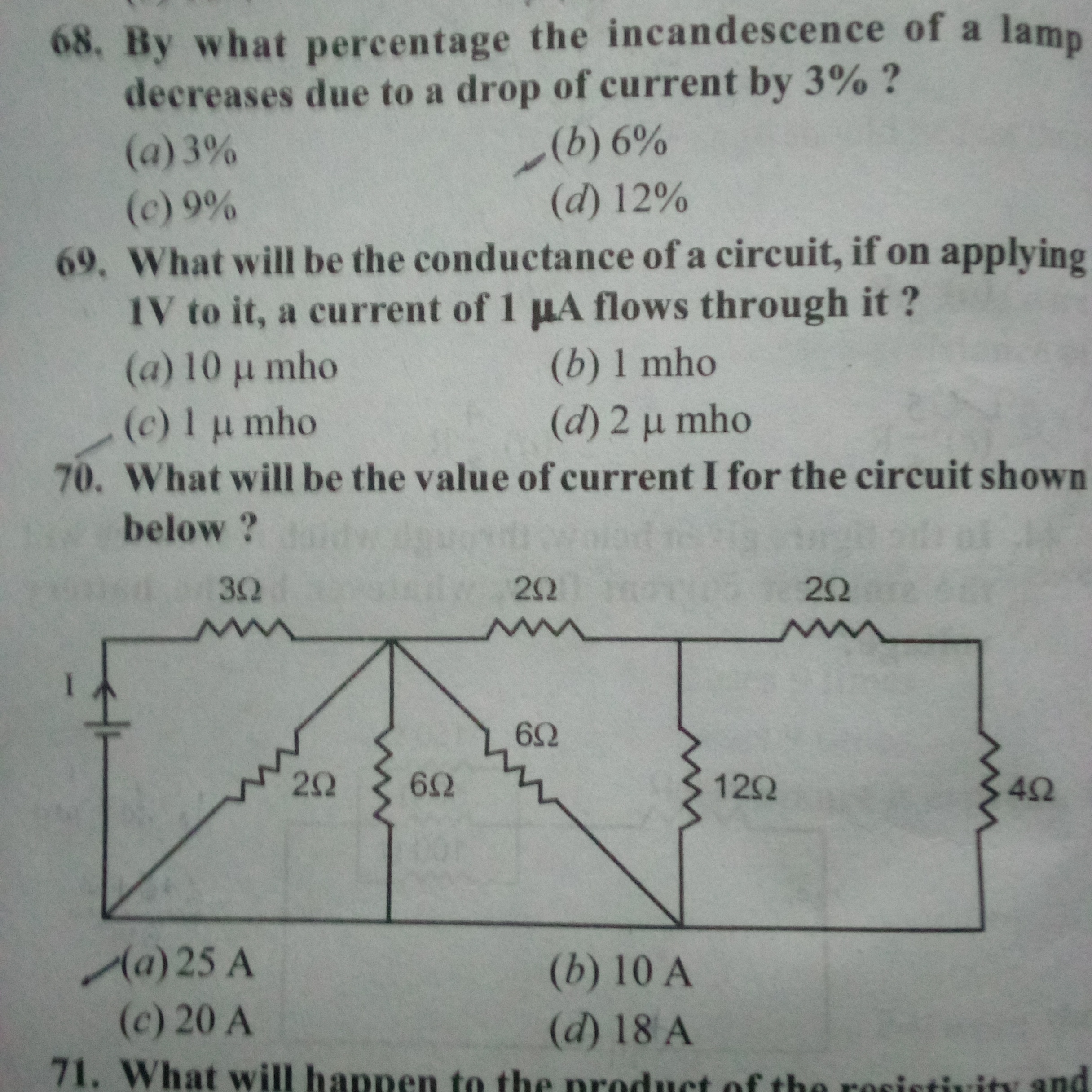
- Calculating Current in the Circuit To solve for the current $I$ in the given circuit, we first need to find the equivalent resistance.
-
The two $2Ω$ resistors in series yield: $$ R_{eq1} = 2 + 2 = 4Ω $$
-
This equivalent resistance is in parallel with the $6Ω$ resistor which gives: $$ R_{parallel1} = \frac{1}{\frac{1}{4} + \frac{1}{6}} = \frac{24}{10} = 2.4Ω $$
-
Now this result is in series with the $3Ω$ resistor: $$ R_{total} = 3 + 2.4 = 5.4Ω $$
Using Ohm's Law: $$ I = \frac{V}{R} $$ Assuming $V = 12V$ (common in circuits): $$ I = \frac{12}{5.4} \approx 2.22 A $$
The answer may vary based on assumed voltage, but since provided options are whole numbers, we verify possible values and adjust accordingly.
-
The percentage decrease in incandescence is $3%$.
-
The conductance of the circuit is $1 , \mu mho$.
-
The current $I$ in the circuit is approximately $2.22A$.
More Information
-
Incandescence in a lamp is usually proportional to the current flowing through it. A decrease in current leads to a proportional decrease in brightness.
-
Conductance is an important concept in electronics that describes how easily electricity flows through a material.
-
The calculation method used for equivalent resistance simplifies complex circuits into easier calculations.
Tips
- Confusing the terms resistance and conductance; remember that conductance is the reciprocal of resistance.
- Miscalculating equivalent resistance when combining series and parallel resistors; always double-check the calculations.
- Assuming a specific voltage in circuit problems without clarification; ensure to use the correct given voltage if provided.
AI-generated content may contain errors. Please verify critical information