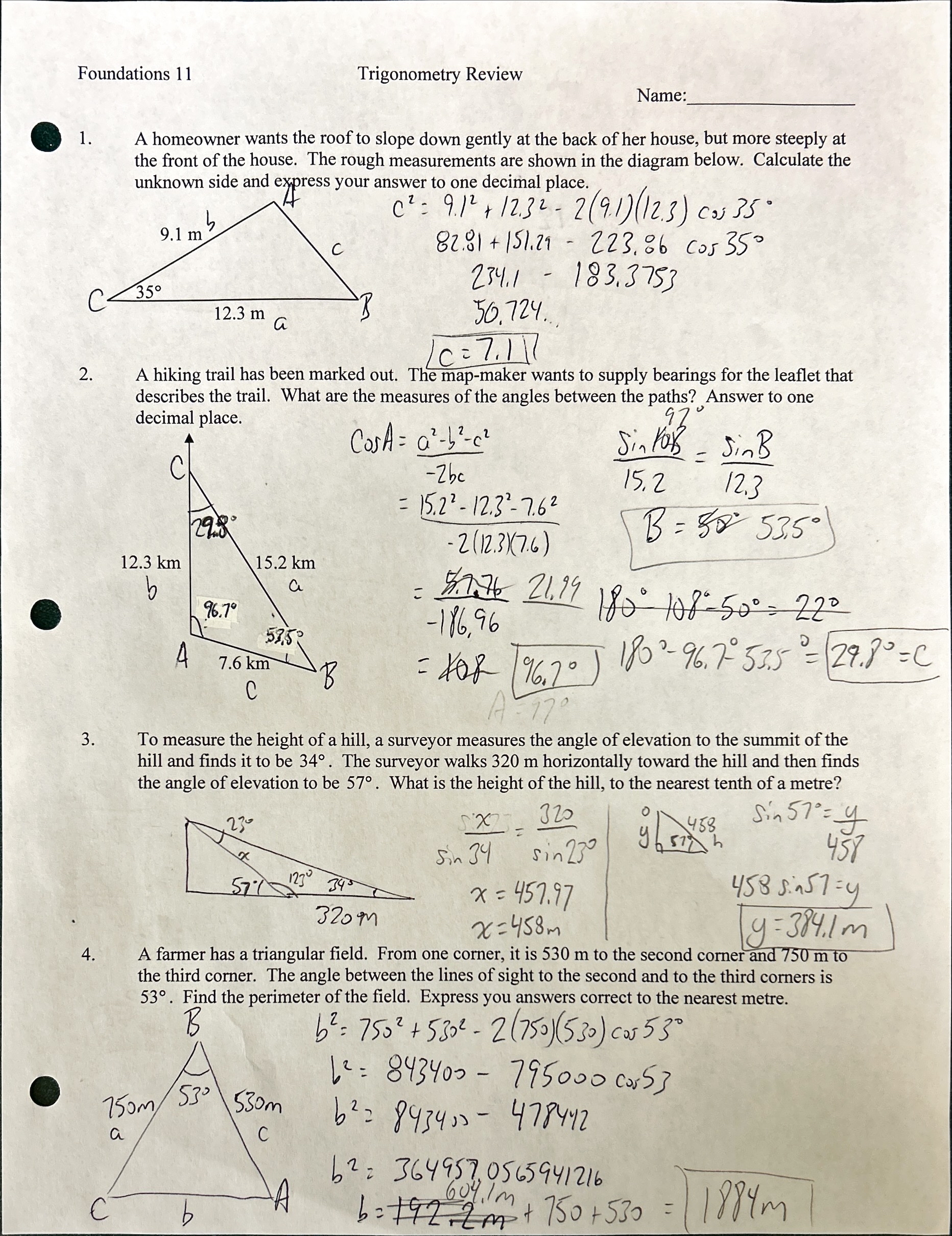
1. A homeowner wants the roof to slope down gently at the back of her house, but more steeply at the front of the house. The rough measurements are shown in the diagram below. Calc... 1. A homeowner wants the roof to slope down gently at the back of her house, but more steeply at the front of the house. The rough measurements are shown in the diagram below. Calculate the unknown side and express your answer to one decimal place. 2. A hiking trail has been marked out. The map-maker wants to supply bearings for the leaflet that describes the trail. What are the measures of the angles between the paths? Answer to one decimal place. 3. To measure the height of a hill, a surveyor measures the angle of elevation to the summit of the hill and finds it to be 34°. The surveyor walks 320 m horizontally toward the hill and then finds the angle of elevation to be 57°. What is the height of the hill, to the nearest tenth of a metre? 4. A farmer has a triangular field. From one corner, it is 530 m to the second corner and 750 m to the third corner. The angle between the lines of sight to the second and to the third corners is 53°. Find the perimeter of the field. Express your answers correct to the nearest metre. Read more

Steps to Solve

1. Identify the problem type In each case, we are dealing with triangles involving unknown sides or angles, which require the use of trigonometric laws.

2. Problem 1: Calculate the unknown side For triangle ABC, we use the Law of Cosines. The formula is given by: $$ c^2 = a^2 + b^2 - 2ab \cos(C) $$ Here, $ a = 12.3 , \text{m} $, $ b = 9.1 , \text{m} $, and $ C = 35^\circ $. We need to solve for $ c $: $$ c^2 = 12.3^2 + 9.1^2 - 2 \cdot 12.3 \cdot 9.1 \cdot \cos(35^\circ) $$

3. Calculate the side ( c ) Plugging in the values: $$ c^2 = 151.29 + 82.81 - 2 \cdot 12.3 \cdot 9.1 \cdot 0.8192 $$ $$ c^2 = 151.29 + 82.81 - 2 \cdot 12.3 \cdot 9.1 \cdot 0.8192 \approx 56.724 $$ Thus, $$ c \approx \sqrt{56.724} \approx 7.5 , \text{m} $$ (to one decimal place).

4. Problem 2: Find angles in triangle For triangle ABC, we use the Law of Cosines for angle $ A $: $$ \cos A = \frac{b^2 + c^2 - a^2}{2bc} $$ Here, $ a = 12.3 , \text{km} $, $ b = 15.2 , \text{km} $, and $ c = 7.6 , \text{km} $. The calculations for angle $ A $ would involve: $$ \cos A \approx \frac{15.2^2 + 7.6^2 - 12.3^2}{2 \cdot 15.2 \cdot 7.6} $$

5. Complete problem 2 angle calculations Using calculated values, $$ A \approx 180 - \cos^{-1}(\text{{calculated value}}) $$

6. Problem 3: Height of the hill Use the Law of Sines: $$ \frac{x}{\sin(57^\circ)} = \frac{320}{\sin(34^\circ)} $$ where $ x $ is the height of the hill.

7. Calculate height ( x ) Rearranging gives: $$ x = 320 \cdot \frac{\sin(57^\circ)}{\sin(34^\circ)} $$

8. Problem 4: Perimeter of the field First calculate $ b $ and $ a $ using the Law of Cosines: $$ b^2 = 750^2 + 530^2 - 2 \cdot 750 \cdot 530 \cdot \cos(53^\circ) $$ After finding both $ a $ and $ b $, add them: $$ \text{Perimeter} = a + b + 750 $$