∫(1 + 3x)x² dx

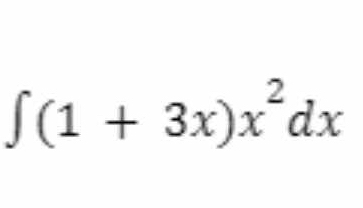
Understand the Problem

The question asks to solve the integral of the expression (1 + 3x)x^2 with respect to x. This involves applying integration techniques to find the antiderivative of the given polynomial expression.

Answer

The integral is $$ \int(1 + 3x)x^2 \, dx = \frac{x^3}{3} + \frac{3x^4}{4} + C $$

Steps to Solve

Distribute the Expression

First, distribute (x^2) across the terms in the expression ( (1 + 3x) ):

[ (1 + 3x)x^2 = x^2 + 3x^3 ]

Set Up the Integral

Now write the integral with the expanded expression:

[ \int (x^2 + 3x^3) , dx ]

Integrate Each Term

Integrate each term separately. For ( \int x^n , dx ), the formula is:

[ \int x^n , dx = \frac{x^{n+1}}{n+1} + C ]

Applying this:

For ( x^2 ):

[ \int x^2 , dx = \frac{x^{2+1}}{2+1} = \frac{x^3}{3} ]

For ( 3x^3 ):

[ \int 3x^3 , dx = 3 \cdot \frac{x^{3+1}}{3+1} = \frac{3x^4}{4} ]

Combine the Results

Now combine the results of the integrals:

[ \int (x^2 + 3x^3) , dx = \frac{x^3}{3} + \frac{3x^4}{4} + C ]

The integral is

$$ \int(1 + 3x)x^2 , dx = \frac{x^3}{3} + \frac{3x^4}{4} + C $$

More Information

This integral represents the area under the curve of the polynomial ( (1 + 3x)x^2 ) with respect to ( x ). The constant ( C ) is included as it represents any constant value that could be added to the antiderivative since the derivative of a constant is zero.

Tips

Forgetting to include the constant ( C ) in the final answer is a common mistake.
Not applying the power rule correctly when integrating can lead to errors in the final expression.

Thank you for voting!