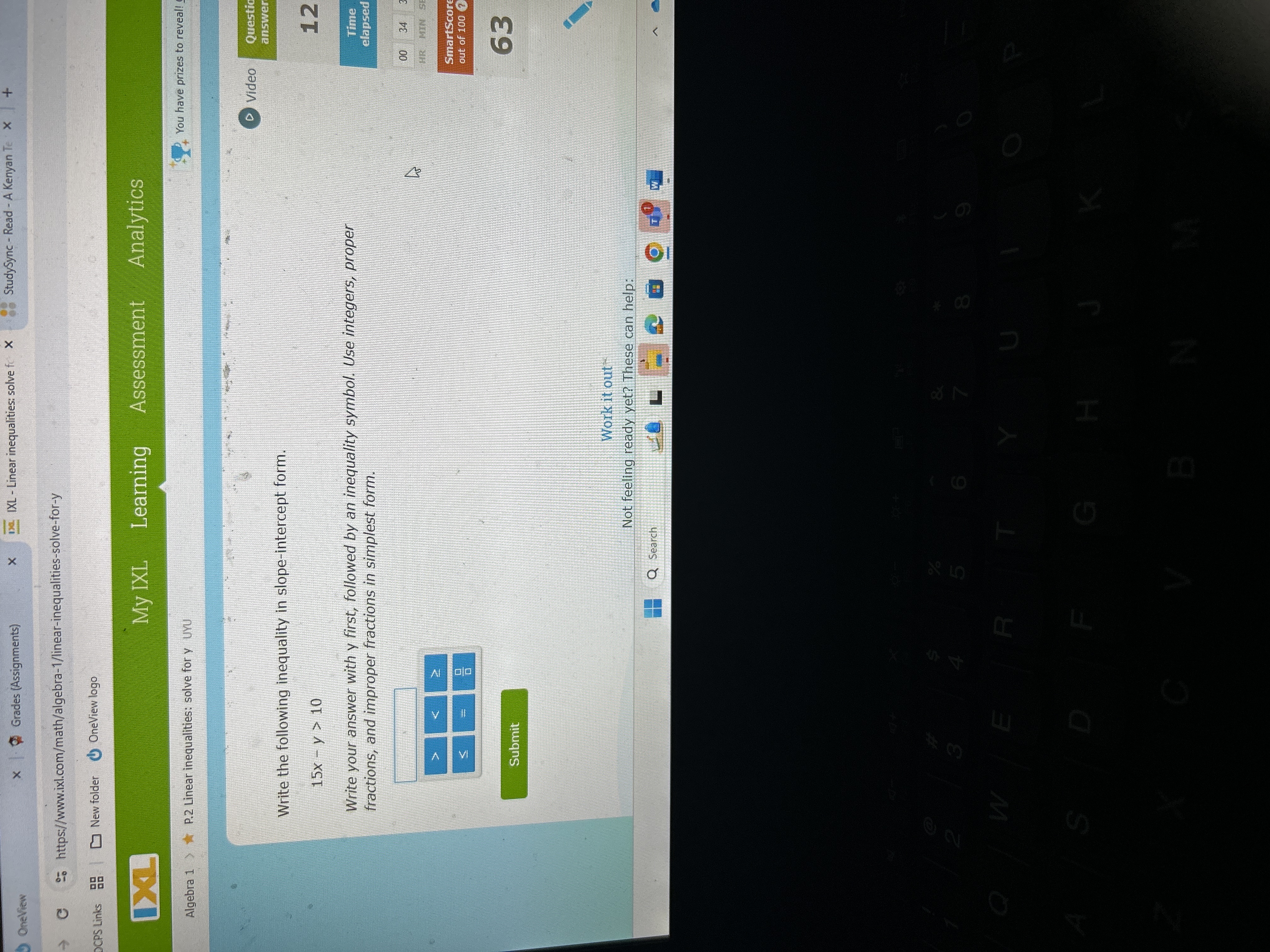
Write the following inequality in slope-intercept form: 15x - y > 10. Write your answer with y first, followed by an inequality symbol. Use integers, proper fractions, and improper... Write the following inequality in slope-intercept form: 15x - y > 10. Write your answer with y first, followed by an inequality symbol. Use integers, proper fractions, and improper fractions in simplest form.
Understand the Problem
The question is asking to rewrite the given inequality (15x - y > 10) into slope-intercept form, which is typically expressed as y = mx + b. This involves isolating y and adjusting the equation to fit the format while selecting the appropriate inequality symbol.
Answer
$$ y < 15x - 10 $$
Answer for screen readers
$$ y < 15x - 10 $$
Steps to Solve
-
Rewrite the Inequality We start with the inequality: $$ 15x - y > 10 $$
-
Isolate y To isolate $y$, we move $15x$ to the right side: $$ -y > 10 - 15x $$
-
Multiply by -1 By multiplying the entire inequality by -1, we need to reverse the inequality sign: $$ y < 15x - 10 $$
-
Final Slope-Intercept Form Now the inequality is in slope-intercept form: $$ y < 15x - 10 $$
$$ y < 15x - 10 $$
More Information
The slope of the line is 15 and the y-intercept is -10. This means for every one unit increase in $x$, $y$ increases by 15 units. The inequality indicates that $y$ is less than the value of $15x - 10$.
Tips
- Failing to reverse the inequality sign when multiplying or dividing by a negative number.
- Not isolating $y$ correctly or misplacing terms during rearrangement.
AI-generated content may contain errors. Please verify critical information