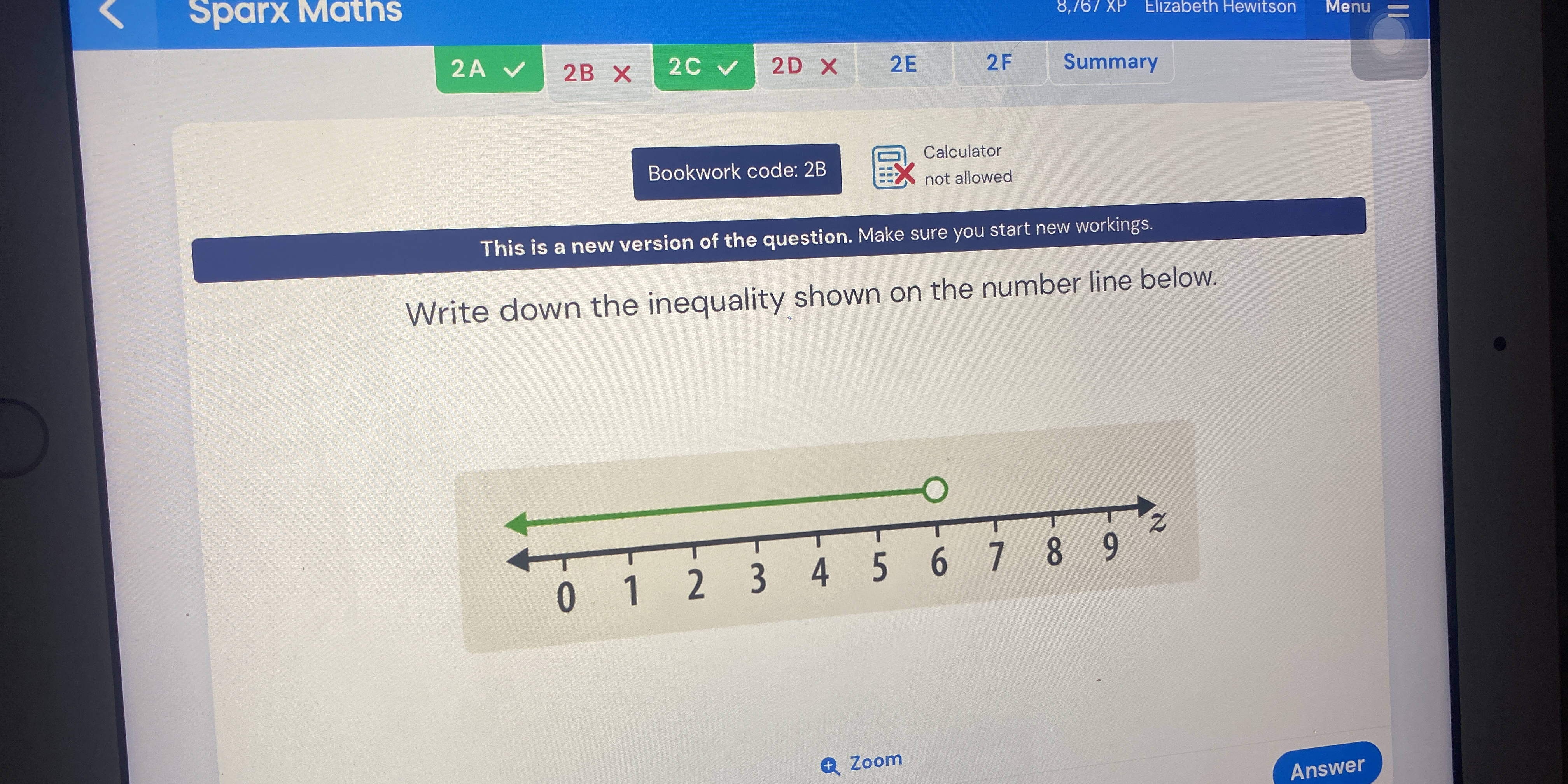
Write down the inequality shown on the number line below.
Understand the Problem
The question is instructing us to represent a specific inequality on a number line, which suggests that we need to interpret the number line and determine the appropriate inequality notation.
Answer
The inequality is \( 0 < x \leq 8 \).
Answer for screen readers
The inequality represented on the number line is: $$ 0 < x \leq 8 $$
Steps to Solve
- Identify the Key Points on the Number Line
Look at the number line and determine the points marked. In this case, it appears that the number line has endpoints at 0 and 8, and it is clear which direction is indicated.
- Determine the Type of Endpoint
Since there is an open circle at 0, it indicates that 0 is not included in the solution. This can be represented mathematically as ( x > 0 ).
- Find the End of the Range
The line then extends to the right towards 8. As there is a filled endpoint at 8, this means that 8 is included in the solution, which is represented as ( x \leq 8 ).
- Combine the Inequalities
Combine the inequalities from steps 2 and 3. The final inequality will be written as: $$ 0 < x \leq 8 $$
The inequality represented on the number line is: $$ 0 < x \leq 8 $$
More Information
This inequality indicates that ( x ) can take any value greater than 0 and up to and including 8. Inequalities are commonly used in algebra to describe ranges of values.
Tips
- Confusing open and closed intervals: Ensure that open circles indicate exclusion from the solution and closed circles indicate inclusion.
- Incorrect direction of the inequality: When the arrow points to the right, use "greater than" inequalities.
AI-generated content may contain errors. Please verify critical information