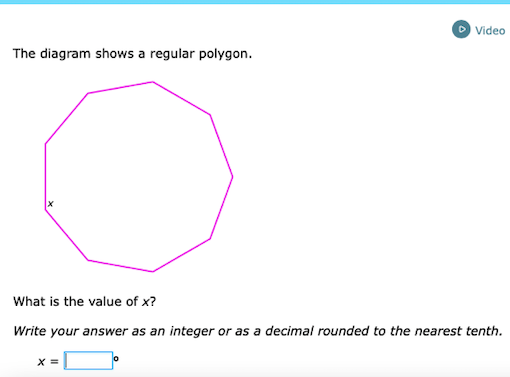
What is the value of x in a regular octagon? Write your answer as an integer or as a decimal rounded to the nearest tenth.
Understand the Problem
The question is asking for the measure of the angle 'x' in a regular polygon, specifically an octagon. To solve this, we need to determine the interior angle of a regular octagon and provide the answer rounded to the nearest tenth.
Answer
The measure of angle \( x \) is \( 135^\circ \).
Answer for screen readers
The value of ( x ) is ( 135^\circ ).
Steps to Solve
-
Determine the number of sides of the polygon
An octagon has 8 sides. -
Use the formula for the interior angle of a regular polygon
The formula for finding the measure of an interior angle ( A ) of a regular polygon is given by:
$$ A = \frac{(n - 2) \times 180}{n} $$
where ( n ) is the number of sides. For an octagon, ( n = 8 ). -
Substitute the number of sides into the formula
Plugging in the value of ( n ):
$$ A = \frac{(8 - 2) \times 180}{8} $$ -
Calculate the interior angle
Simplifying the equation:
$$ A = \frac{6 \times 180}{8} = \frac{1080}{8} = 135 $$
So, the measure of angle ( x ) is ( 135^\circ ). -
Round the answer to the nearest tenth (if necessary)
In this case, ( 135 ) is already an integer and doesn't require rounding.
The value of ( x ) is ( 135^\circ ).
More Information
An octagon is a polygon with eight sides, and each interior angle in a regular octagon measures ( 135 ) degrees. This is because regular polygons are symmetrical and share equal angles.
Tips
- Confusing the formula for interior angles with the exterior angle formula.
- Miscalculating the number of sides for the polygon, leading to incorrect interior angle calculations.
AI-generated content may contain errors. Please verify critical information