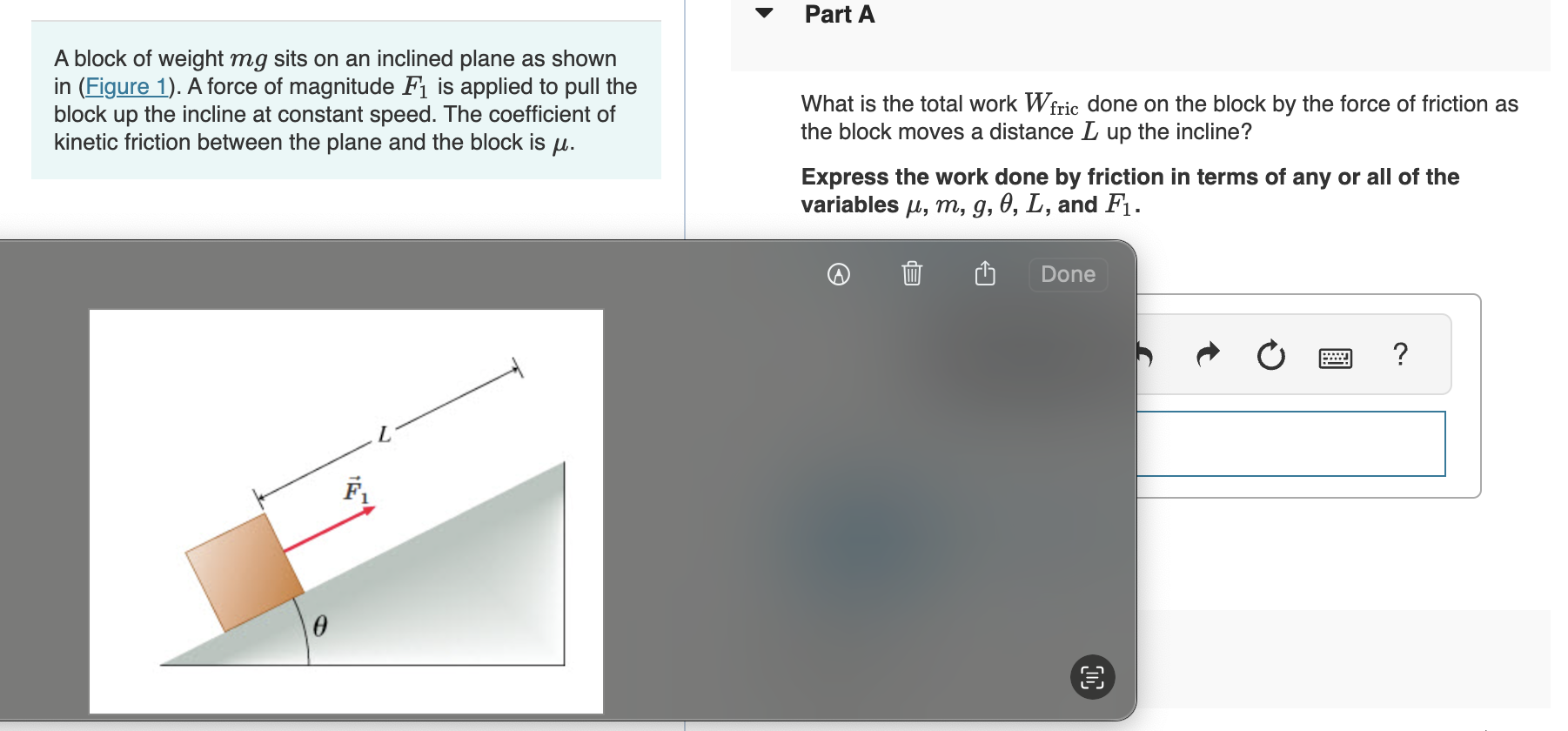
What is the total work done on the block by the force of friction as the block moves a distance L up the incline? Express the work done by friction in terms of any or all of the va... What is the total work done on the block by the force of friction as the block moves a distance L up the incline? Express the work done by friction in terms of any or all of the variables μ, m, g, θ, L, and F1.
Understand the Problem
The question is asking for the total work done on a block by the force of friction as it moves up an incline. The work needs to be expressed in terms of variables such as the coefficient of friction, mass, gravitational acceleration, distance traveled, and the applied force.
Answer
The work done by friction is given by $W_{\text{fric}} = -\mu mg \cos(\theta) L$.
Answer for screen readers
The total work done by the friction force on the block is given by: $$ W_{\text{fric}} = -\mu mg \cos(\theta) L $$
Steps to Solve
- Identify the forces acting on the block
The forces acting on the block include its weight, which can be broken down into components along the incline and perpendicular to the incline, the applied force $F_1$, and the frictional force $f_k$. The weight can be expressed as: $$ W = mg $$
The component of the weight acting down the incline is: $$ W_{\parallel} = mg \sin(\theta) $$
- Determine the normal force
The normal force $N$ acting on the block can be calculated using the component of the weight that acts perpendicular to the incline: $$ N = mg \cos(\theta) $$
- Calculate the kinetic friction force
The force of kinetic friction $f_k$ can be calculated using the coefficient of friction $\mu$: $$ f_k = \mu N = \mu (mg \cos(\theta)) $$
- Calculate the total work done by friction
The total work done by the friction force as the block moves a distance $L$ up the incline is given by: $$ W_{\text{fric}} = - f_k L $$ Substituting $f_k$ gives: $$ W_{\text{fric}} = - (\mu mg \cos(\theta)) L $$
- Final expression for work done by friction
The final expression for the work done by the friction force as the block moves distance $L$ is: $$ W_{\text{fric}} = -\mu mg \cos(\theta) L $$
The total work done by the friction force on the block is given by: $$ W_{\text{fric}} = -\mu mg \cos(\theta) L $$
More Information
The work done against friction is negative because friction acts in the opposite direction to the movement of the block. This indicates that friction dissipates energy as the block moves up the incline.
Tips
- Forgetting to consider the direction of the friction force can lead to incorrect sign in the work calculation. Always remember that friction opposes motion.
- Neglecting to account for both components of weight when calculating the normal force can result in an inaccurate friction force.
AI-generated content may contain errors. Please verify critical information