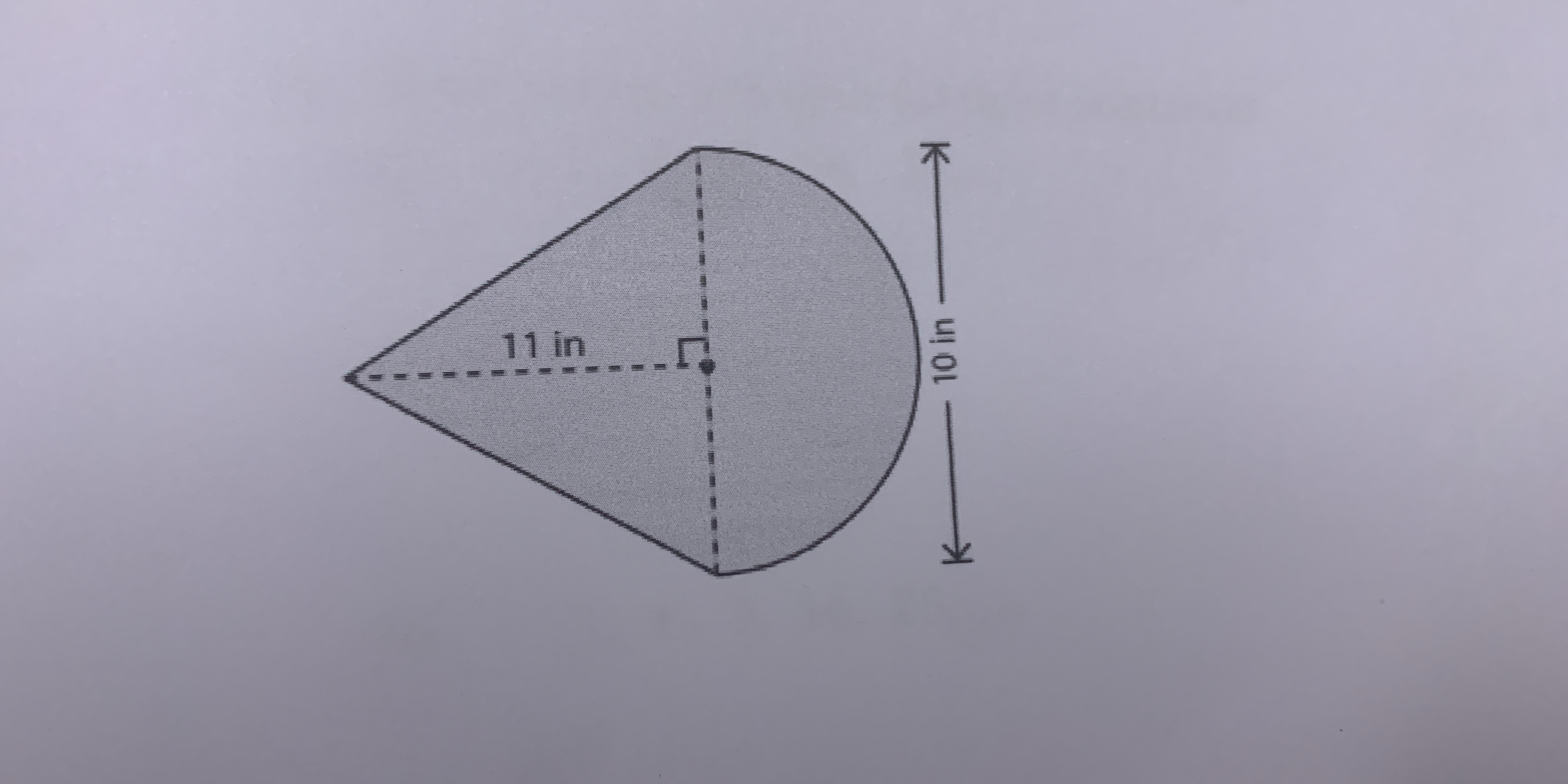
What is the surface area of the pictured shape with a height of 10 in and a base radius of 11 in?
Understand the Problem
The question involves a geometric shape, likely a composite shape consisting of a cone and a circular base. It is providing dimensions of the shape and seems to ask for a calculation related to its area, volume, or surface area. The task will involve applying formulas related to geometry.
Answer
The volume of the cone is approximately $70\pi$ cubic inches, or about 219.8 cubic inches.
Answer for screen readers
The volume of the cone is approximately $70\pi$ cubic inches, or about 219.8 cubic inches.
Steps to Solve
-
Identify the dimensions and shape The given shape is a cone with a height ($h$) of 10 inches and a slant height ($l$) of 11 inches. We need to find the radius ($r$) of the base of the cone.
-
Use the Pythagorean theorem to find the radius Since the radius, height, and slant height form a right triangle, we can apply the Pythagorean theorem: $$ l^2 = r^2 + h^2 $$ Substituting the given values: $$ 11^2 = r^2 + 10^2 $$ This simplifies to: $$ 121 = r^2 + 100 $$
-
Solve for the radius Rearranging the equation gives: $$ r^2 = 121 - 100 $$ $$ r^2 = 21 $$ Taking the square root of both sides to find $r$: $$ r = \sqrt{21} $$
-
Calculate the volume of the cone The volume ($V$) of a cone is calculated using the formula: $$ V = \frac{1}{3} \pi r^2 h $$ Substituting the known values: $$ V = \frac{1}{3} \pi (\sqrt{21})^2 (10) $$ $$ V = \frac{1}{3} \pi (21) (10) $$ This simplifies to: $$ V = 70 \pi $$
-
Approximate the volume Using $\pi \approx 3.14$ for approximation: $$ V \approx 70 \times 3.14 \approx 219.8 $$
The volume of the cone is approximately $70\pi$ cubic inches, or about 219.8 cubic inches.
More Information
The volume formula for a cone is derived from the geometry of three-dimensional shapes. The cone is part of a larger topic concerning solid figures and their properties. An interesting fact is that the formula for the volume of a cone can be understood as one-third of the product of the base area and the height, emphasizing how cones are often represented as "pyramids" with a circular base.
Tips
- Confusing slant height and height: Ensure that you are using the correct dimensions; slant height is not equivalent to the vertical height of the cone.
- Not applying the Pythagorean theorem correctly: It’s essential to set up the equation properly to solve for the radius.
AI-generated content may contain errors. Please verify critical information