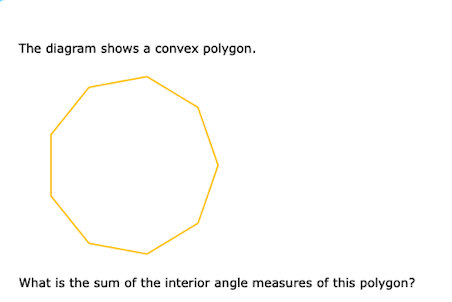
What is the sum of the interior angle measures of this polygon?
Understand the Problem
The question is asking for the sum of the interior angles of a convex polygon, which can be calculated using the formula (n - 2) × 180°, where n is the number of sides of the polygon.
Answer
The sum of the interior angle measures of this polygon is $1080^\circ$.
Answer for screen readers
The sum of the interior angle measures of this polygon is $1080^\circ$.
Steps to Solve
-
Identify the number of sides The given polygon is an octagon, which has 8 sides. Thus, we have $n = 8$.
-
Use the formula for the sum of interior angles To find the sum of the interior angles of a polygon, use the formula: $$(n - 2) \times 180^\circ$$
-
Substitute the value of n into the formula Substituting $n = 8$ into the formula gives: $$(8 - 2) \times 180^\circ$$
-
Calculate the result Now we perform the calculations: $$(6) \times 180^\circ = 1080^\circ$$
The sum of the interior angle measures of this polygon is $1080^\circ$.
More Information
The sum of the interior angles formula is a fundamental concept in geometry. For any polygon, the sum increases as the number of sides increases, with a triangle (3 sides) having $180^\circ$ and a quadrilateral (4 sides) having $360^\circ$.
Tips
- Confusing the number of sides: Always ensure you count the sides correctly; mistakes often arise from misidentifying the shape.
- Misapplying the formula: Double-check the substitution and the arithmetic operations performed after applying the formula.
AI-generated content may contain errors. Please verify critical information