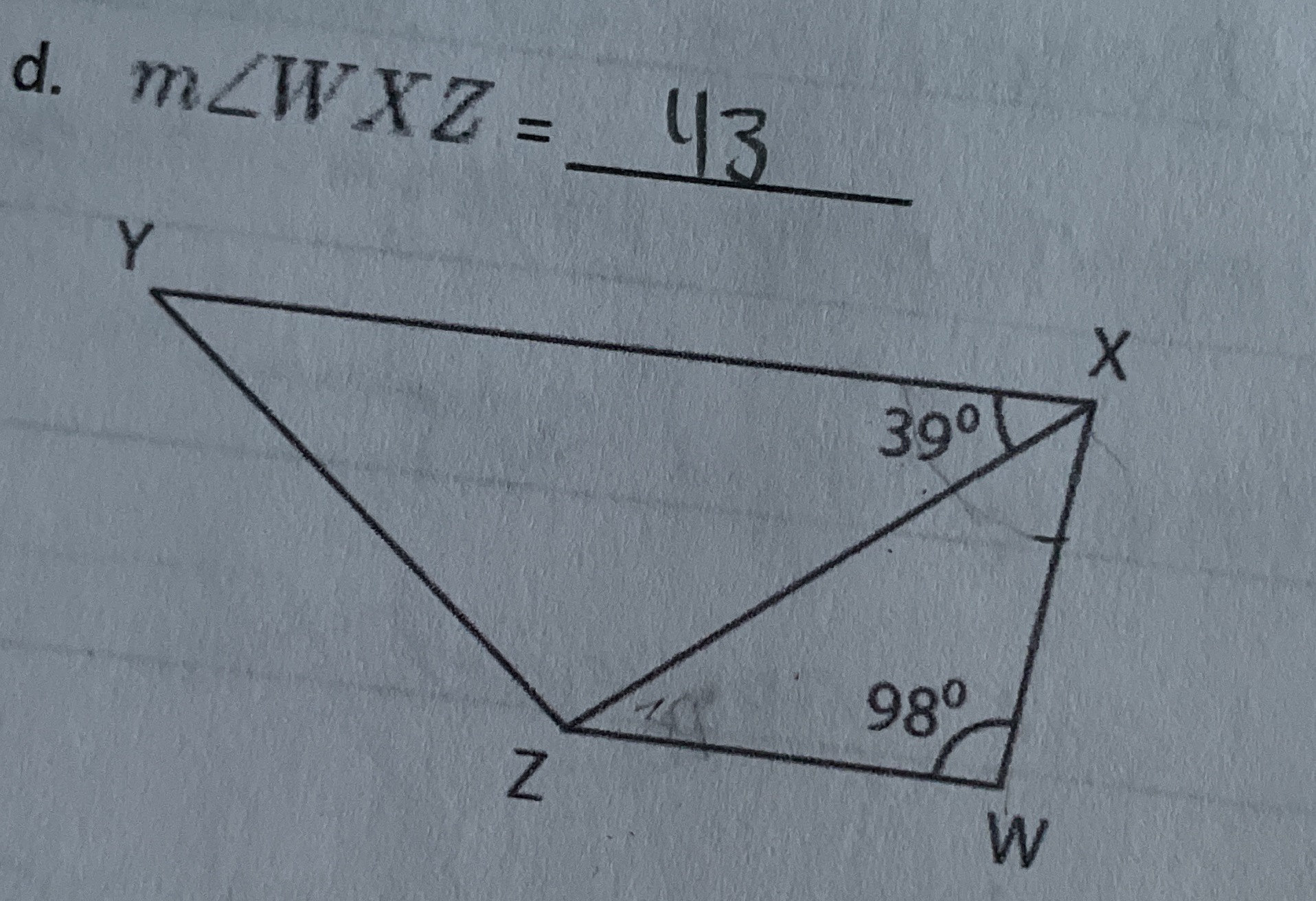
What is the measure of angle WXZ?
Understand the Problem
The question is asking for the measure of angle WXZ in the given triangle. To find this, we can use the properties of angles in a triangle, specifically that the sum of the interior angles equals 180 degrees.
Answer
The measure of angle $WXZ$ is $43^\circ$.
Answer for screen readers
The measure of angle $WXZ$ is $43^\circ$.
Steps to Solve
- Understand the triangle angle sum property
The sum of the interior angles in a triangle is always $180^\circ$. In triangle $WXY$, we can set up the equation:
$$ m∠WXY + m∠XYW + m∠WXZ = 180^\circ $$
- Identify known angles
From the diagram, we know:
- $m∠WXY = 39^\circ$
- $m∠XYW = 98^\circ$
- Set up the equation with known values
Substituting the known angles into the equation gives:
$$ 39^\circ + 98^\circ + m∠WXZ = 180^\circ $$
- Combine the known angles
Now, add $39^\circ$ and $98^\circ$:
$$ 39^\circ + 98^\circ = 137^\circ $$
- Solve for the unknown angle
Now we can find $m∠WXZ$ by rearranging the equation:
$$ m∠WXZ = 180^\circ - 137^\circ $$
- Calculate the measure
Perform the calculation:
$$ m∠WXZ = 43^\circ $$
The measure of angle $WXZ$ is $43^\circ$.
More Information
In any triangle, the sum of the angles must equal $180^\circ$. This fundamental property of triangles is essential for solving various geometry problems.
Tips
- Forgetting to use the angle sum property correctly.
- Adding the angles incorrectly.
AI-generated content may contain errors. Please verify critical information