What is the magnitude of force Q needed to achieve this?
Understand the Problem
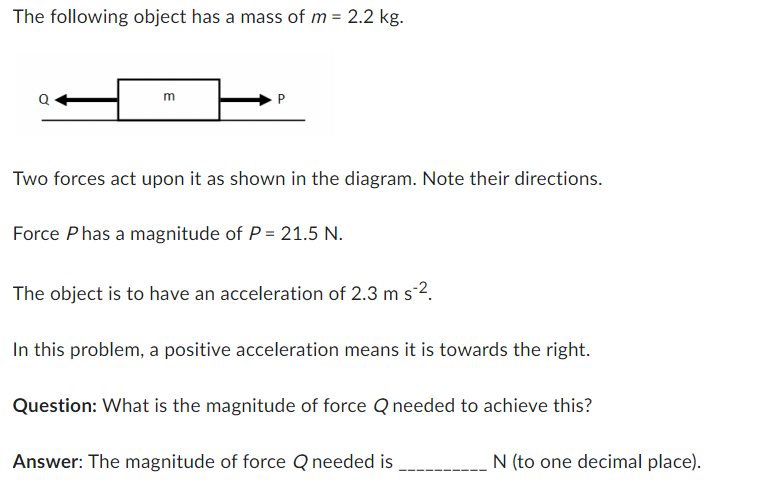
The question is asking for the magnitude of force Q needed to produce a specific acceleration of an object given its mass and the opposing force P. We will use Newton's second law, F = ma, to solve for force Q.
Answer
The magnitude of force $Q$ needed is $26.6 \, \text{N}$.
Answer for screen readers
The magnitude of force $Q$ needed is $26.6 , \text{N}$.
Steps to Solve
- Identify the known quantities
The mass of the object is given as $m = 2.2 , \text{kg}$.
The opposing force is $P = 21.5 , \text{N}$ (acting to the left).
The desired acceleration is $a = 2.3 , \text{m/s}^2$ (to the right).
- Write down Newton's second law
According to Newton’s second law, the net force $F_{net}$ acting on an object is equal to the product of the mass $m$ and its acceleration $a$:
$$ F_{net} = ma $$
- Calculate the net force
Substituting the known values into the equation to find the net force:
$$ F_{net} = (2.2 , \text{kg})(2.3 , \text{m/s}^2) $$
- Solve for the net force
Calculating the above expression:
$$ F_{net} = 5.06 , \text{N} $$
- Set up the equation for forces
The net force is also equal to the difference between the applied force $Q$ and the opposing force $P$:
$$ F_{net} = Q - P $$
- Substitute the known values into the equation
Now substitute the values we have into the equation:
$$ 5.06 , \text{N} = Q - 21.5 , \text{N} $$
- Solve for force Q
Rearranging the equation gives:
$$ Q = 5.06 , \text{N} + 21.5 , \text{N} $$
Calculating this gives:
$$ Q = 26.56 , \text{N} $$
- Round to one decimal place
The final value for force $Q$ rounded to one decimal place is $Q = 26.6 , \text{N}$.
The magnitude of force $Q$ needed is $26.6 , \text{N}$.
More Information
This problem applies Newton's second law, which indicates how the net force acting on an object is related to its mass and acceleration. Understanding the balance of forces is crucial in physics.
Tips
- Confusing the direction of forces. Always ensure you're applying the correct signs (positive for right, negative for left).
- Not accounting for the opposing force when calculating the net force. Remember to consider all forces acting on the object.
AI-generated content may contain errors. Please verify critical information