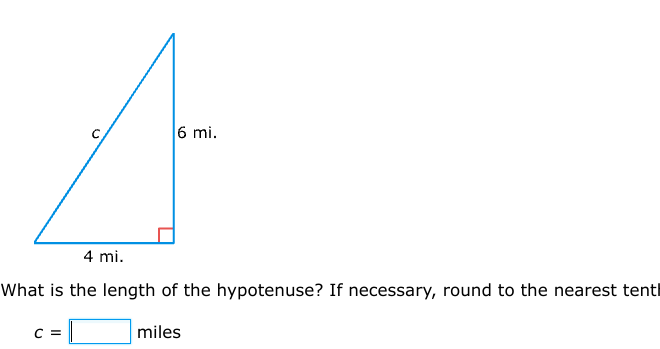
What is the length of the hypotenuse? If necessary, round to the nearest tenth.
Understand the Problem
The question is asking for the length of the hypotenuse of a right triangle, given the lengths of the two other sides (4 miles and 6 miles). We will use the Pythagorean theorem to solve this.
Answer
The length of the hypotenuse is approximately $7.2$ miles.
Answer for screen readers
The length of the hypotenuse is approximately $7.2$ miles.
Steps to Solve
- Apply the Pythagorean Theorem
To find the length of the hypotenuse $c$ in a right triangle, we use the Pythagorean theorem, which states: $$ c^2 = a^2 + b^2 $$ where $a$ and $b$ are the lengths of the other two sides. Here, $a = 4$ miles and $b = 6$ miles.
- Square the lengths of the sides
First, we calculate the square of each side: [ a^2 = 4^2 = 16 ] [ b^2 = 6^2 = 36 ]
- Add the squares together
Now, we add the squared lengths: $$ c^2 = 16 + 36 = 52 $$
- Find the length of the hypotenuse
To solve for $c$, take the square root of both sides: $$ c = \sqrt{52} $$
- Simplify $c$ if necessary
We can simplify $\sqrt{52}$: $$ c = \sqrt{4 \times 13} = 2\sqrt{13} $$
- Calculate the approximate value
To express this as a decimal, we will compute: $$ c \approx 2 \times 3.60555 \approx 7.211 $$
- Round to the nearest tenth
Finally, rounding $c$ to the nearest tenth gives: $$ c \approx 7.2 $$
The length of the hypotenuse is approximately $7.2$ miles.
More Information
The Pythagorean theorem is a fundamental principle in geometry, applicable only to right triangles. It reveals the relationship between the lengths of the sides, allowing for calculations of distances in a variety of fields, including architecture and surveying.
Tips
- Incorrectly squaring the sides: It's essential to square the lengths accurately. Double-check your calculations to avoid simple arithmetic errors.
- Forgetting to take the square root: Always remember to find the square root after adding the squares of the sides to obtain the hypotenuse.
AI-generated content may contain errors. Please verify critical information