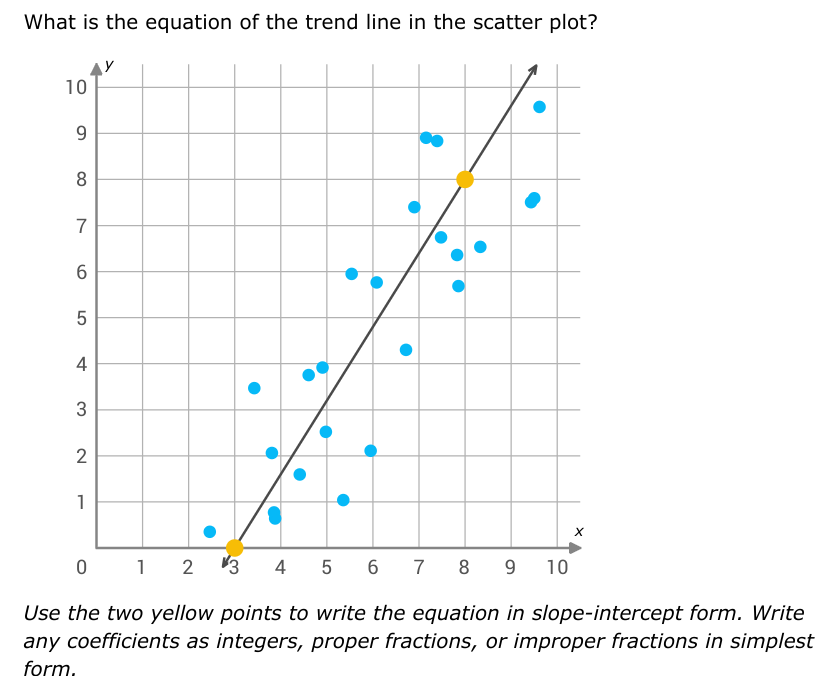
What is the equation of the trend line in the scatter plot? Use the two yellow points to write the equation in slope-intercept form. Write any coefficients as integers, proper frac... What is the equation of the trend line in the scatter plot? Use the two yellow points to write the equation in slope-intercept form. Write any coefficients as integers, proper fractions, or improper fractions in simplest form. Read more

Steps to Solve

1. Identify the yellow points The yellow points on the scatter plot are the key to finding the trend line. Let's denote the two yellow points as ( P_1(2, 5) ) and ( P_2(4, 8) ).

2. Calculate the slope (m) The slope of the line can be found using the formula:

$$ m = \frac{y_2 - y_1}{x_2 - x_1} $$

Substituting in the values from points ( P_1 ) and ( P_2 ):

$$ m = \frac{8 - 5}{4 - 2} = \frac{3}{2} $$

3. Find the y-intercept (b) To find the y-intercept, we can use the slope-intercept form of the equation ( y = mx + b ). We can plug in one of the points and the slope:

Using point ( P_1(2, 5) ):

$$ 5 = \frac{3}{2}(2) + b $$

Calculating this gives:

$$ 5 = 3 + b $$

Solving for ( b ):

$$ b = 5 - 3 = 2 $$

4. Write the equation of the trend line Now we have both the slope and y-intercept. We can write the equation in slope-intercept form:

$$ y = mx + b $$

Substituting ( m ) and ( b ):

$$ y = \frac{3}{2}x + 2 $$