What error did Lennox make in her conclusion about the similarity of triangles ABE and DCE?
Understand the Problem
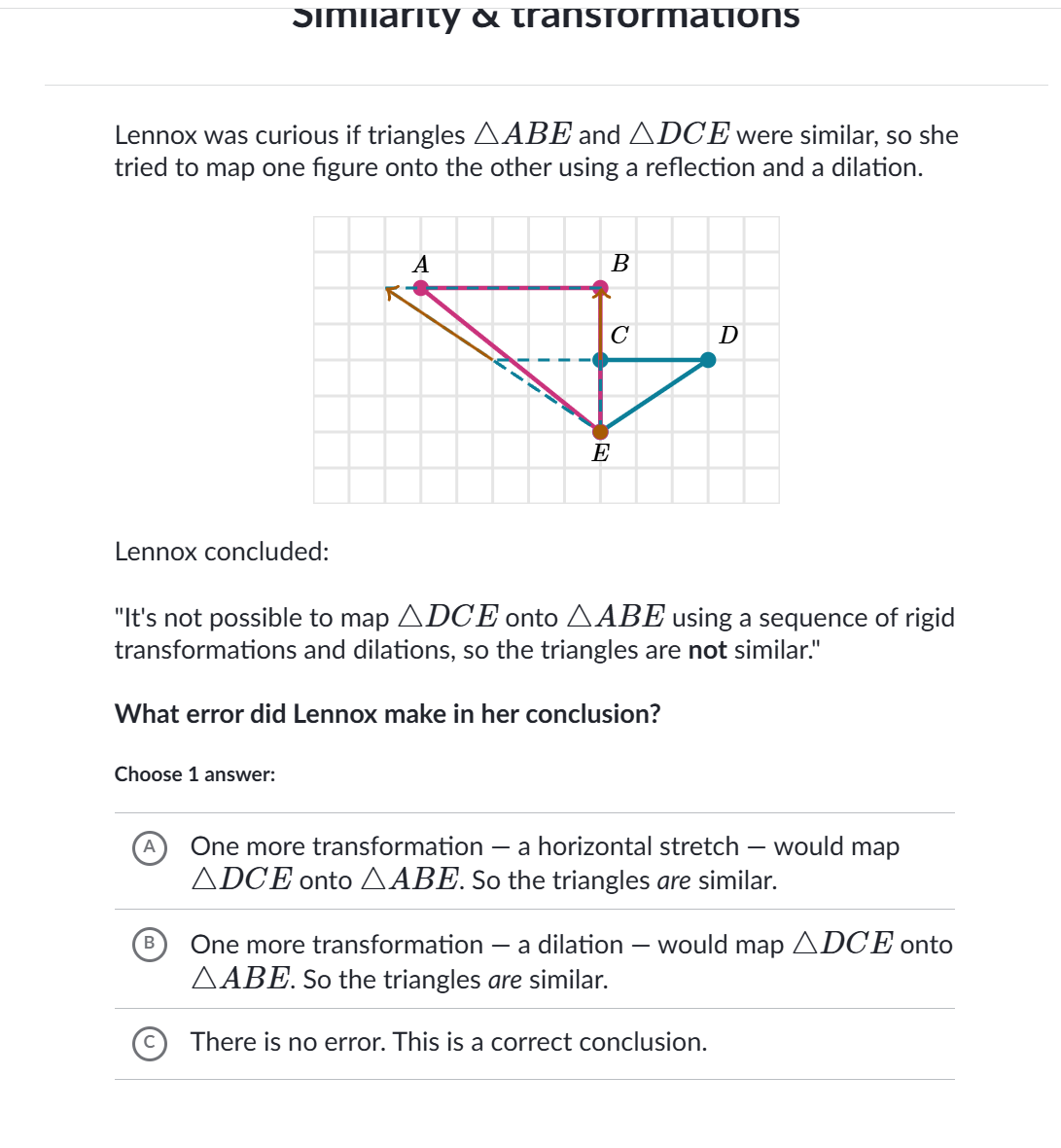
The question is asking about the error made by Lennox in concluding that two triangles are not similar based on the transformations allowed. It is necessary to analyze whether the given transformations can successfully show the similarity of the triangles.
Answer
B: One more transformation — a dilation — would map $ \triangle DCE $ onto $ \triangle ABE $. So the triangles are similar.
Answer for screen readers
The correct answer is B: One more transformation — a dilation — would map $ \triangle DCE $ onto $ \triangle ABE $. So the triangles are similar.
Steps to Solve
-
Identify the Transformations Lennox stated that the triangles cannot be mapped onto each other using rigid transformations and dilations. Rigid transformations include rotations, reflections, and translations, while dilations involve resizing the triangles.
-
Analyze the Triangles Check if triangles $ \triangle DCE $ and $ \triangle ABE $ can be similar. Generally, two triangles are similar if their angles are equal and the lengths of their sides are proportional.
-
Consider Additional Transformations Since Lennox concluded that a rigid transformation and dilation weren’t enough, we need to evaluate whether adding additional transformations could yield a mapping from $ \triangle DCE $ to $ \triangle ABE $.
-
Evaluate the Options Examine the provided options:
- Option A suggests a horizontal stretch could map the triangles, which implies they may be similar.
- Option B asserts that a dilation can map the two triangles onto each other, indicating similarity.
- Option C states that there is no error in her conclusion.
-
Determine the Correctness To determine Lennox’s error, we analyze whether any of the options can prove the similarity of the triangles based on transformations. If at least one option correctly identifies a transformation that validates similarity, Lennox's conclusion is flawed.
The correct answer is B: One more transformation — a dilation — would map $ \triangle DCE $ onto $ \triangle ABE $. So the triangles are similar.
More Information
Lennox's conclusion disregarded the potential for additional dilations to confirm the similarity between the triangles. In geometry, triangles can be similar even if they are not congruent, as long as their corresponding angles are equal and their sides are in proportion.
Tips
- Misunderstanding transformations: Students often confuse rigid transformations with similarity, overlooking the role of dilations.
- Assuming that two triangles must be congruent to be similar: Similar triangles can differ in size provided their angles are equal.
AI-generated content may contain errors. Please verify critical information