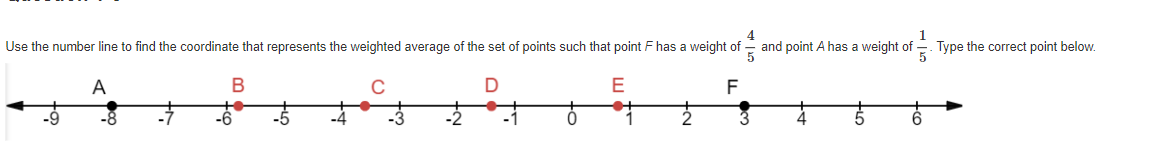
Use the number line to find the coordinate that represents the weighted average of the set of points such that point F has a weight of 4/5 and point A has a weight of 1/5. Type the... Use the number line to find the coordinate that represents the weighted average of the set of points such that point F has a weight of 4/5 and point A has a weight of 1/5. Type the correct point below. Read more

Steps to Solve

1. Identify coordinates and weights
   Point ( A ) is at -9 and point ( F ) is at 3. The weights given are:

   • Weight of ( F = \frac{4}{5} )
   • Weight of ( A = \frac{1}{5} )

2. Use the weighted average formula
   The weighted average ( \overline{x} ) can be calculated using the formula:
   $$ \overline{x} = \frac{(A \cdot w_A) + (F \cdot w_F)}{w_A + w_F} $$
   where ( A ) is the value at point A, ( w_A ) is its weight, ( F ) is the value at point F, and ( w_F ) is its weight.

3. Plug in the values
   Substituting the known values into the formula:
   $$ \overline{x} = \frac{(-9 \cdot \frac{1}{5}) + (3 \cdot \frac{4}{5})}{\frac{1}{5} + \frac{4}{5}} $$

4. Calculate the numerator
   The numerator becomes:
   $$ (-9 \cdot \frac{1}{5}) + (3 \cdot \frac{4}{5}) = -\frac{9}{5} + \frac{12}{5} = \frac{3}{5} $$

5. Calculate the denominator
   The denominator simplifies to:
   $$ \frac{1}{5} + \frac{4}{5} = \frac{5}{5} = 1 $$

6. Find the weighted average
   Therefore, the weighted average is:
   $$ \overline{x} = \frac{\frac{3}{5}}{1} = \frac{3}{5} $$

7. Convert to a numerical coordinate
   We need to convert ( \frac{3}{5} ) to a numerical value on the number line.
   This is equivalent to 0.6, which lies between 0 and 1.