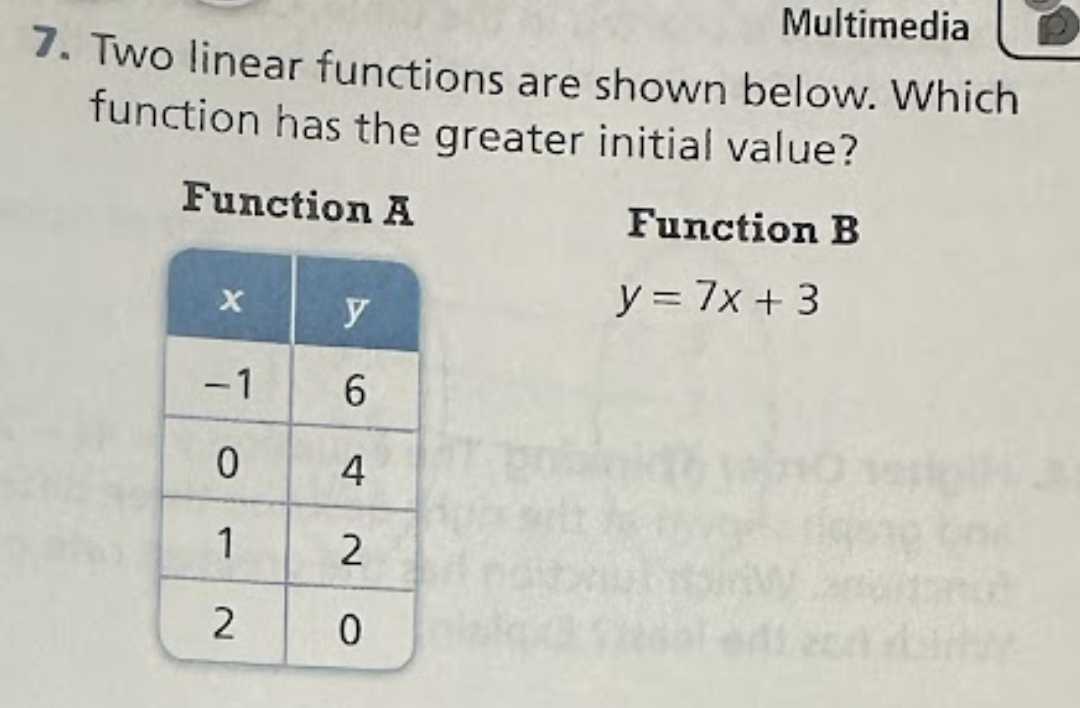
Two linear functions are shown below. Which function has the greater initial value?
Understand the Problem
The question is asking to compare the initial values of two linear functions, one represented by a table (Function A) and the other by a linear equation (Function B). The initial value refers to the y-intercept of the functions.
Answer
Function A has the greater initial value, which is 4.
Answer for screen readers
The function that has the greater initial value is Function A, with an initial value of 4.
Steps to Solve
- Identify Function A's Initial Value
To find the initial value of Function A, we look for the value of $y$ when $x = 0$. From the table for Function A, when $x = 0$, $y = 4$. Thus, the initial value of Function A is 4.
- Identify Function B's Initial Value
For Function B, the equation is given as $y = 7x + 3$. The initial value is found by evaluating the function at $x = 0$:
$$ y = 7(0) + 3 = 3 $$
So, the initial value of Function B is 3.
- Compare the Initial Values
Now that we have the initial values:
- Function A: 4
- Function B: 3
Function A has a greater initial value than Function B.
The function that has the greater initial value is Function A, with an initial value of 4.
More Information
Initial values (or y-intercepts) show the starting point of linear functions on a graph. Function A's table helps determine the initial value quickly, while Function B's equation demonstrates how to find it using algebra.
Tips
- Forgetting to use $x = 0$ to find initial values.
- Confusing the y-intercept with other points on the graph.
- Misreading values from the table.
AI-generated content may contain errors. Please verify critical information