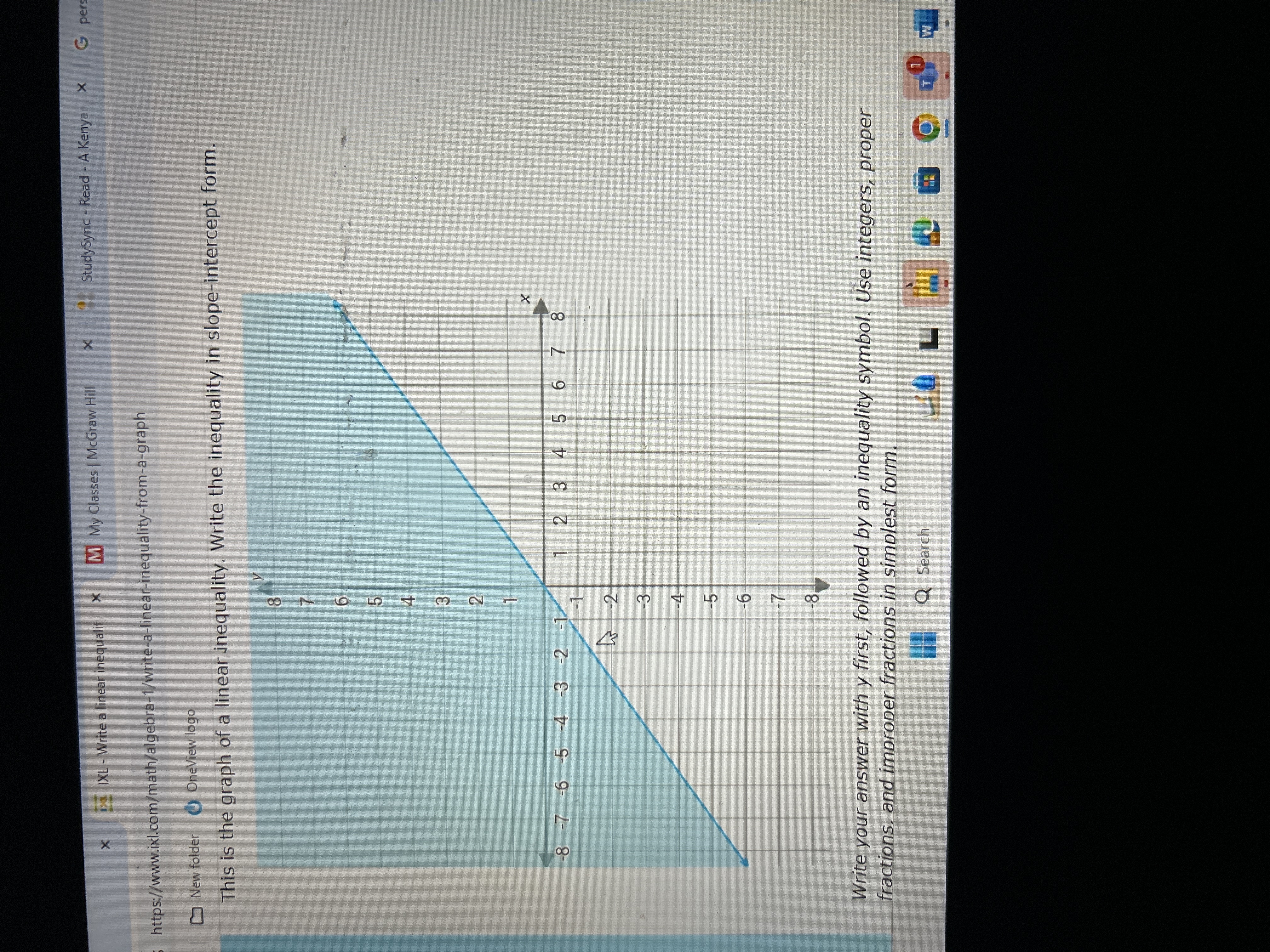
This is the graph of a linear inequality. Write the inequality in slope-intercept form.
Understand the Problem
The question is asking to derive the linear inequality from the provided graph, specifically in slope-intercept form (y = mx + b) and to include an inequality symbol based on the graph's shading.
Answer
The final inequality is: $$ y < \frac{3}{4}x + 2 $$
Answer for screen readers
The inequality in slope-intercept form is: $$ y < \frac{3}{4}x + 2 $$
Steps to Solve
- Identify the slope and y-intercept
From the graph, identify two clear points on the line. For instance, we can use the points (2, 4) and (6, 7).
The slope ( m ) is calculated as follows: $$ m = \frac{y_2 - y_1}{x_2 - x_1} = \frac{7 - 4}{6 - 2} = \frac{3}{4} $$
The y-intercept ( b ) can be observed as the point where the line intersects the y-axis, which is at (0, 2). Hence, ( b = 2 ).
- Write the slope-intercept form
The slope-intercept form of the equation is given by: $$ y = mx + b $$ Substituting in the values from our findings:
$$ y = \frac{3}{4}x + 2 $$
- Determine the inequality symbol
Since the line is dashed (not solid), it indicates that the inequality does not include the line itself. Therefore, we use '<' instead of '≤'.
The shaded region is above the line, indicating that ( y ) is greater than values on the line.
Thus, the inequality is: $$ y < \frac{3}{4}x + 2 $$
The inequality in slope-intercept form is: $$ y < \frac{3}{4}x + 2 $$
More Information
This inequality indicates that for any point (x, y) in the shaded area above the dashed line, the value of y will always be less than the corresponding value given by the linear equation.
Tips
- Not identifying whether the line is solid or dashed, which affects the choice of inequality symbol.
- Incorrectly calculating the slope or intercept from the graph.
AI-generated content may contain errors. Please verify critical information