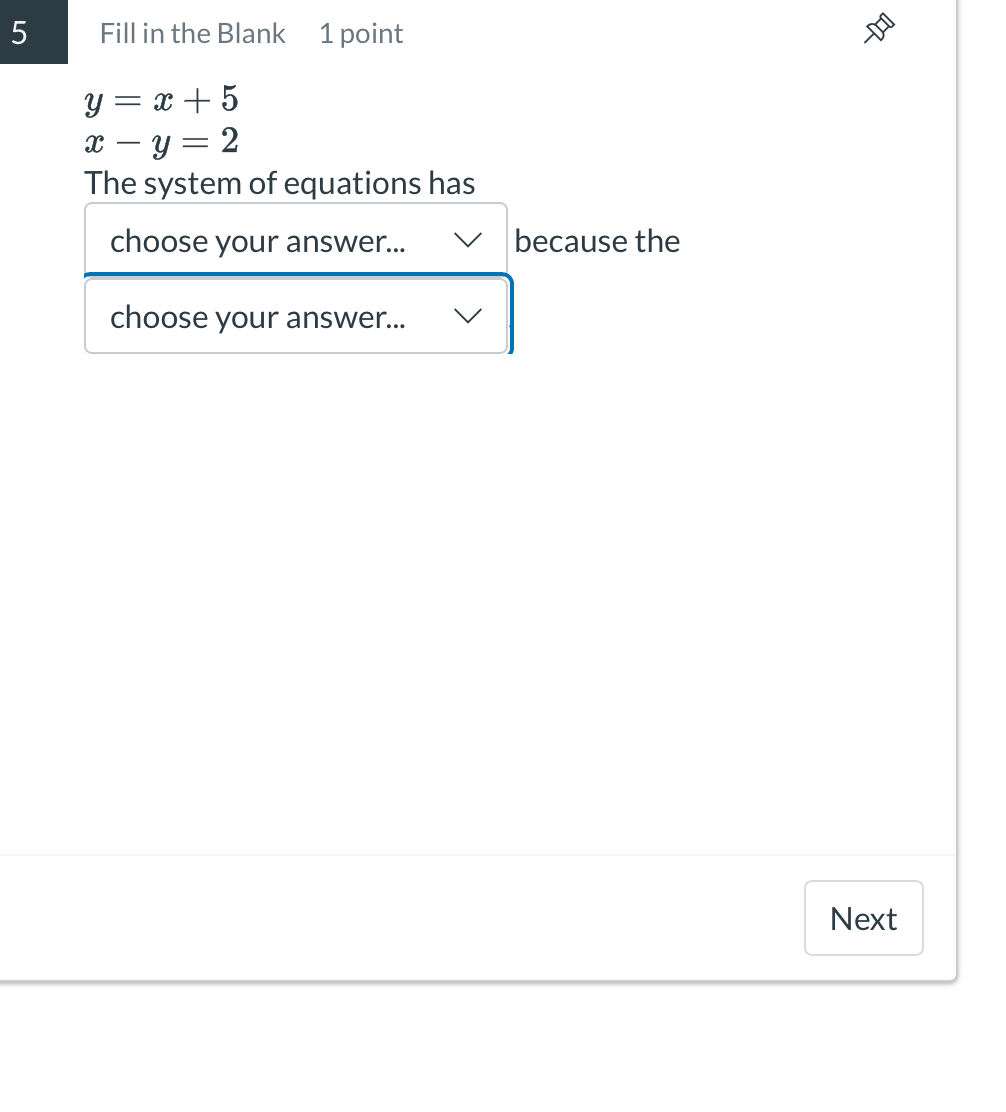
The system of equations has __ because the __.
Understand the Problem
The question is asking to analyze a system of equations and fill in the blanks regarding its properties and the reason behind that classification.
Answer
The system of equations has **no solution** because the **lines are parallel**.
Answer for screen readers
The system of equations has no solution because the lines are parallel.
Steps to Solve
-
Identify the equations The system consists of the equations:
- ( y = x + 5 ) (Equation 1)
- ( x - y = 2 ) (Equation 2)
-
Rearrange Equation 2 We can first rearrange Equation 2 to isolate ( y ): [ x - y = 2 ] Adding ( y ) to both sides gives: [ x = y + 2 ] Rearranging this, we get: [ y = x - 2 ]
-
Set the two equations equal Now we have two expressions for ( y ):
- From Equation 1: ( y = x + 5 )
- From the rearranged Equation 2: ( y = x - 2 )
Set them equal to each other: [ x + 5 = x - 2 ]
-
Simplify the equation Subtract ( x ) from both sides: [ 5 = -2 ] This is a contradiction and indicates that the two equations do not intersect.
-
Conclusion about the system Since the equations lead to a contradiction, the system has no solution. Thus, the system is classified as inconsistent.
The system of equations has no solution because the lines are parallel.
More Information
Inconsistent systems occur when two linear equations represent parallel lines. Since parallel lines never intersect, they have no points in common, leading to no solutions.
Tips
- Misinterpreting parallel lines as having solutions. Remember, parallel lines never cross and therefore have no intersection points.
- Confusing inconsistent systems with systems that have infinitely many solutions, which occur when lines overlap perfectly.
AI-generated content may contain errors. Please verify critical information